Delta RMC151 - Manual (Page 561)

6 Communication
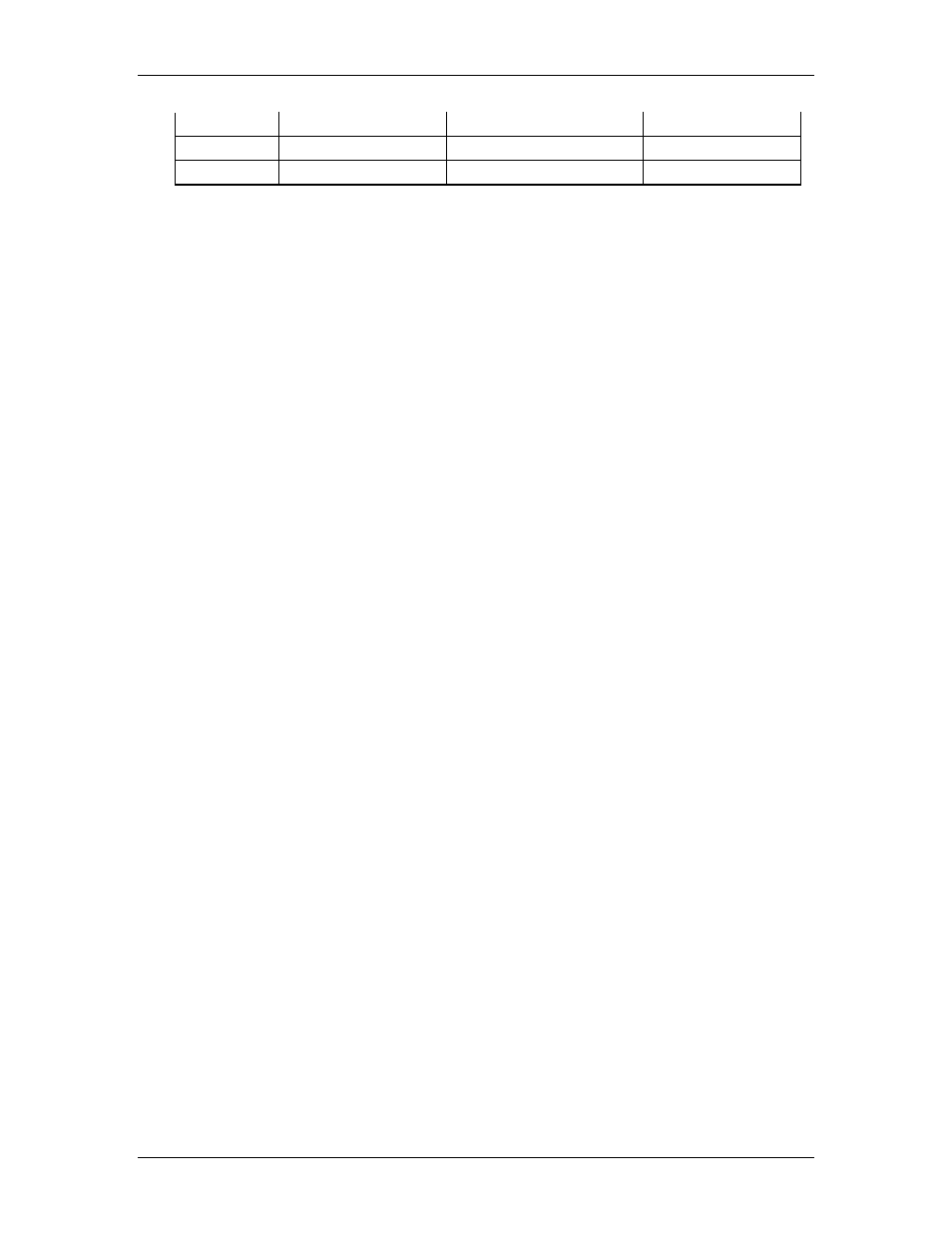
9,600 4000 Not Required 4000
4,800 4000 Not Required 4000
2,400 4000 Not Required 4000
Cable Length Derivation
The values presented in the chart above are based on 24AWG cable with capacitance of
16 pF/ft and the following reasoning. Signals travel through a cable at approximately
66% of c or 0.66 ft/ns. It is assumed that a signal transition will dampen out after three
round trips in the cable. This damping must occur before the bit is sampled or within half
a bit time. One bit time is equal to the reciprocal of the baud rate.
Example:
Compute the cable length for 115,200 baud RS422.
First, we compute a half bit time at this baud rate.
Half Bit Time = 0.5 * 1 / 115200
= 4,340 ns
Next, we convert this time to the distance the signal would travel in this time,
assuming a speed of 0.66 ft/ns as described above:
Distance = 4,340 ns * 0.66 ft / ns
= 2890 ft
Since three round trips are required for the signal transition to dampen and each
round trip is twice the length of the cable, the total distance in feet is divided by six
to get the final unterminated cable length:
Length = 2890 ft / 6
= 482 ft
This value is then rounded down to allow for inexact cable velocities and damping
rates, giving us 475 ft.
The Biasing Concept
RS-485 indicates a binary 1 when the A line is at least 200 mV negative with respect to B,
and a binary 0 when A is at least 200 mV positive with respect to B. It is important that
the lines always be in a known state, not only when being driven. Biasing forces the
network into a known state when the lines are idle and therefore otherwise not driven.
A known state is forced by allowing current to flow across the termination resistor.
Therefore, biasing is usually selected on the RMC that also has a termination. However,
some masters only have termination, in which case the user may want to only select
biasing on an RMC close to the master. The current will then flow across the master's
termination resistor.
The RMC7xS requires biasing in order to be in a known state when the lines are idle. The
biasing forces a binary 1.
Example:
This example assumes that there is a single master and two RMCs on the network.
Compute the voltage across a 120Ω termination resistor when using 1150Ω biasing
resistors.
deltamotion.com 541