Metrohm viva 1.0 Manual - Manual (Page 604)

5.6 Evaluation subwindow
￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭￭
592 ￭￭￭￭￭￭￭￭
viva 1.0
Name Symbol Unit
Electrical potential U V = WA -1
5.6.7.2 Result calculation from calibration curves and error calculation
For all three possible calibration methods, the concentration (e.g. a mass
concentration, a volume concentration or a molar concentration) must be
determined in the measuring vessel with the help of a calibration curve.
This calibration curve itself must be determined by the measurement of
solutions for which the concentrations are known. The parameters for a
specified linear or non-linear curve function are then calculated from the
value pairs Evaluation quantity / concentration. Because of the fact
that, as a general principle, the calculations of the calibration curves and
of the results based upon them, in addition to the associated error calcula-
tion, remain the same for all calibration methods, the calculation proce-
dure that is applied shall be described in general terms in the following.
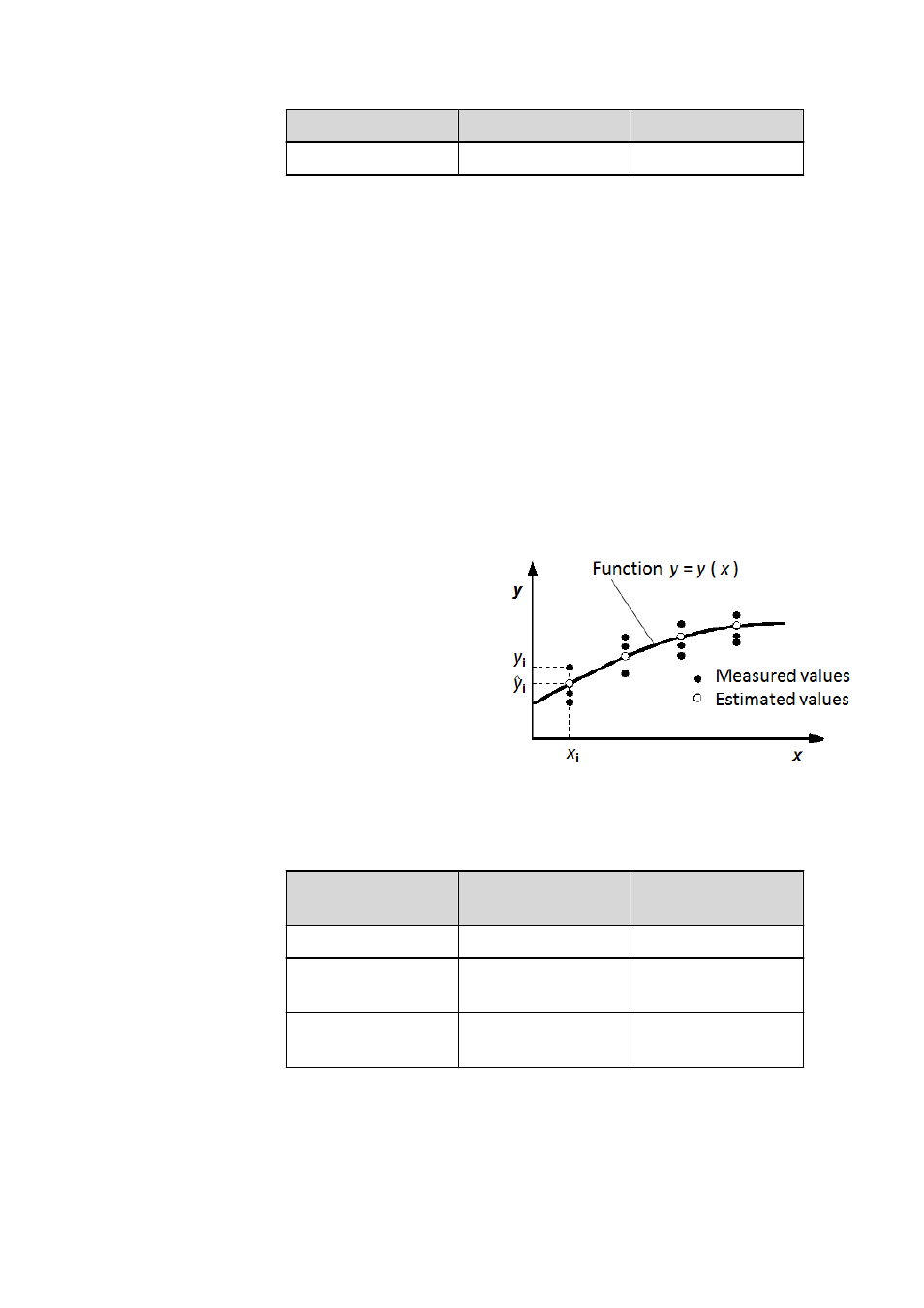
The connection between the variables x and y are sought on the basis of
the measured values x
i
, y
i
, for which it is assumed that the following pre-
requisites apply:
￭ The variable x is error-
free.
￭ The variable y is
dependent on x and
can be described by the
function y = y(x).
￭ The error with the mea-
surement of y is distrib-
uted normally and is
sufficiently small to be
able to apply linear
error calculation.
Depending on the calibration method selected, the following model func-
tions are available for the calculation of the calibration curve y = y(x):
Selected curve type Calibration func-
tion
Description
Linear regression y = a + bx Line
Quadratic regres-
sion y = a + bx + cx 2 Non-linear curve of
the 2nd degree
Nonlinear regres-
sion y = a + bx + dx 4 Non-linear curve of
the 4th degree