HP 48g Graphing Calculator - Manual (Page 354)

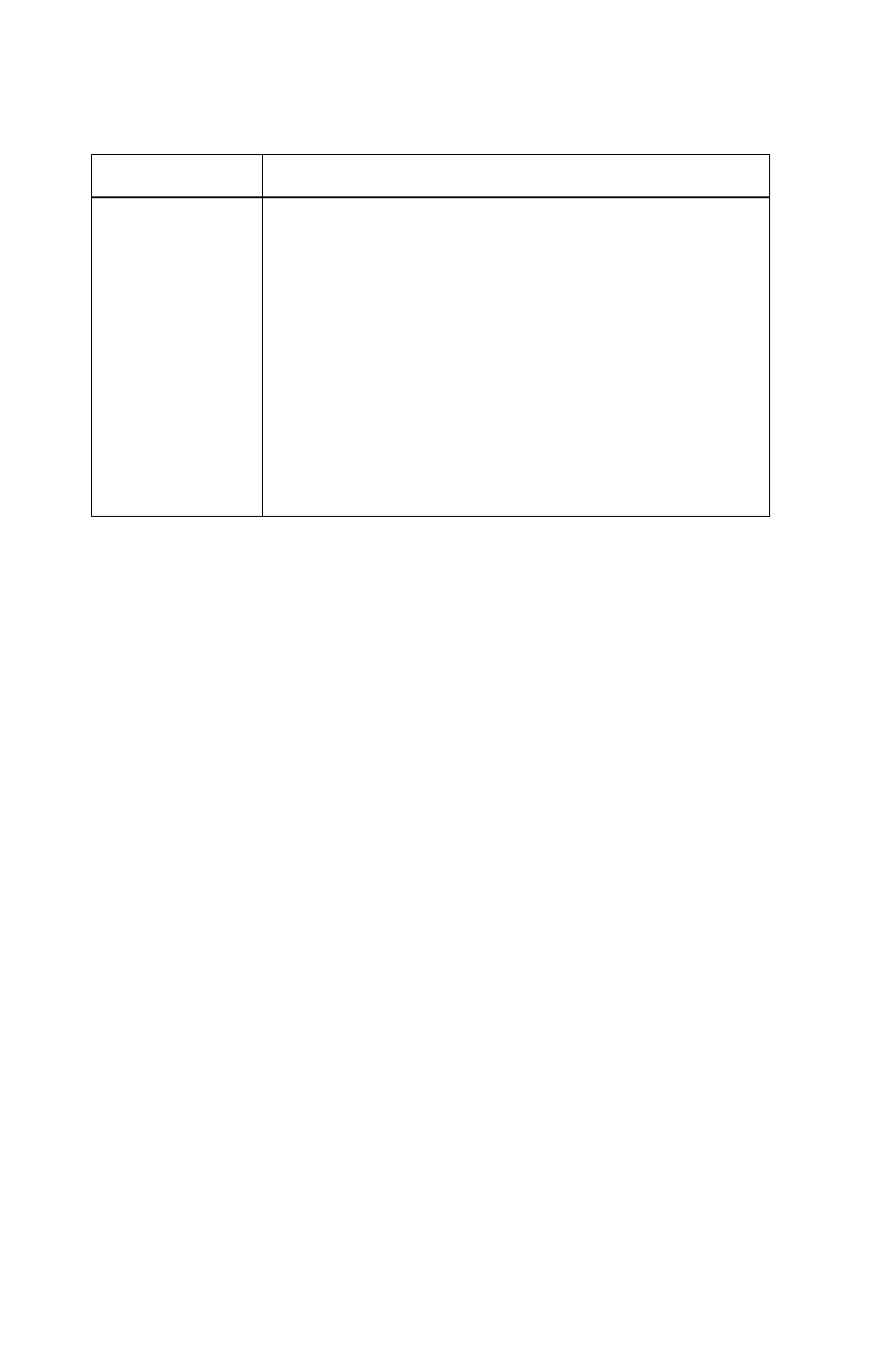
Meanings of Menu Labels
label Meaning
[IIBoIlj
Value xO not defined by you and not used in the
last solution—it can change in the next solution.
1............. 1
Value xO not defined by you, but found in the last
solution—it can change in the next solution.
0 Value xO defined by you, but not used in the last
solution—it can’t change in the next solution
(unless you solve for only this variable).
L' Value xO defined by you and used in the last
solution—it can’t change in the next solution
(unless you solve for only this variable).
25
Defining a Set of Equations
When you design a set of equations, you should do it with an
understanding of how the Multiple-Equation Solver uses the equations
to solve problems.
The Multiple-Equation Solver uses the same process you’d use to solve
for an unknown variable—assuming you weren’t allowed to create
additional equations. You’d look through the set of equations for one
that has only one variable that you don’t know. You’d use the HP 48
root-finder to find its value. Then you’d do this again until you’ve
found the variable you want.
You should choose your equations to allow likely unknown variables to
occur individually in equations. You must avoid having two or more
unknown variables in all equations. You can also specify the equations
in an order that’s best for your problems.
For example, the following three equations define initial velocity and
acceleration based on two observed distances and times. The first two
equations alone are mathematically sufficient for solving the problem,
but each equation contains two unknown variables. Adding the third
equation allows a successful solution because it contains only one of
the unknown variables.
25-8 The Equation Library