Agilent Technologies N9010A - Manual (Page 170)

170 Chapter 17
Noise Figure Measurement Application
General Specifications
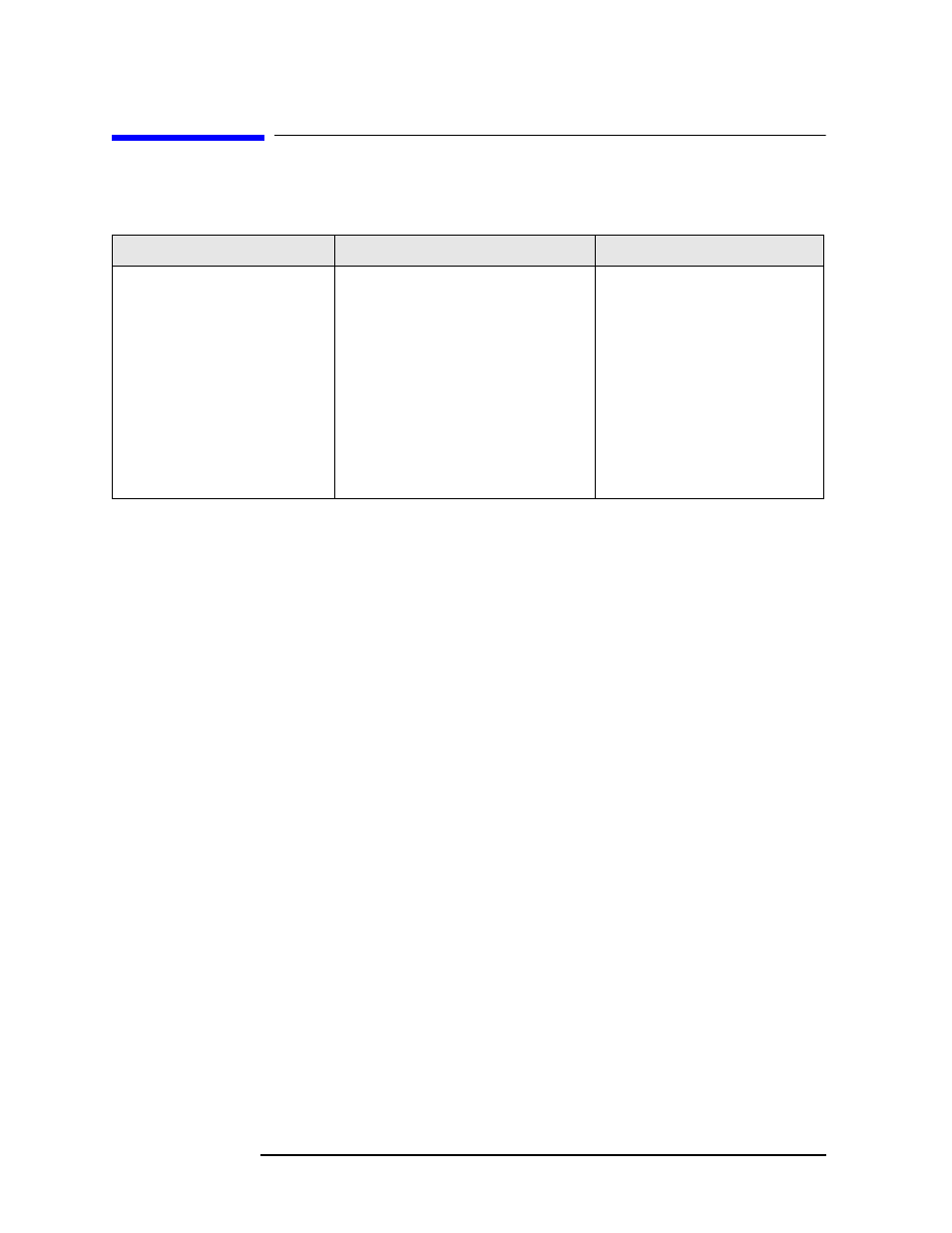
General Specifications
Description Specifications Supplemental Information
Noise Figure Uncertainty Calculator
a
a. The figures given in the table are for the uncertainty added by the EXASignal Analyzer instrument
only. To compute the total uncertainty for your noise figure measurement, you need to take into
account other factors including: DUT NF, Gain and Match, Instrument NF, Gain Uncertainty and
Match; Noise source ENR uncertainty and Match. The computations can be performed with the uncer-
tainty calculator included with the Noise Figure Measurement Personality. Go to
Mode Setup then
select Uncertainty Calculator . Similar calculators are also available on the Agilent web site; go to
http://www.agilent.com/find/nfu .
<200 kHz
See note
b
b. Instrument Uncertainty is nominally the same in this frequency range as in the higher frequency range.
However, total uncertainty is higher because the analyzer has poorer noise figure, leading to higher
uncertainties as computed by the uncertainty calculator. Also, there is a paucity of available noise
sources in this range.
10 MHz to internal
preamplifier’s frequency limit
c
c. At the highest frequencies, especially above 40 GHz, the only Agilent Supra-26-GHz noise source, the
346CK01, often will not have enough ENR to allow for the calibration operation. For high gain DUTs,
operation without calibration can still be highly accurate. The Noisecom NC5200 series does have ade-
quate ENR for calibration.
Using internal preamp
(such as Option P07) and
RBW = 4 MHz
Noise Source ENR
Measurement
Range Instrument
Uncertainty
de
d. “Instrument Uncertainty” is defined for noise figure analysis as uncertainty due to relative amplitude
uncertainties encountered in the analyzer when making the measurements required for a noise figure
computation. The relative amplitude uncertainty depends on, but is not identical to, the relative display
scale fidelity, also known as incremental log fidelity. The uncertainty of the analyzer is multiplied
within the computation by an amount that depends on the Y factor to give the total uncertainty of the
noise figure or gain measurement.
See Agilent App Note 57-2, literature number 5952-3706E for details on the use of this specification.
Jitter (amplitude variations) will also affect the accuracy of results. The standard deviation of the mea-
sured result decreases by a factor of the square root of the Resolution Bandwidth used and by the
square root of the number of averages. This application uses the 4 MHz Resolution Bandwidth as
default because this is the widest bandwidth with uncompromised accuracy.
e. The instrument uncertainties shown are under best-case sweep time conditions, which is a sweep time
near to the period of the power line, such as 20 ms for 50 Hz power sources. The behavior can be
greatly degraded (uncertainty increased nominally by 0.12 dB) by setting the sweep time per point far
from an integer multiple of the period of the line frequency.
4 to 6.5 dB 0 to 20 dB
±0.02 dB
12 to 17 dB 0 to 30 dB
±0.025 dB
20 to 22 dB 0 to 35 dB
±0.03 dB