Casio ClassPad II fx-CP400 User Manual
Page 137
Chapter 7: Statistics Application
137
When
n
is an odd number, using the median of the total population as the reference, the population
elements are divided into two groups: values less than the median and values greater than the median.
The median value is excluded. Q
1
and Q
3
then become the values described below.
Q
1
= {median of the group of (
n
− 1)/2 items from the bottom of the population}
Q
3
= {median of the group of (
n
− 1)/2 items from the top of the population}
When
n
= 1, Q
1
= Q
3
= population center point.
[Q
1
, Q
3
on Data] checked: The Q
1
and Q
3
values for this calculation method are described below.
Q
1
= {value of element whose cumulative frequency ratio is greater than 1/4 and nearest to 1/4}
Q
3
= {value of element whose cumulative frequency ratio is greater than 3/4 and nearest to 3/4}
The following shows an actual example of the above. (Number of Elements: 10)
Data Value
Frequency
Cumulative Frequency
Cumulative Frequency Ratio
1
1
1
1/10 = 0.1
2
1
2
2/10 = 0.2
3
2
4
4/10 = 0.4
4
3
7
7/10 = 0.7
5
1
8
8/10 = 0.8
6
1
9
9/10 = 0.9
7
1
10
10/10 = 1.0
3 is the value whose cumulative frequency
ratio is greater than 1/4 and nearest to 1/4,
so Q
1
= 3.
5 is the value whose cumulative frequency
ratio is greater than 3/4 and nearest to 3/4,
so Q
3
= 5.
Reference Point (0.25)
Reference Point (0.75)
Q
1
0.1
0.2
0.4
0.7
0.8
0.9
1.0
Q
3
1
2
6
3
3
4
4
4
7
5
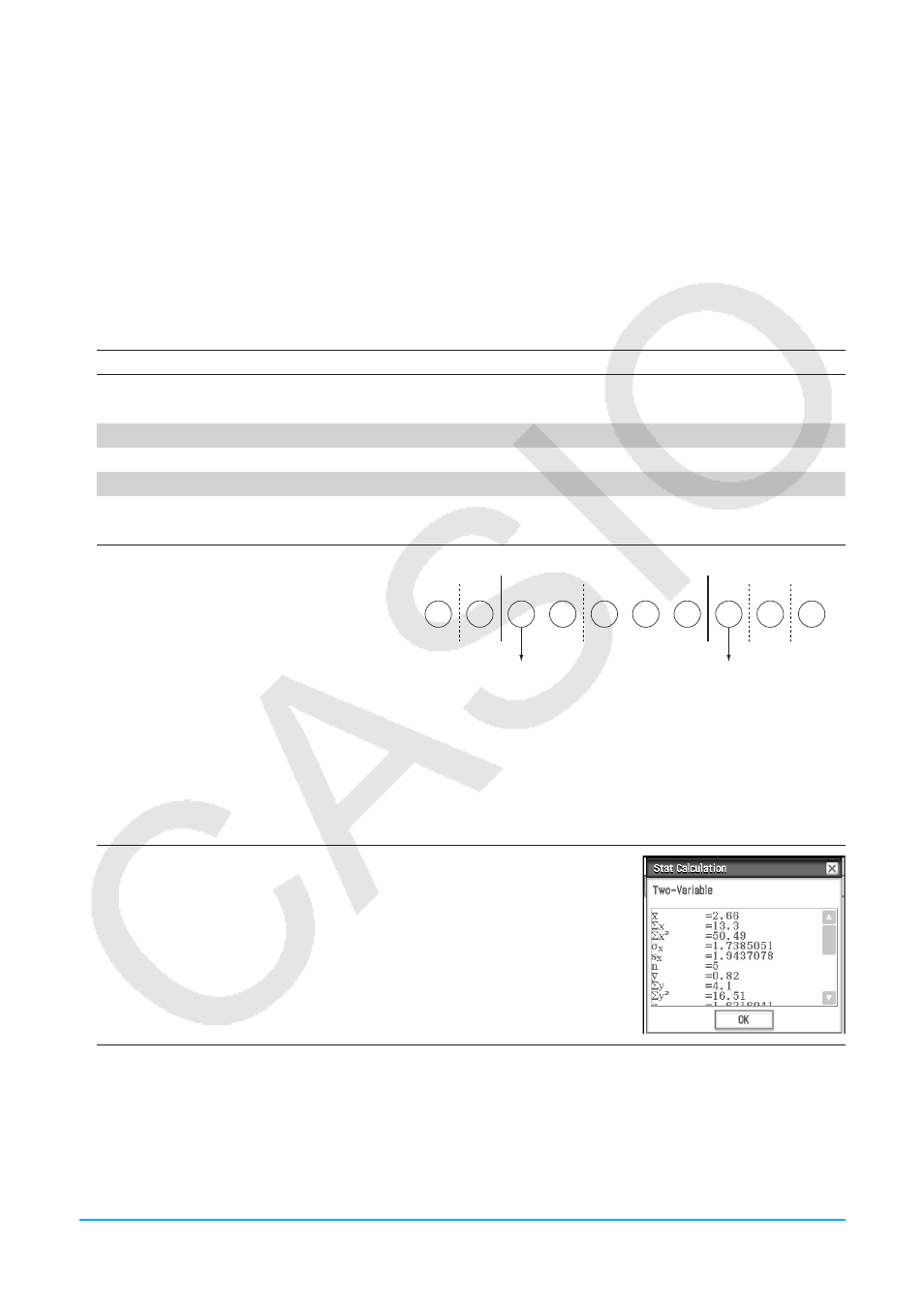
u To display paired-variable calculation results
1. On the Stat Editor window or Stat Graph window, tap [Calc] - [Two-Variable].
2. On the dialog box that appears, specify the [XList] and [YList] names, select the [Freq] setting, and then tap
[OK].
• This displays the dialog box with the paired-variable statistical calculation results described below.
o, p :
sample mean
Σ
x
,
Σ
y
: sum of data
Σ
x
2
,
Σ
y
2
: sum of squares
σ
x
,
σ
y
:
population standard
deviation
s
x
, s
y
:
sample standard deviation
n
:
sample size
Σ
xy
:
sum of products of
XList and YList data
minX, minY : minimum
maxX, maxY : maximum
