HP 50g Graphing Calculator User Manual
Page 593
Page 18-26
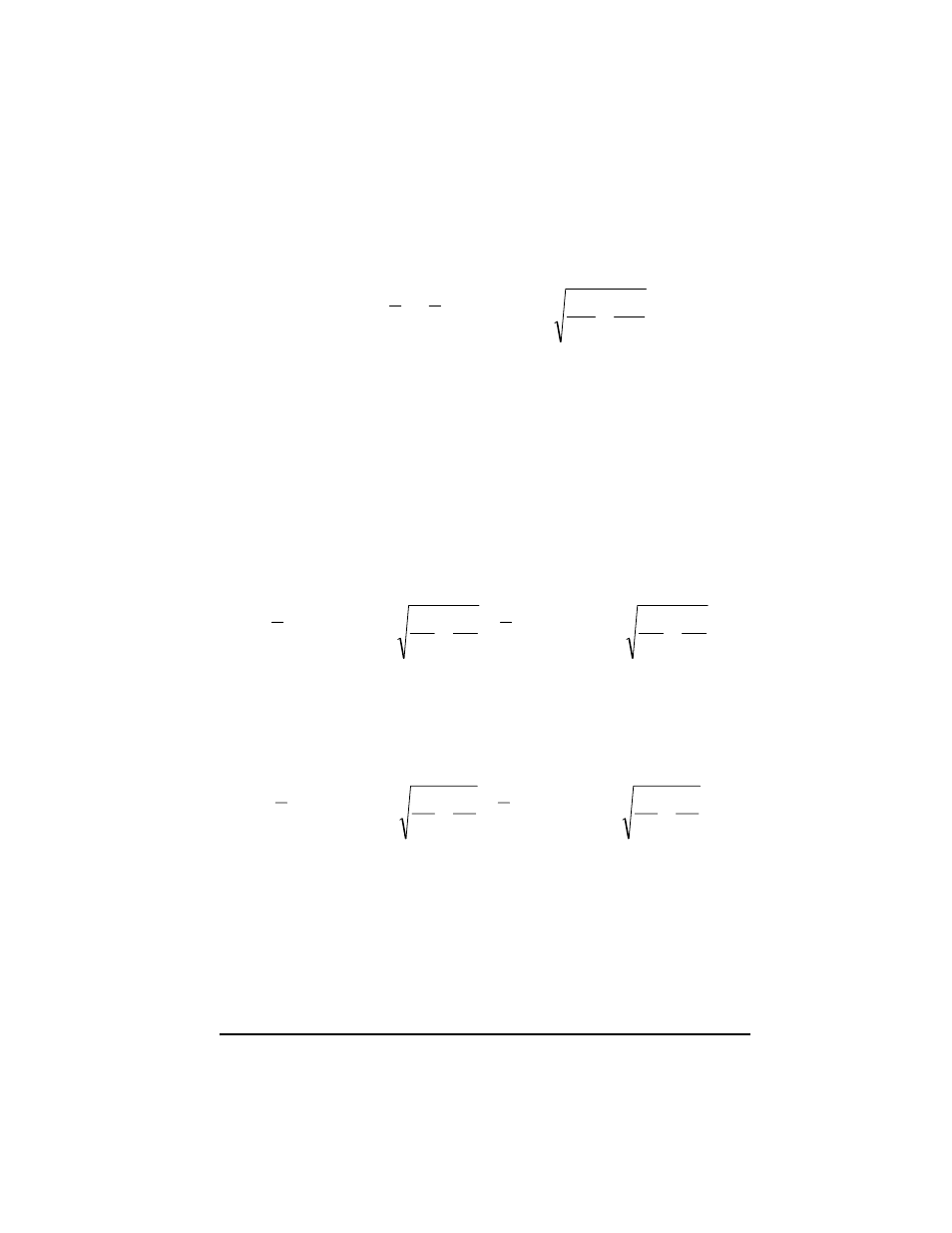
Estimators for the mean and standard deviation of the difference and sum of the
statistics S
1
and S
2
are given by:
In these expressions,
⎯X
1
and
⎯X
2
are the values of the statistics S
1
and S
2
from
samples taken from the two populations, and
σ
S1
2
and
σ
S2
2
are the variances
of the populations of the statistics S
1
and S
2
from which the samples were
taken.
Confidence intervals for sums and differences of mean values
If the population variances
σ
1
2
and
σ
2
2
are known, the confidence intervals for
the difference and sum of the mean values of the populations, i.e.,
μ
1
±μ
2
, are
given by:
For large samples, i.e., n
1
> 30 and n
2
> 30, and unknown, but equal,
population variances
σ
1
2
=
σ
2
2
, the confidence intervals for the difference and
sum of the mean values of the populations, i.e.,
μ
1
±μ
2
, are given by:
If one of the samples is small, i.e., n
1
< 30 or n
2
< 30, and with unknown, but
equal, population variances
σ
1
2
=
σ
2
2
, we can obtain a “pooled” estimate of
the variance of
μ
1
±μ
2
, as s
p
2
= [(n
1
-1)
⋅s
1
2
+(n
2
-1)
⋅s
2
2
]/( n
1
+n
2
-2).
2
2
2
1
2
1
2
1
2
1
2
1
ˆ
,
ˆ
n
n
X
X
S
S
S
S
S
S
σ
σ
σ
μ
+
=
±
=
±
±
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
+
⋅
+
±
+
⋅
−
±
2
2
2
1
2
1
2
/
2
1
2
2
2
1
2
1
2
/
2
1
)
(
,
)
(
n
n
z
X
X
n
n
z
X
X
σ
σ
σ
σ
α
α
.
)
(
,
)
(
2
2
2
1
2
1
2
/
2
1
2
2
2
1
2
1
2
/
2
1
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
+
⋅
+
±
+
⋅
−
±
n
S
n
S
z
X
X
n
S
n
S
z
X
X
α
α
