Solution with the inverse matrix, Solution by “division” of matrices – HP 50g Graphing Calculator User Manual
Page 354
Page 11-27
Compare these three solutions with the ones calculated with the numerical
solver.
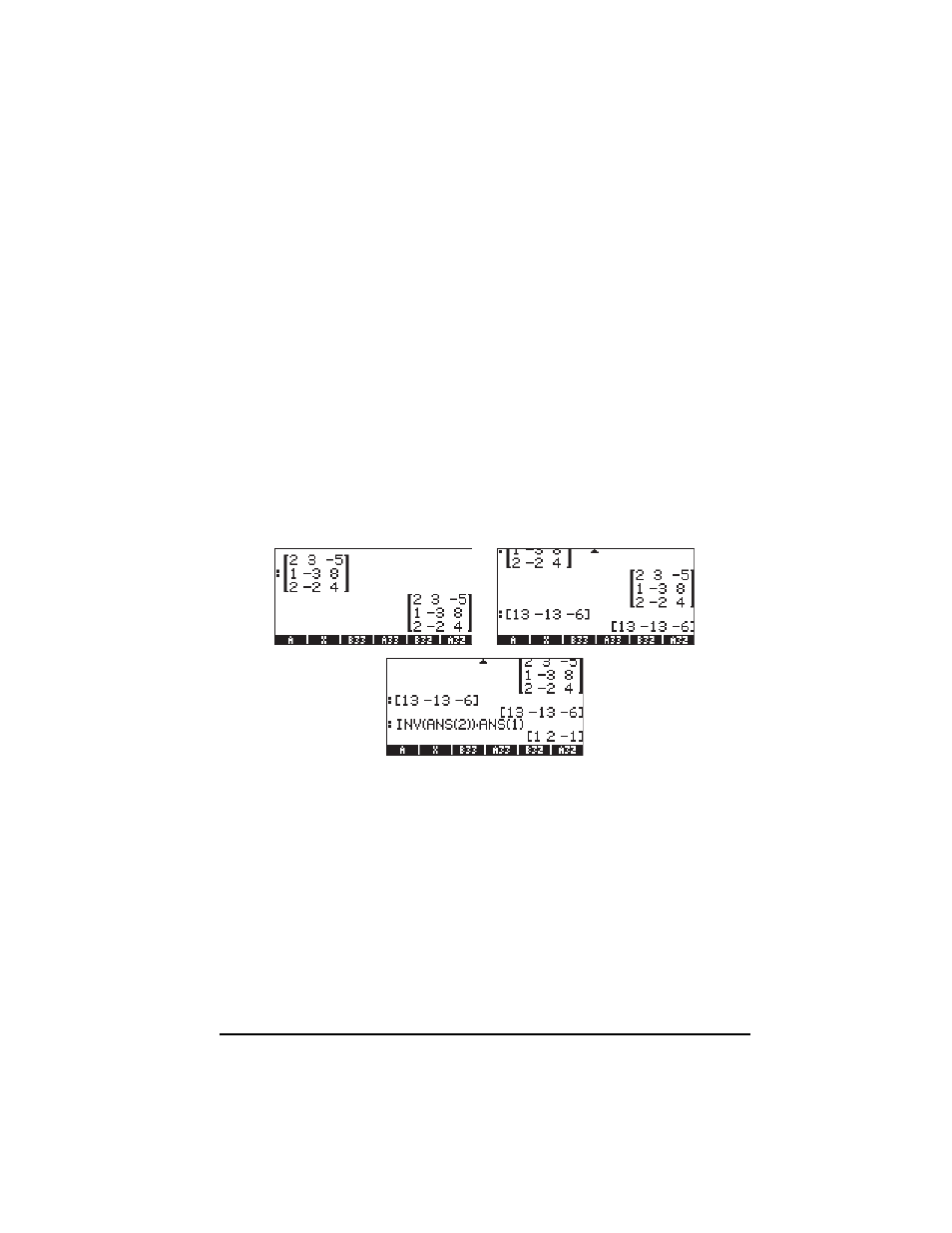
Solution with the inverse matrix
The solution to the system A
⋅x = b, where A is a square matrix is x = A
-1
⋅ b.
This results from multiplying the first equation by A
-1
, i.e., A
-1
⋅A⋅x = A
-1
⋅b. By
definition, A
-1
⋅A = I, thus we write I⋅x = A
-1
⋅b. Also, I⋅x = x, thus, we have, x
= A
-1
⋅ b.
For the example used earlier, namely,
2x
1
+ 3x
2
–5x
3
= 13,
x
1
– 3x
2
+ 8x
3
= -13,
2x
1
– 2x
2
+ 4x
3
= -6,
we can find the solution in the calculator as follows:
which is the same result found earlier.
Solution by “division” of matrices
While the operation of division is not defined for matrices, we can use the
calculator’s / key to “divide” vector b by matrix A to solve for x in the matrix
equation A
⋅x = b. This is an arbitrary extension of the algebraic division
operation to matrices, i.e., from A
⋅x = b, we dare to write x = b/A
(Mathematicians would cringe if they see this!) This, of course is interpreted as
(1/A)
⋅b = A
-1
⋅b, which is the same as using the inverse of A as in the previous
section.
