HP 50g Graphing Calculator - Manual (Page 361)

Page 11-34
Multiply row 3 by –1/7: 7\Y 3 @RCI!
Multiply row 3 by –1, add it to row 2, replacing it: 1\ # 3
#2 @RCIJ!
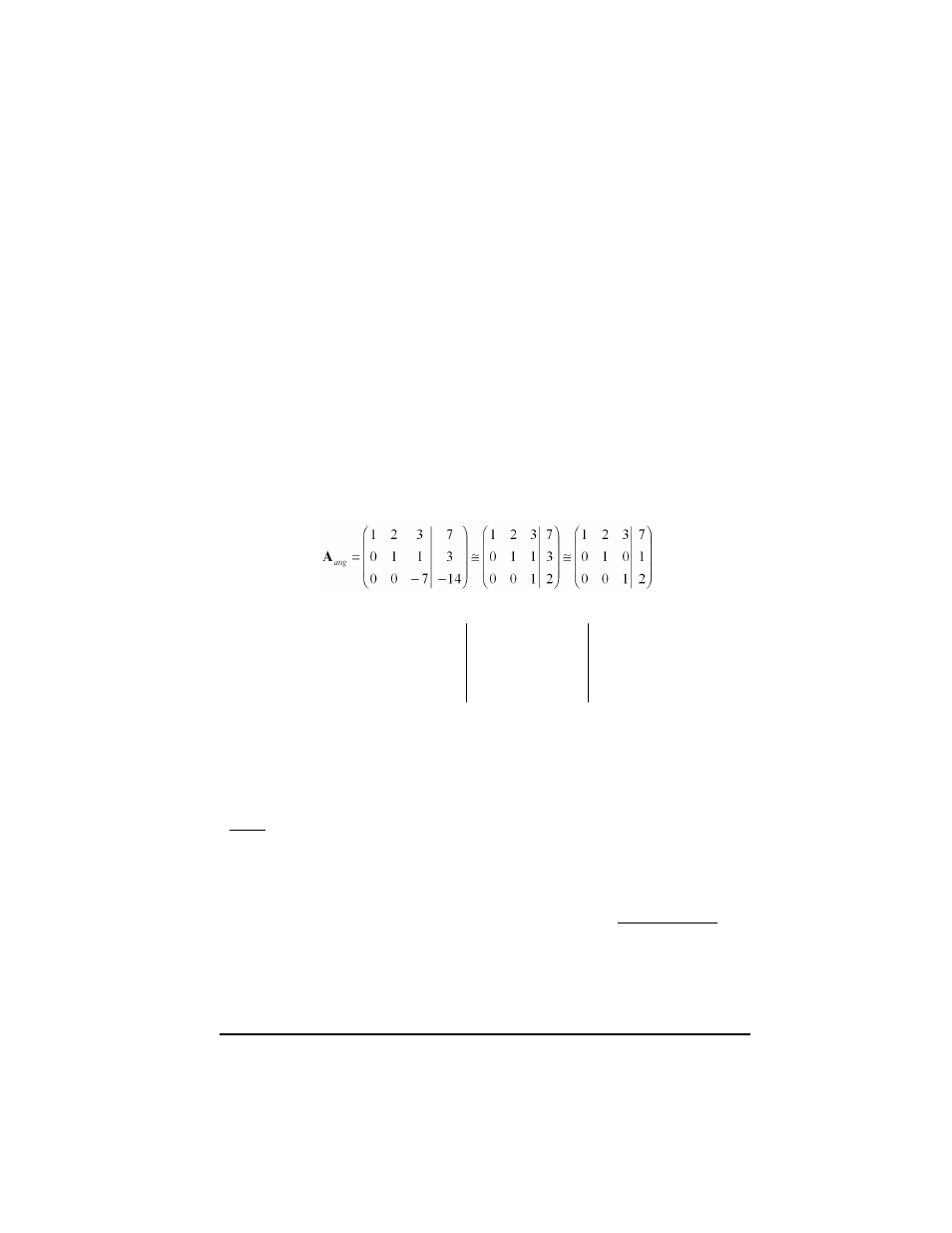
Multiply row 3 by –3, add it to row 1, replacing it:
3\#3#1@RCIJ!
Multiply row 2 by –2, add it to row 1, replacing it: 2\#2#1
@RCIJ!
Writing this process by hand will result in the following steps:
Pivoting
If you look carefully at the row operations in the examples shown above, you
will notice that many of those operations divide a row by its corresponding
element in the main diagonal. This element is called a pivot element, or simply,
a
pivot. In many situations it is possible that the pivot element become zero, in
which case we cannot divide the row by its pivot. Also, to improve the
numerical solution of a system of equations using Gaussian or Gauss-Jordan
elimination, it is recommended that the pivot be the element with the largest
absolute value in a given column. In such cases, we exchange rows before
performing row operations. This exchange of rows is called partial pivoting. To
follow this recommendation is it often necessary to exchange rows in the
augmented matrix while performing a Gaussian or Gauss-Jordan elimination.
.
2
1
1
1 0 0
0 1 0
0 0 1
2
1
1
1 0 0
0 1 0
0 2 1
⎟
⎟
⎟
⎠
⎞
⎜
⎜
⎜
⎝
⎛ −
≅
⎟
⎟
⎟
⎠
⎞
⎜
⎜
⎜
⎝
⎛
≅
aug
A