Exploring cos(x) = sin(x), Method 1: graph plot, Method 2: symbolic manipulation – Texas Instruments PLUS TI-89 User Manual
Page 406
Chapter 23: Activities 389
23ACTS.DOC TI-89/TI-92 Plus: Activities (English) Susan Gullord Revised: 02/23/01 1:24 PM Printed: 02/23/01 2:20 PM Page 389 of 26
Perform the following steps to observe where the graphs of the
functions
y1(x)=cos(x)
and
y2(x)=sin(x)
intersect.
1. In the Y= Editor, set
y1(x)=cos(x
) and
2(x)=sin(x)
.
2. In the Window Editor, set
xmin=0
and
xmax=3
p.
3. Press „ and select
A:ZoomFit
.
4. Find the intersection point of
the two functions.
5. Note the
x
and
y
coordinates.
(Repeat steps 4 and 5 to find
the other intersections.)
Perform the following steps to solve the equation
sin(x)=cos(x)
with
respect to
x
.
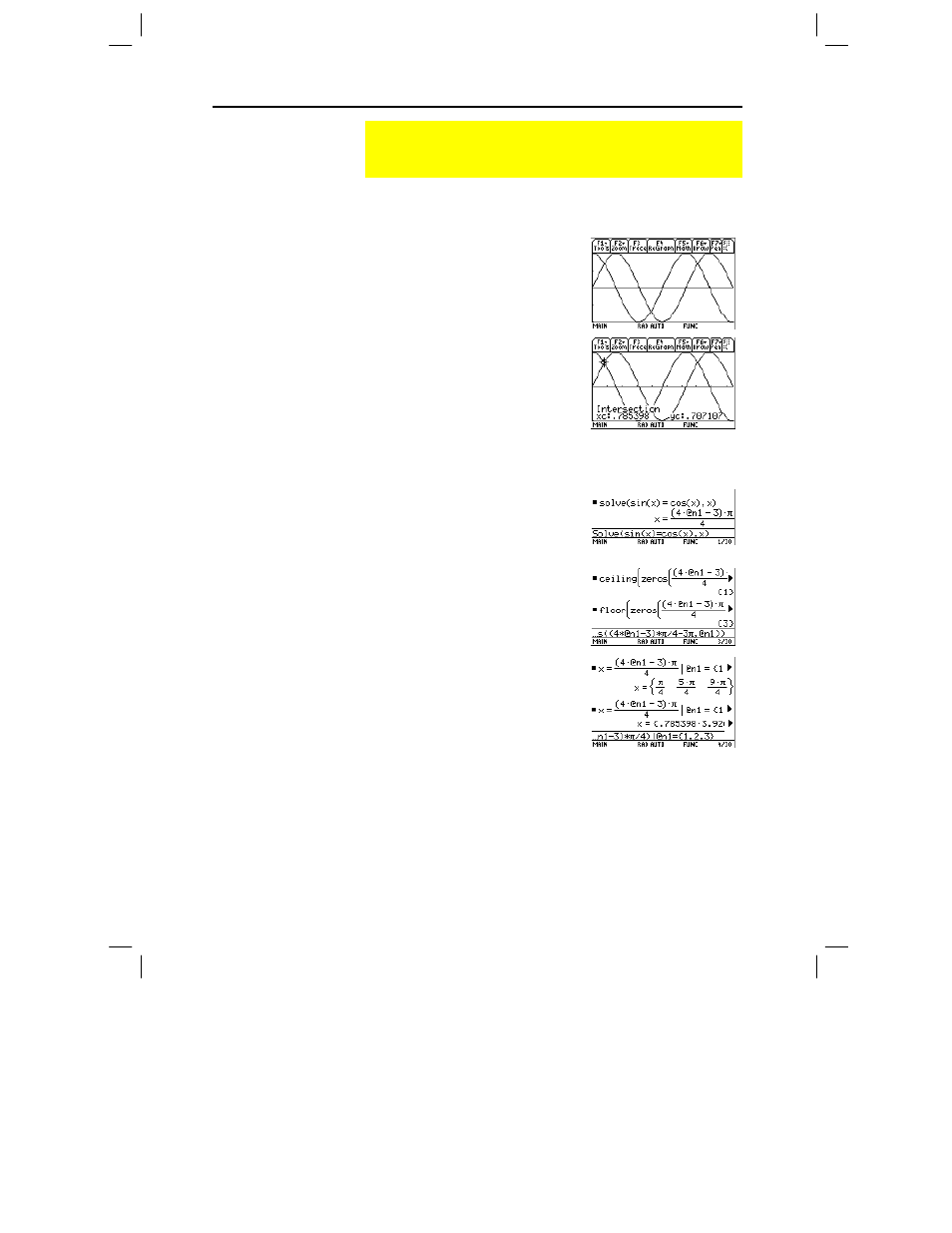
1. On the Home screen, enter
solve(sin(x)= cos(x),x)
.
The solution for
x
is where
@n1
is any integer.
2. Using the
ceiling()
and
floor()
functions, find the ceiling and
floor values for the
intersection points as shown.
3. Enter the general solution for
x
and apply the constraint for
@n1
as shown.
Compare the result with
Method 1.
Exploring cos(x) = sin(x)
This activity uses two methods to find where
cos(x) = sin(x) for
the values of x between 0 and 3
p
.
Method 1:
Graph Plot
Hint: Press
‡
and select
5:Intersection.
Respond to
the screen prompts to select
the two curves, and the
lower and upper bounds for
intersection
A
.
Method 2:
Symbolic
Manipulation
Hint: Move the cursor into
the history area to highlight
the last answer. Press
¸
to copy the result of
the general solution.
Hint: To get the “with”
operator:
TI
-
89:
Í
TI
-
92 Plus:
2
И
