Analyzing the pole-corner problem, Maximum length of pole in hallway – Texas Instruments PLUS TI-89 User Manual
Page 401
384 Chapter 23: Activities
23ACTS.DOC TI-89/TI-92 Plus: Activities (English) Susan Gullord Revised: 02/23/01 1:24 PM Printed: 02/23/01 2:20 PM Page 384 of 26
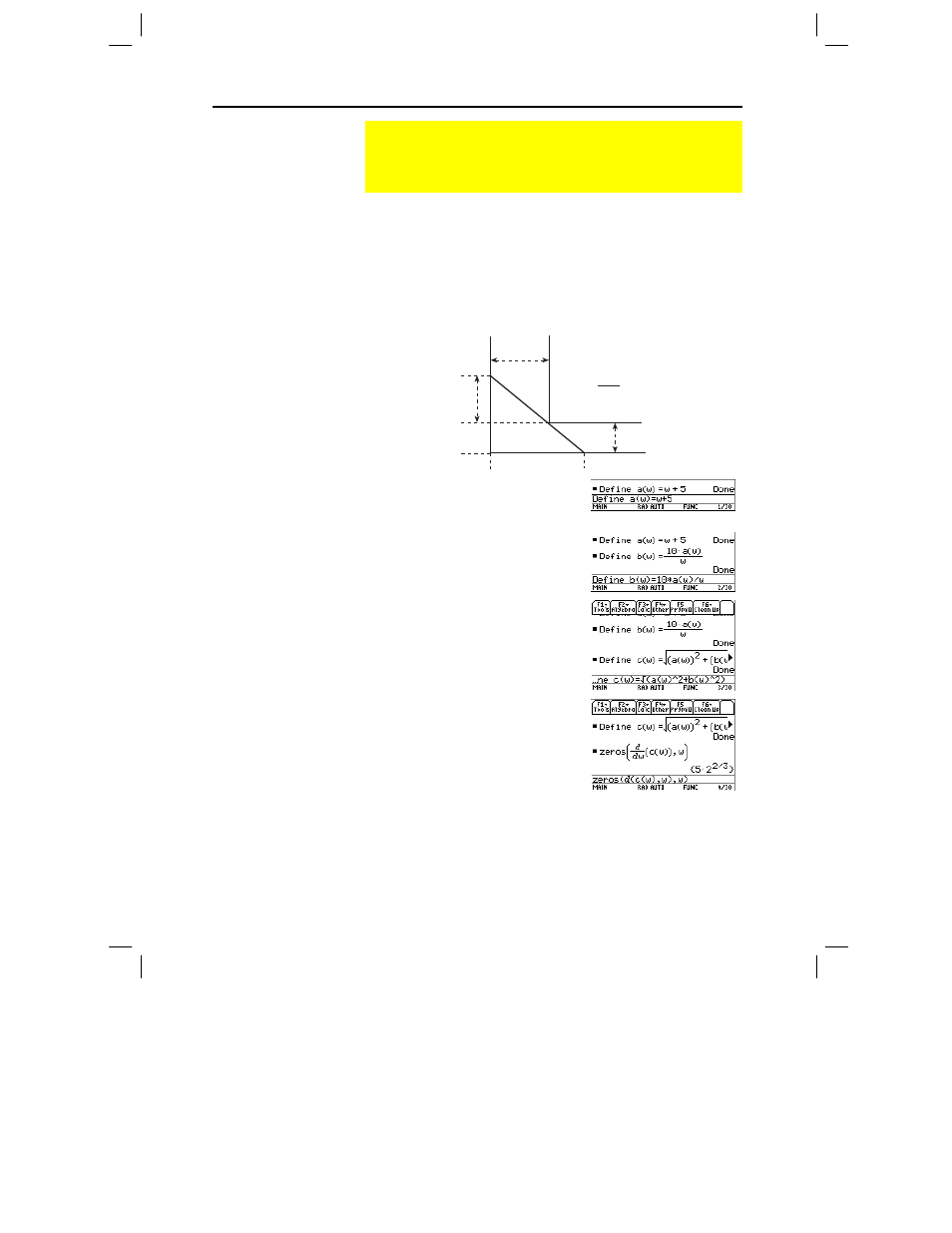
The maximum length of a pole
c
is the shortest line segment touching
the interior corner and opposite sides of the two hallways as shown
in the diagram below.
Hint:
Use proportional sides and the Pythagorean theorem to find
the length
c
with respect to
w
. Then find the zeros of the first
derivative of
c(w)
. The minimum value of
c(w)
is the maximum length
of the pole.
10
5
w
a
b
c
a = w+5
b = 10a
w
1.
Define
the expression for side
a
in terms of
w
and store it in
a(w)
.
2.
Define
the expression for side
b
in terms of
w
and store it in
b(w)
.
3.
Define
the expression for side
c
in terms of
w
and store it in
c(w)
Enter:
Define c(w)=
‡
(a(w)^2+b(w)^2)
4. Use the
zeros()
function to
compute the zeros of the first
derivative of
c(w)
to find the
minimum value of
c(w)
.
Analyzing the Pole-Corner Problem
A ten-foot-wide hallway meets a five-foot-wide hallway in the
corner of a building. Find the maximum length pole that can be
moved around the corner without tilting the pole.
Maximum Length of
Pole in Hallway
Tip: When you want to
define a function, use
multiple character names as
you build the definition.
Note: The maximum length
of the pole is the minimum
value of c(w).
