HP 48gII User Manual
Page 561
Page 17-8
(Continuous FUNctions) and define the following functions (change to Approx
mode):
Gamma pdf:
'gpdf(x) = x^(
α-1)*EXP(-x/β)/(β^α*GAMMA(α))'
Gamma cdf:
'gcdf(x) =
∫(0,x,gpdf(t),t)'
Beta pdf:
' βpdf(x)= GAMMA(α+β)*x^(α-1)*(1-x)^(β-1)/(GAMMA(α)*GAMMA(β))'
Beta cdf:
'
βc
df(x)
=
∫(0,x, βpdf(t),t)'
Exponential pdf:
'epdf(x) = EXP(-x/
β)/β'
Exponential cdf:
'ecdf(x) = 1 - EXP(-x/
β)'
Weibull pdf:
'Wpdf(x) =
α*β*x^(β-1)*EXP(-α*x^β)'
Weibull cdf:
'Wcdf(x) = 1 - EXP(-
α*x^β)'
Use function DEFINE to define all these functions. Next, enter the values of
α
and
β, e.g., 1K~‚a` 2K ~‚b`
Finally, for the cdf for Gamma and Beta cdf’s, you need to edit the program
definitions to add NUM to the programs produced by function DEFINE. For
example, the Gamma cdf, i.e., the function gcdf, should be modified to read:
«
x
' NUM(
∫ (0,x,gpdf(t),t))' » and stored back into
@gcdf
.
Repeat the procedure for
βcdf.
Unlike the discrete functions defined earlier, the continuous functions defined
in this section do not include their parameters (
α and/or β) in their definitions.
Therefore, you don't need to enter them in the display to calculate the
functions. However, those parameters must be previously defined by storing
the corresponding values in the variables
α and β. Once all functions and the
values
α and β have been stored, you can order the menu labels by using
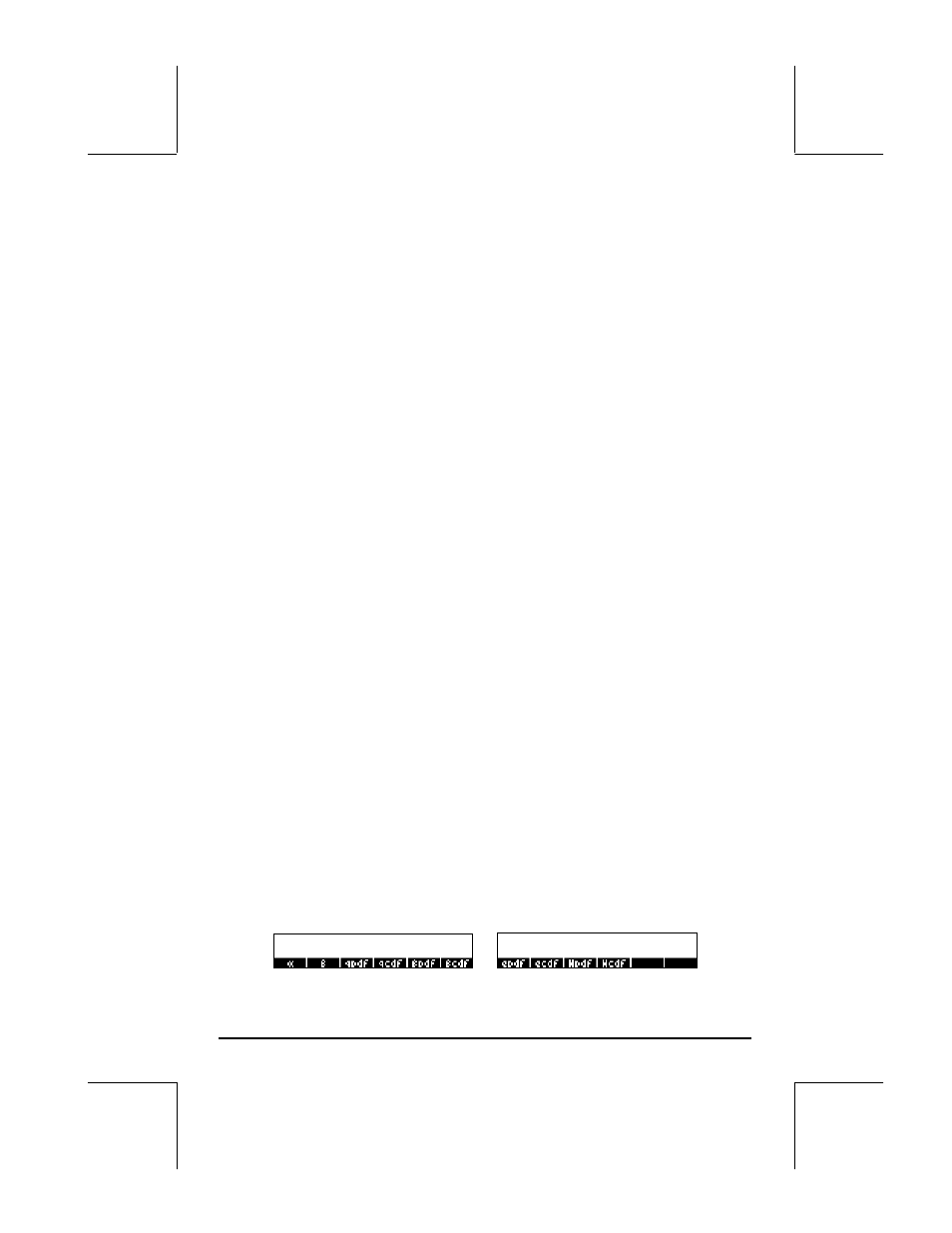
function ORDER. The call to the function will be the following:
ORDER({‘
α’,’β’,’gpdf’,’gcdf’,’βpdf’,’βcdf’,’epdf’,’ecdf’,’Wpdf’,’Wcdf’})
Following this command the menu labels will show as follows (Press
L to
move to the second list. Press
L once more to move to the first list):
