HP 48gII - Manual (Page 482)

Page 16-4
The CALC/DIFF menu
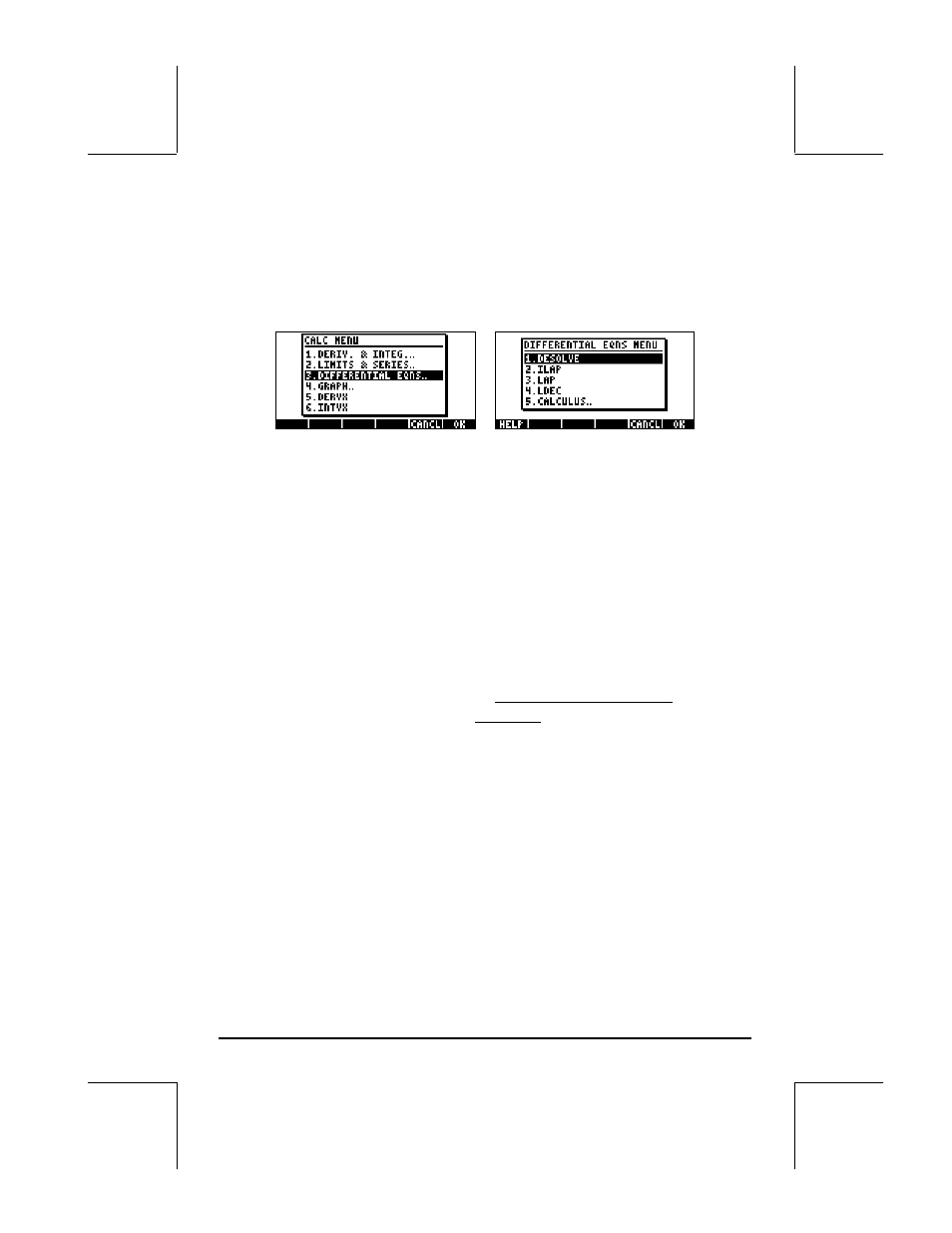
The DIFFERENTIAL EQNS.. sub-menu within the CALC ( „Ö) menu
provides functions for the solution of differential equations. The menu is listed
below with system flag 117 set to CHOOSE boxes:
These functions are briefly described next. They will be described in more
detail in later parts of this Chapter.
DESOLVE: Differential Equation SOLVEr, provides a solution if possible
ILAP: Inverse LAPlace transform, L
-1 [F(s)] = f(t)
LAP: LAPlace transform, L[f(t)]=F(s)
LDEC: solves Linear Differential Equations with Constant coefficients, including
systems of differential equations with constant coefficients
Solution to linear and non-linear equations
An equation in which the dependent variable and all its pertinent derivatives
are of the first degree is referred to as a linear differential equation.
Otherwise, the equation is said to be non-linear. Examples of linear
differential equations are: d
2 x/dt 2 + β⋅(dx/dt) + ω
o
⋅x = A sin ω
f
t, and ∂C/∂t
+ u⋅(∂C/∂x) = D⋅(∂ 2 C/∂x 2 ).
An equation whose right-hand side (not involving the function or its derivatives)
is equal to zero is called a homogeneous equation. Otherwise, it is called
non-homogeneous. The solution to the homogeneous equation is known as a
general solution. A particular solution is one that satisfies the non-
homogeneous equation.