Casio 330 - Manual (Page 442)

20090601
2-Sample t Test
Menu: [Test]-[Two-Sample TTest]
Description: This command compares the population means of two populations when
population standard deviation is unknown. A 2-Sample t Test is used for t
distribution.
M
1
: sample mean of sample 1 data
M
2
: sample mean of sample 2 data
s
x 1
: sample standard deviation of sample 1
s
x 2
: sample standard deviation of sample 2
n
1
: size of sample 1
n
2
: size of sample 2
This formula is applicable when the population standard deviations of the two
populations are not equal. The denominator is different when the population
standard deviations are equal.
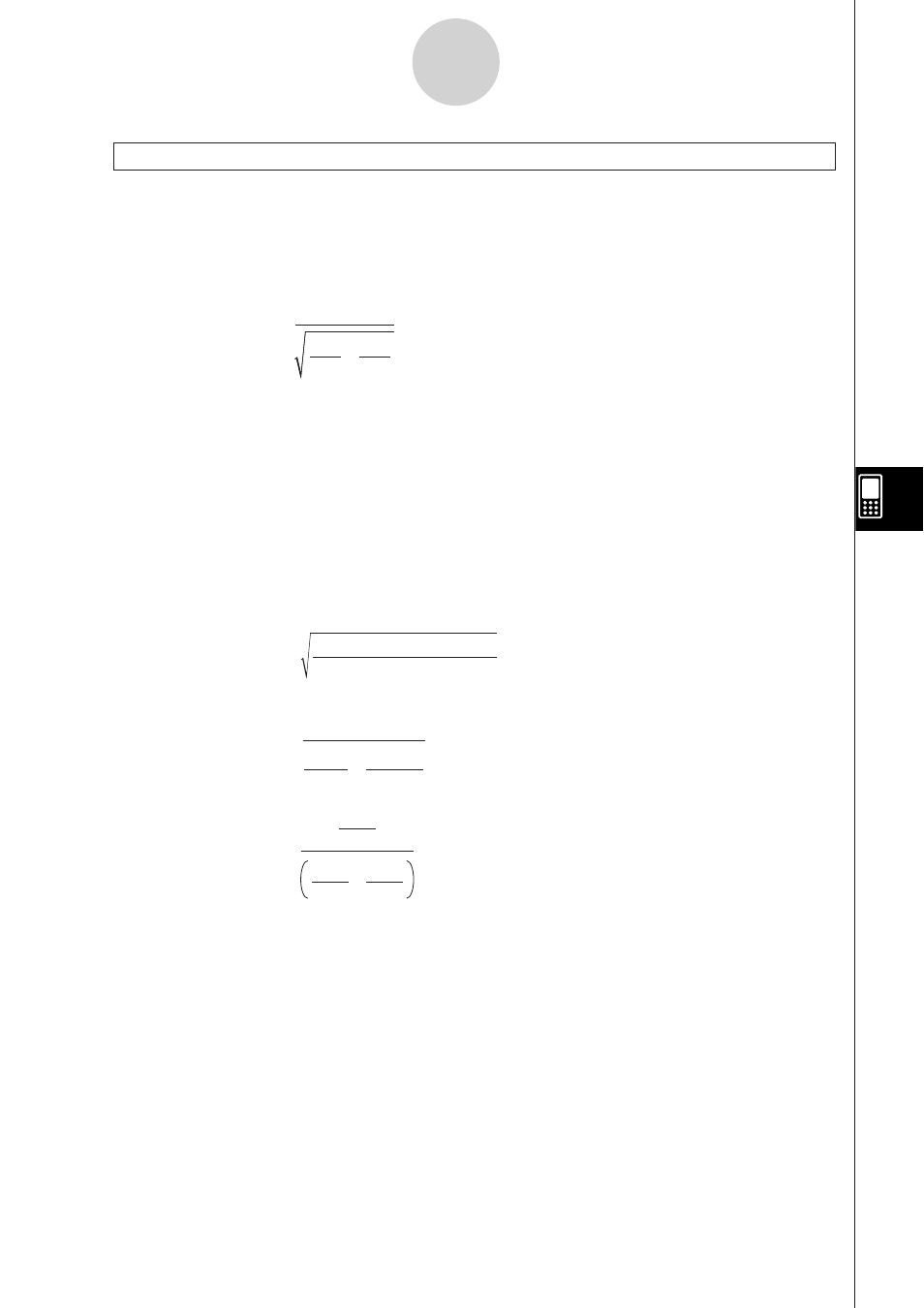
The t distribution degrees of freedom df and s
p
differ according to whether the
population standard deviations of the two populations are equal.
When the two population standard deviations are equal (pooled)
df n
1
+ n
2
– 2
s
p
=
n
1
+ n
2
– 2
(n
1
–1)s
x 1
2 +(n
2
–1)s
x 2
2
When the two population standard deviations are not equal (not pooled)
df =
1
C 2
n
1
–1
+
(1–C ) 2
n
2
–1
C =
n
1
+ n
2
n
1
s
x 1
2 s
x 2
2
s
x 1
2
Definition of Terms
μ
1
condition : sample mean value test conditions (“ x” specifies two-tail test, “<”
specifies one-tail test where sample 1 is smaller than sample 2, “>”
specifies one-tail test where sample 1 is greater than sample 2.)
List(1) : list where sample 1 data is located
List(2) : list where sample 2 data is located
Freq(1) : frequency of sample 1 (1 or list name)
Freq(2) : frequency of sample 2 (1 or list name)
Pooled : On or Off
M
1
: sample mean of sample 1 data
s
x 1
: sample standard deviation of sample 1 (s
x 1
> 0)
n
1
: size of sample 1 (positive integer)
M
2
: sample mean of sample 2 data
s
x 2
: sample standard deviation of sample 2 (s
x 2
> 0)
n
2
: size of sample 2 (positive integer)
t
=
M
1
—
M
2
n
1
+
s
x 1
2
n
2
s
x 2
2
t
=
M
1
—
M
2
n
1
+
s
x 1
2
n
2
s
x 2
2
7-9-10
Tests