HP 49g+ - Manual (Page 593)

Page 18-26
Confidence intervals for sums and differences of mean values
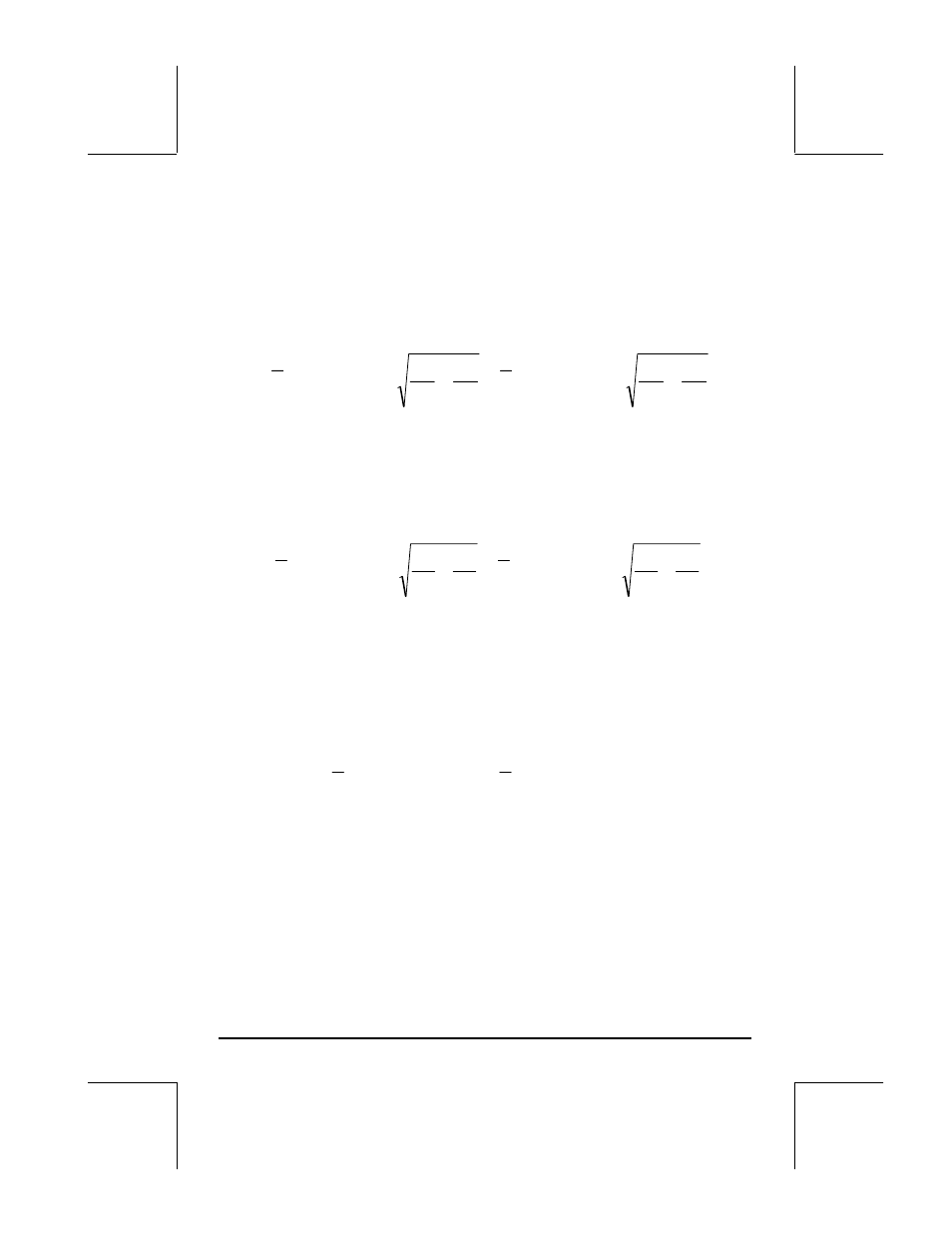
If the population variances σ
1
2 and σ
2
2 are known, the confidence intervals for
the difference and sum of the mean values of the populations, i.e., µ
1
±µ
2
, are
given by:








+ ⋅ + ± + ⋅ − ±
2
2
2
1
2
1
2 / 2 1
2
2
2
1
2
1
2 / 2 1
) ( , ) (
n n
z X X
n n
z X X
σ σ σ σ
α α
For large samples, i.e., n
1
> 30 and n
2
> 30, and unknown, but equal,
population variances σ
1
2 = σ
2
2 , the confidence intervals for the difference and
sum of the mean values of the populations, i.e., µ
1
±µ
2
, are given by:
. ) ( , ) (
2
2
2
1
2
1
2 / 2 1
2
2
2
1
2
1
2 / 2 1 







+ ⋅ + ± + ⋅ − ±
n
S
n
S
z X X
n
S
n
S
z X X
α α
If one of the samples is small, i.e., n
1
< 30 or n
2
< 30, and with unknown, but
equal, population variances σ
1
2 = σ
2
2 , we can obtain a “pooled” estimate of
the variance of µ
1
±µ
2
, as s
p
2 = [(n
1
-1) ⋅s
1
2 +(n
2
-1) ⋅s
2
2 ]/( n
1
+n
2
-2).
In this case, the centered confidence intervals for the sum and difference of
the mean values of the populations, i.e.,
µ
1
±µ
2
, are given by:
( )
2
2 / , 2 1
2
2 / , 2 1
) ( , ) (
p p
s t X X s t X X ⋅ + ± ⋅ − ±
α ν α ν
where
ν = n
1
+n
2
-2 is the number of degrees of freedom in the Student’s t
distribution.
In the last two options we specify that the population variances, although
unknown, must be equal. This will be the case in which the two samples are
taken from the same population, or from two populations about which we
suspect that they have the same population variance. However, if we have