HP 49g+ User Manual
Page 241
Page 7-11
cosine law, and sum of interior angles of a triangle, to solve for the other
three variables.
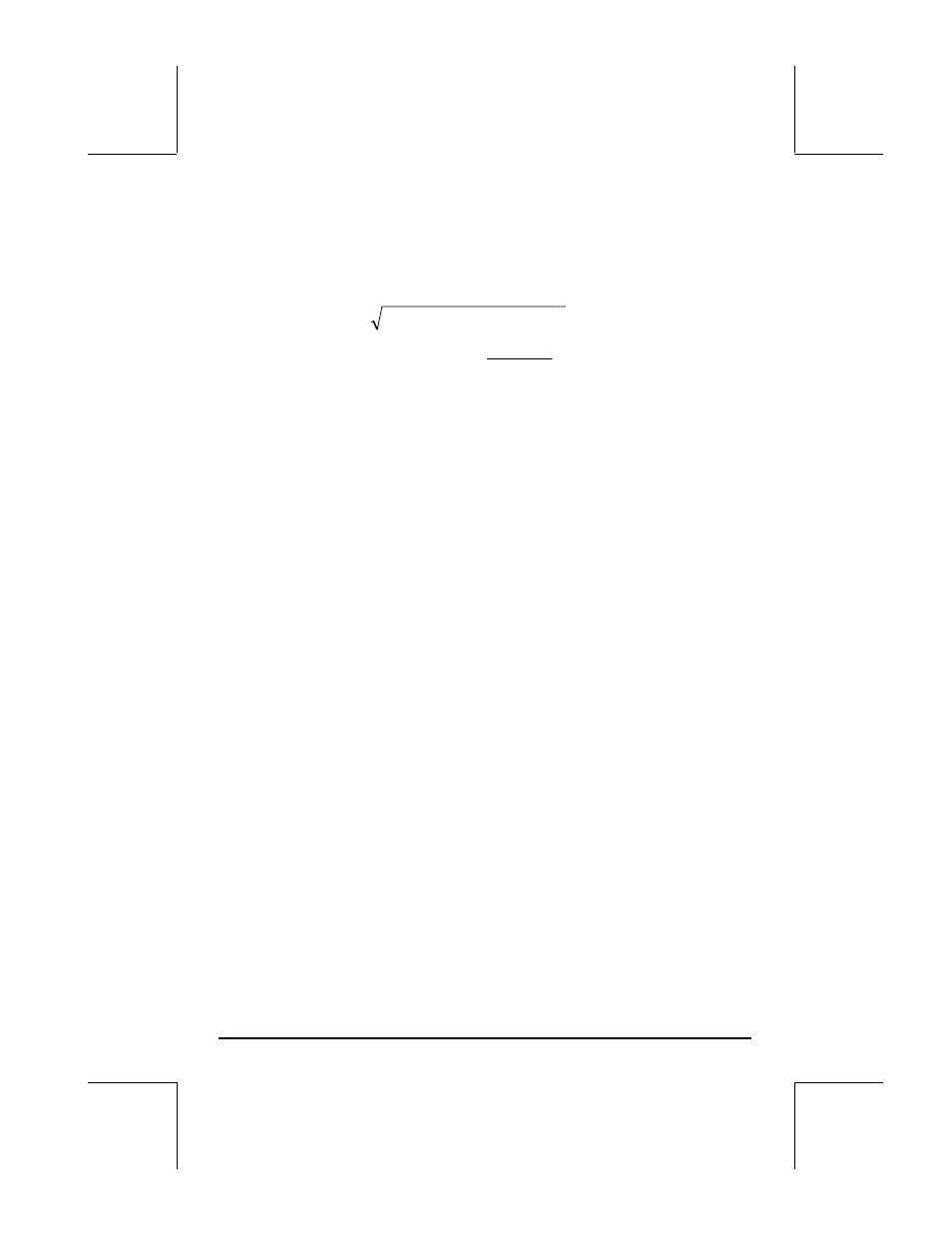
If the three sides are known, the area of the triangle can be calculated with
Heron’s formula
)
(
)
(
)
(
c
s
b
s
a
s
s
A
−
⋅
−
⋅
−
⋅
=
,where
s is known as the
semi-perimeter of the triangle, i.e.,
.
2
c
b
a
s
+
+
=
Triangle solution using the Multiple Equation Solver (MES)
The Multiple Equation Solver (MES) is a feature that can be used to solve two
or more coupled equations. It must be pointed out, however, that the MES
does not solve the equations simultaneously. Rather, it takes the known
variables, and then searches in a list of equations until it finds one that can be
solved for one of the unknown variables. Then, it searches for another
equation that can be solved for the next unknowns, and so on, until all
unknowns have been solved for.
Creating a working directory
We will use the MES to solve for triangles by creating a list of equations
corresponding to the sine and cosine laws, the law of the sum of interior
angles, and Heron’s formula for the area. First, create a sub-directory within
HOME that we will call TRIANG, and move into that directory. See Chapter
2 for instructions on how to create a new sub-directory.
Entering the list of equations
Within TRIANG, enter the following list of equations either by typing them
directly on the stack or by using the equation writer. (Recall that ~‚a
produces the character
α, and ~‚b produces the character β. The
character
γ needs to be @ECHOed from ‚±):
‘SIN(
α)/a = SIN(β)/b’
‘SIN(
α)/a = SIN(γ)/c’
‘SIN(
β)/b = SIN(γ)/c’
‘c^2 = a^2+b^2-2*a*b*COS(
γ)’
‘b^2 = a^2+c^2-2*a*c*COS(
β)’
