Properties of the fourier transform – HP 49g+ User Manual
Page 522
Page 16-48
−
−
⋅
+
⋅
=
+
⋅
=
ω
ω
ω
π
ω
π
ω
i
i
i
i
F
1
1
1
1
2
1
1
1
2
1
)
(
+
⋅
−
+
=
2
2
1
1
1
2
1
ω
ω
ω
π
i
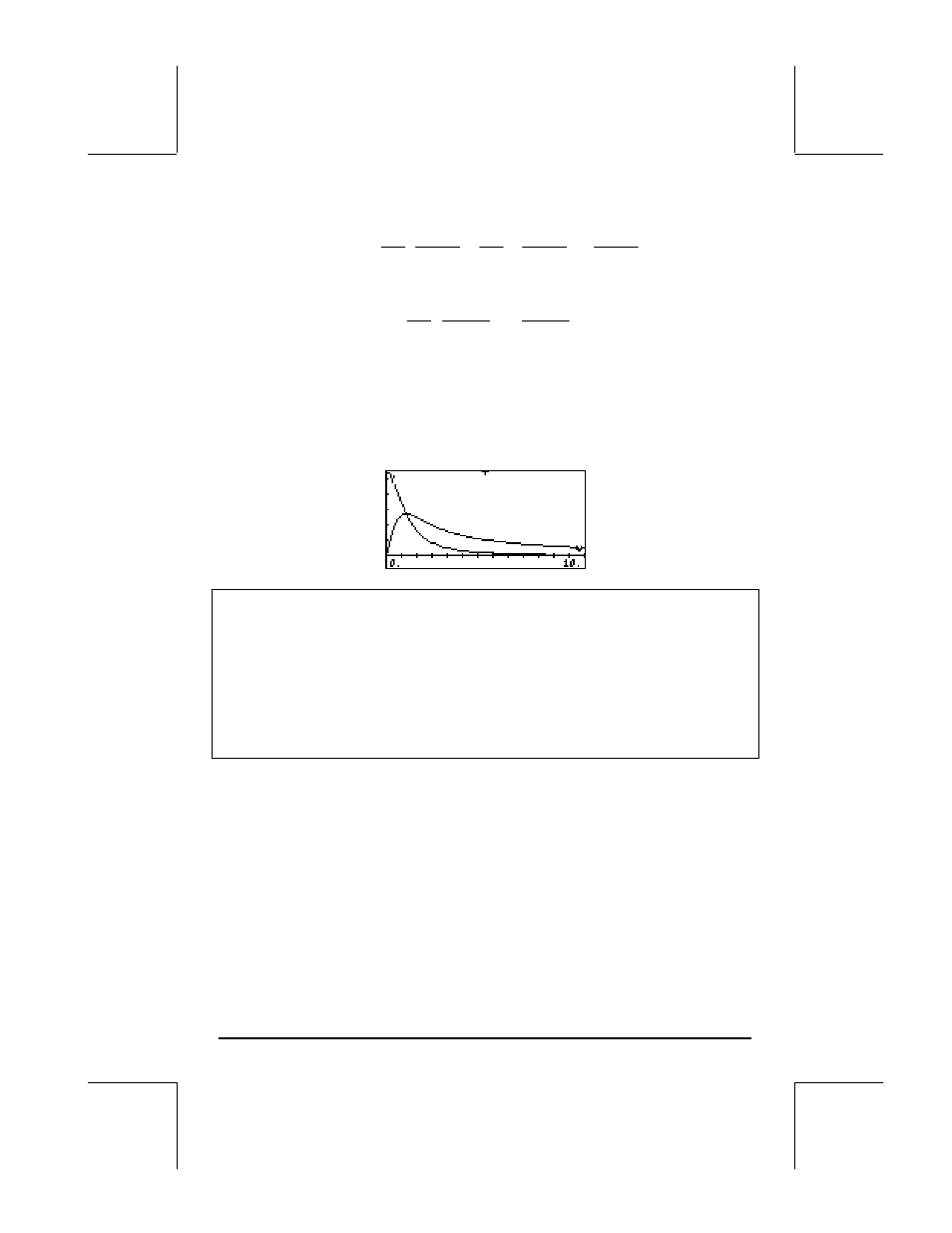
which is a complex function.
The absolute value of the real and imaginary parts of the function can be
plotted as shown below
Notes:
The magnitude, or absolute value, of the Fourier transform, |F(
ω)|, is the
frequency spectrum of the original function f(t). For the example shown above,
|F(
ω)| = 1/[2π(1+ω
2
)]
1/2
. The plot of |F(
ω)| vs. ω was shown earlier.
Some functions, such as constant values, sin x, exp(x), x
2
, etc., do not have
Fourier transform. Functions that go to zero sufficiently fast as x goes to
infinity do have Fourier transforms.
Properties of the Fourier transform
Linearity: If a and b are constants, and f and g functions, then F{a
⋅f + b⋅g} =
a F{f }+ b F{g}.
Transformation of partial derivatives. Let u = u(x,t). If the Fourier transform
transforms the variable x, then
F{
∂u/∂x} = iω F{u},
F{
∂
2
u/
∂x
2
} = -
ω
2
F{u},
F{
∂u/∂t} = ∂F{u}/∂t, F{∂
2
u/
∂t
2
} =
∂
2
F{u}/
∂t
2
