HP 49g+ User Manual
Page 215
Page 6-18
The solution can be seen from within the SOLVE EQUATION input form by
pressing
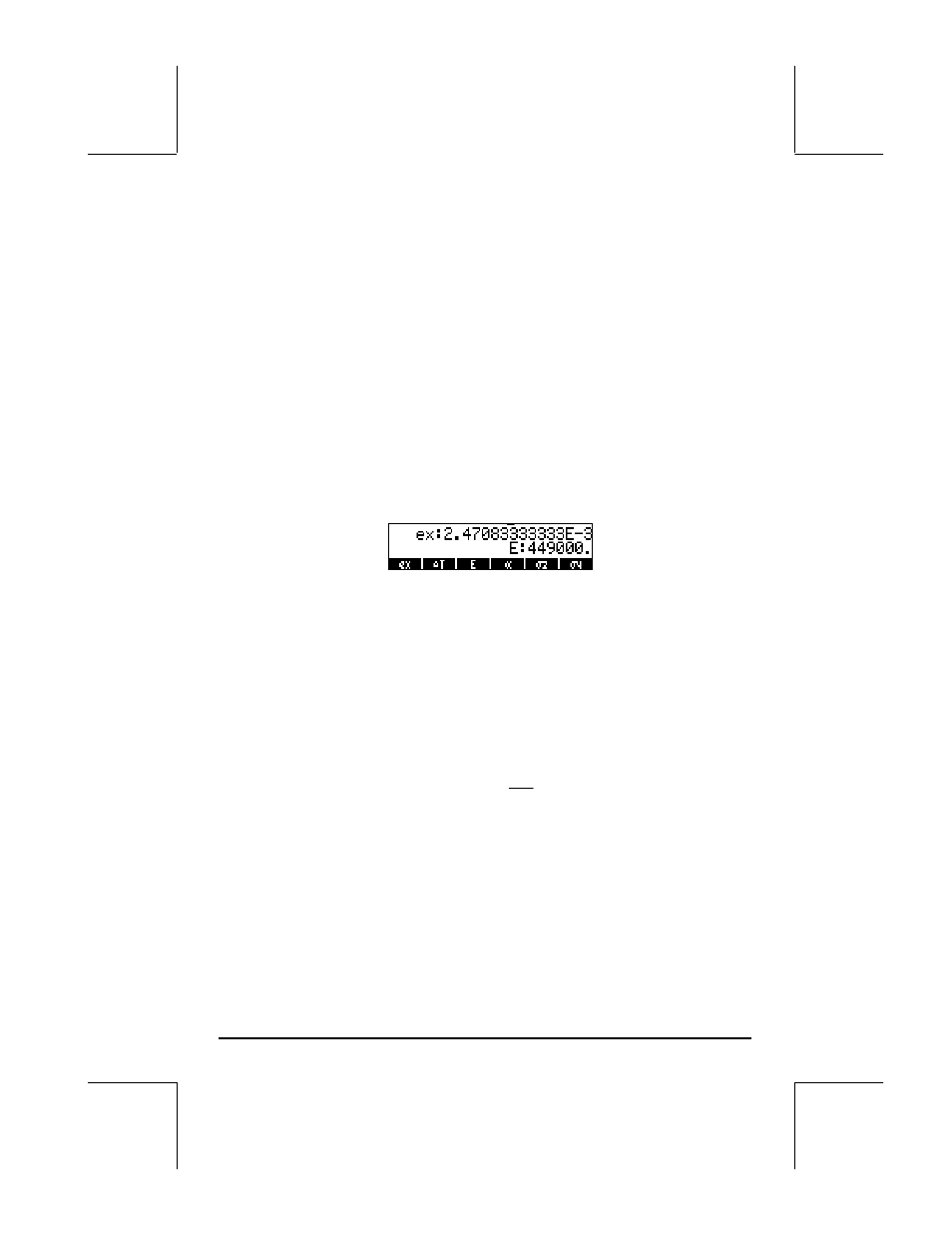
@EDIT while the ex: field is highlighted. The resulting value is
2.470833333333E-3. Press
@@@OK@@ to exit the EDIT feature.
Suppose that you now, want to determine the Young’s modulus that will
produce a strain of
e
xx
= 0.005 under the same state of stress, neglecting
thermal expansion. In this case, you should enter a value of 0.005 in the
ex:
field, and a zero in the
∆T: field (with ∆T = 0, no thermal effects are
included). To solve for E, highlight the
E: field and press @SOLVE@. The result,
seeing with the
@EDIT feature is, E = 449000 psi. Press @SOLVE@ ` to return
to normal display.
Notice that the results of the calculations performed within the numerical
solver environment have been copied to the stack:
Also, you will see in your soft-menu key labels variables corresponding to
those variables in the equation stored in EQ (press
L to see all variables in
your directory), i.e., variables
ex, ∆T, α, σz, σy, n, σx, and E.
Example 2 – Specific energy in open channel flow
Specific energy in an open channel is defined as the energy per unit weight
measured with respect to the channel bottom. Let E = specific energy, y =
channel depth, V = flow velocity, g = acceleration of gravity, then we write
The flow velocity, in turn, is given by V = Q/A, where Q = water discharge,
A = cross-sectional area. The area depends on the cross-section used, for
example, for a trapezoidal cross-section, as shown in the figure below, A =
(b+m
⋅y) ⋅y, where b = bottom width, and m = side slope of cross section.
.
2
2
g
V
y
E
+
=
