Simple operations with complex numbers, Changing sign of a complex number – HP 49g+ User Manual
Page 162
Page 4-4
Simple operations with complex numbers
Complex numbers can be combined using the four fundamental operations
(
+-*/). The results follow the rules of algebra with the caveat that
i
2
= -1. Operations with complex numbers are similar to those with real
numbers. For example, with the calculator in ALG mode and the CAS set to
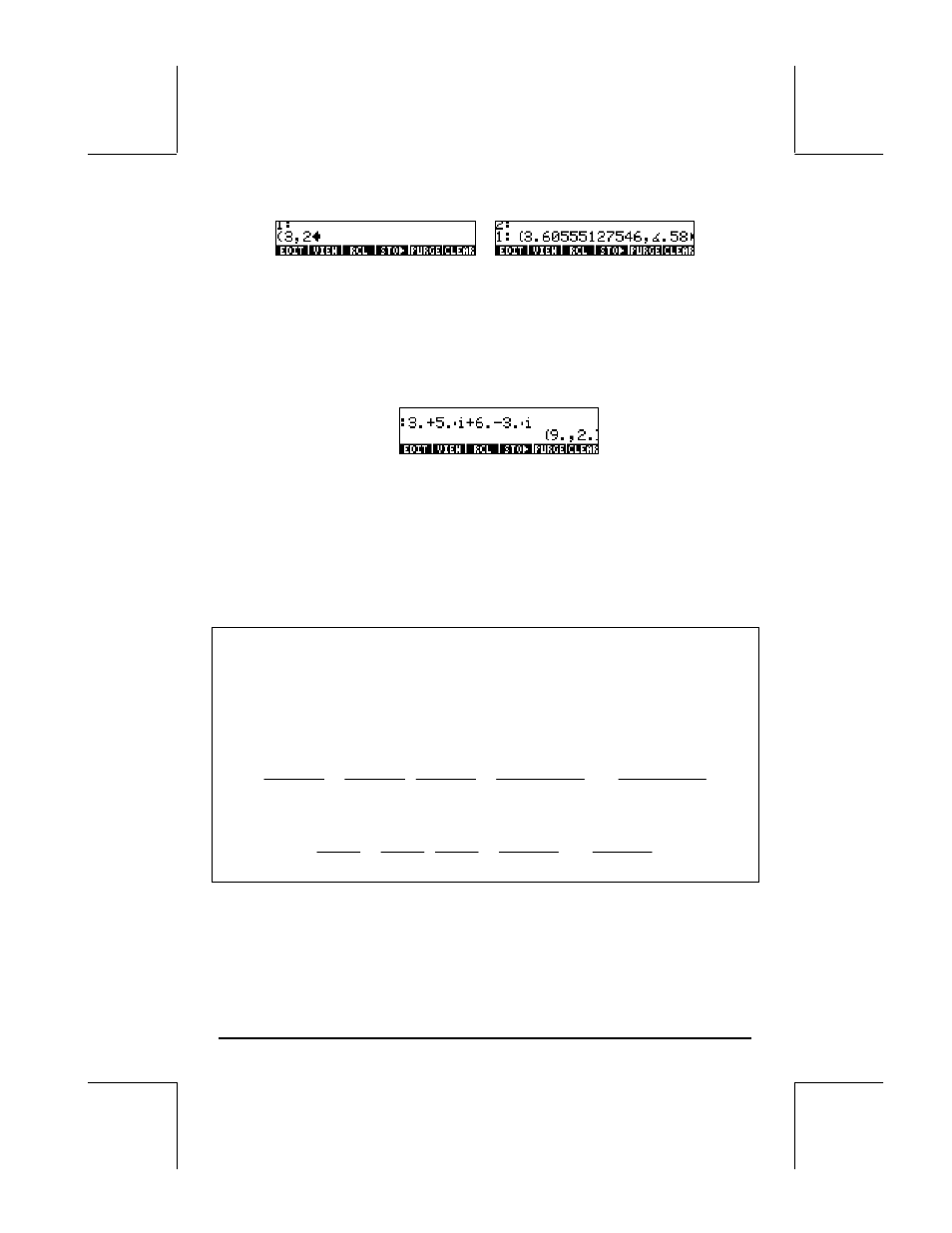
Complex, we’ll attempt the following sum: (3+5i) + (6-3i):
Notice that the real parts (3+6) and imaginary parts (5-3) are combined
together and the result given as an ordered pair with real part 9 and
imaginary part 2. Try the following operations on your own:
(5-2i) - (3+4i) = (2,-6)
(3-i) (2-4i) = (2,-14)
(5-2i)/(3+4i) = (0.28,-1.04)
1/(3+4i) = (0.12, -0.16)
Notes:
The product of two numbers is represented by: (x
1
+iy
1
)(x
2
+iy
2
) = (x
1
x
2
- y
1
y
2
) +
i (x
1
y
2
+ x
2
y
1
).
The division of two complex numbers is accomplished by multiplying both
numerator and denominator by the complex conjugate of the denominator,
i.e.,
2
2
2
2
2
1
1
2
2
2
2
2
2
1
2
1
2
2
2
2
2
2
1
1
2
2
1
1
y
x
y
x
y
x
i
y
x
y
y
x
x
iy
x
iy
x
iy
x
iy
x
iy
x
iy
x
+
−
⋅
+
+
+
=
−
−
⋅
+
+
=
+
+
Thus, the inverse function INV (activated with the
Y key) is defined as
2
2
2
2
1
1
y
x
y
i
y
x
x
iy
x
iy
x
iy
x
iy
x
+
⋅
+
+
=
−
−
⋅
+
=
+
Changing sign of a complex number
Changing the sign of a complex number can be accomplished by using the
\ key, e.g., -(5-3i) = -5 + 3i
