Laplace transforms, Definitions – HP 49g+ User Manual
Page 484
Page 16-10
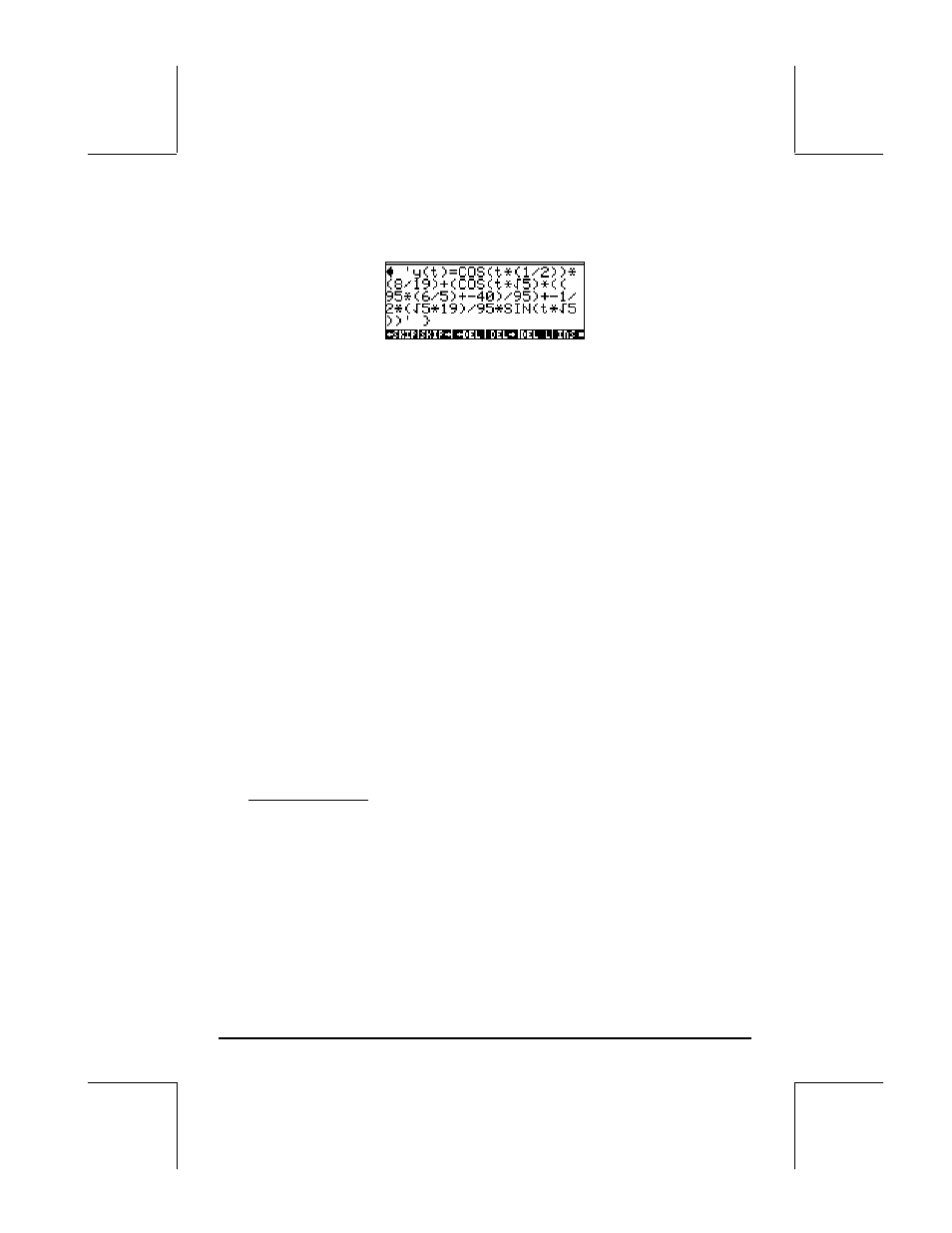
The solution is:
Press
µµto simplify the result to
‘y(t) = -((19*
√5*SIN(√5*t)-(148*COS(√5*t)+80*COS(t/2)))/190)’.
Press
J @ODETY to get the string “
Linear w/ cst coeff
” for the ODE type
in this case.
Laplace Transforms
The Laplace transform of a function f(t) produces a function F(s) in the image
domain that can be utilized to find the solution of a linear differential equation
involving f(t) through algebraic methods. The steps involved in this
application are three:
1. Use of the Laplace transform converts the linear ODE involving f(t) into an
algebraic equation.
2. The unknown F(s) is solved for in the image domain through algebraic
manipulation.
3. An inverse Laplace transform is used to convert the image function found
in step 2 into the solution to the differential equation f(t).
Definitions
The Laplace transform for function f(t) is the function F(s) defined as
The image variable s can be, and it generally is, a complex number.
Many practical applications of Laplace transforms involve an original function
f(t) where t represents time, e.g., control systems in electric or hydraulic
∫
∞
−
⋅
=
=
0
.
)
(
)
(
)}
(
{
dt
e
t
f
s
F
t
f
st
L
