Kipp&Zonen BSRN Scientific Solar Monitoring System User Manual
Page 129
117
Country
R
mm
r
mm
L
mm
Slope
angle
Limit
angle
Opening
angle
Remark
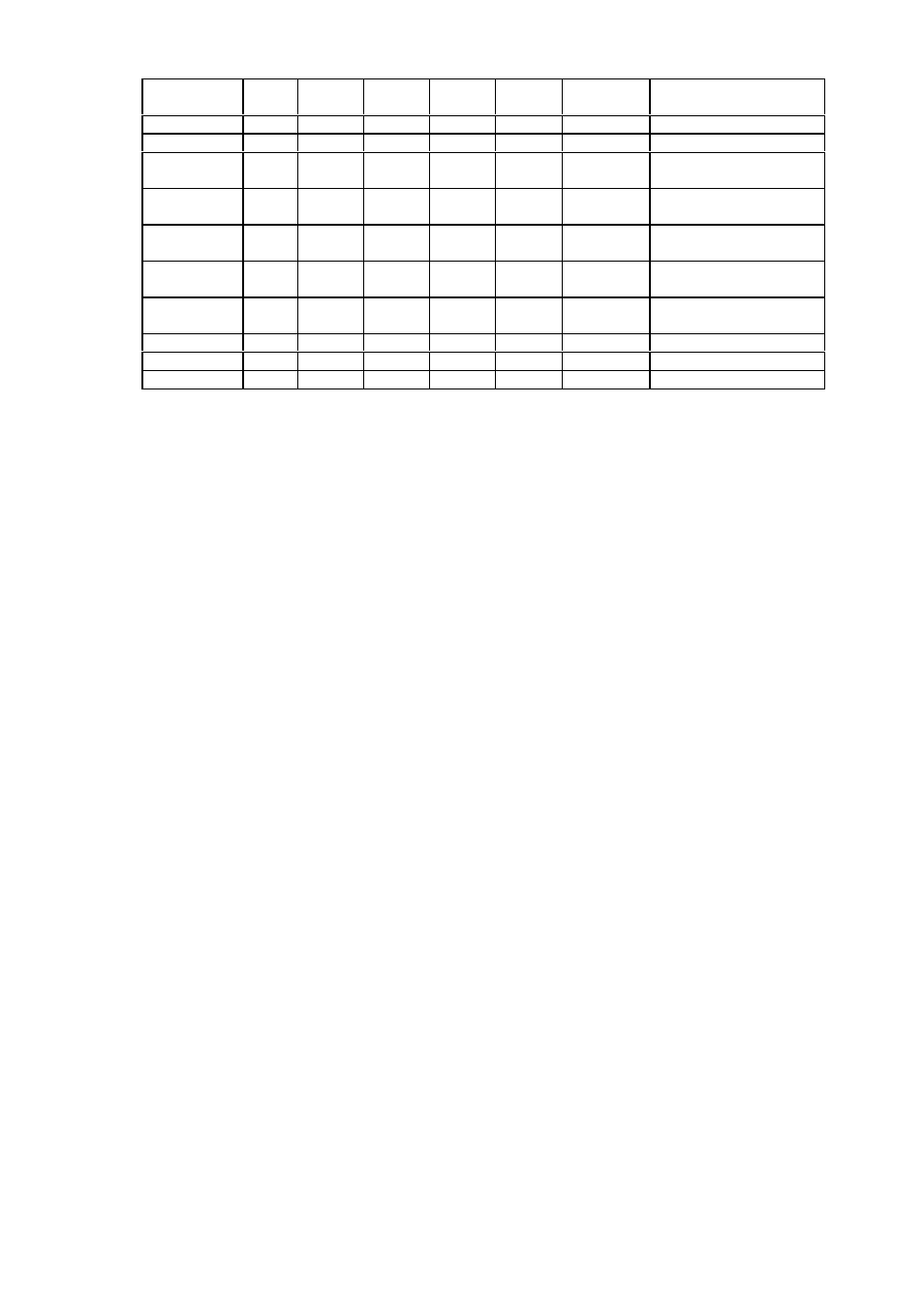
Australia I
34.8
10
795
1.79
3.23
2.51
Sky solar
Australia II
34.8
5.64
795
2.10
2.91
2.51
Sky infrared
Austria
45
16
500
3.32
6.96
5.14
To calibrate star
pyranom eters
Germ any I
25
10
298
2.88
6.70
4.79
To fit to Linke-Feussner
pyrheliom eter
Germ any II
30
10
603
1.90
3.80
2.85
To fit to NIP
pyrheliom eter
Germ any III
30
10
687
1.67
3.33
2.50
BSRN station
diffusom eter
Hungary I
25.4
10
577
1.53
3.51
2.52
SciTec-KIPP
diffusom eter
Hungary II
30
10
577
1.74
3.72
2.73
Experim ental 1
Hungary III
34
10
577
2.23
4.21
3.22
Experim ental 2
USA
30.17
5.64
603.3
2.33
3.40
2.86
NOAA diffusom eters
Table C 2.1. Geom etrical data of diffusom eters.
R: radius of the shading disk or sphere; r: radius of the pyranometer sensing area;
L: distance between shader and sensor. The slope, limit and opening angles are valid when the shader
is above the pyranometer (Sun at zenith)
C 2.2.1
The penum bra functions.
In the case of circular pyrheliom eters and diffusom eters (when rotational sym m etry exists around the
optical axis of the instrum ent) the penum bra functions can be calculated by the relatively sim ple form ula
of Pastiels (see Major 1994). This holds for diffusom eters only then, when the Sun is in the zenith.
At lower solar elevations the rotational sym m etry of diffusom eters is not valid, since the radiation receiving
surface is not perpendicular to the optical axis. W ith deacreasing solar elevation the slope and lim it
angles tend to the (half)opening angle (Major 1992, 1994). In this case the geom etrical penum bra depends
also on the azim uth angle m easured around the optical axis in the plane perpendicular to it. To calculate
the penum bra function for any position of shader num erical sim ulation should be used (Major 1994).
In Figures C 2.1a and C 2.1b the penum bra functions (averaged in azim uth) are shown for the
diffusom eters listed in Table C 2.1. As it is seen from Figure C 2.1 the sm allest diffuse radiation is
m easured by the Austrian diffusom eter (largest shaded area around the Sun), while the largest one
m ight belong to any of a group of instrum ents (Australian I, Australian II, Germ an III, Hungarian I).
C 2.2.2
Sky functions
It is a century long effort to derive reliable (spectrally integrated) circum solar sky functions. For clear
atm osphere calculations can be m ade using aerosol m odels. In this respect Deirmendjian (1959),
Frohlich and Quenzel (1974), Thomalla et al. (1983) and Putsay (1995) published results usable for
pyrheliom etric/diffusom etric purposes. Their results are identical for identical aerosol conditions. The
m easurem ent of circum solar radiation is m ore com plicated than that of diffuse or direct solar radiation.
Statistically significant am ount of such m easurem ents has only been m ade available in 1991 by the
National Renewable Energy Laboratory (NREL), USA (Noring, Grether and Hunt). This database contains
170000 sky functions collected in eleven sites in the United States and cover a wide range of solar
elevations and atm ospheric conditions. The NREL kindly provided us with the data m easured in April
and May of 1977 at Boardm an (Oregon). This dataset contains about 2000 functions of radiance distribution
along the solar disc and the aureola up to 3.2 from the solar center.
0
