HEIDENHAIN TNC 310 (286 140) - Manual (Page 93)

81 HEIDENHAIN TNC 310
6.5 P
ath Cont
ours
—
P
olar Coor
dinat
es
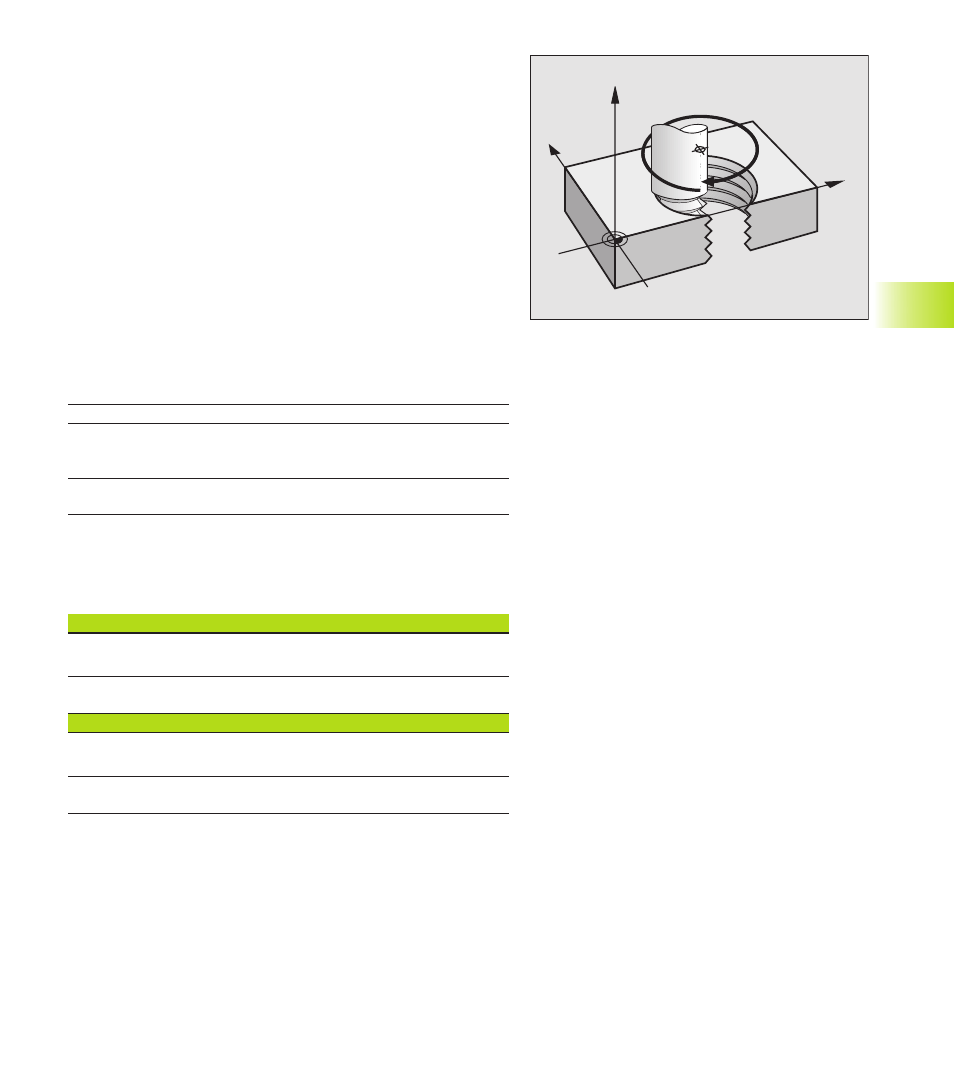
Helical interpolation
A helix is a combination of a circular movement in a main plane and
a linear movement perpendicular to this plane.
A helix is programmed only in polar coordinates.
Application
■ Large-diameter internal and external threads
■ Lubrication grooves
Calculating the helix
To program a helix, you must enter the total angle through which
the tool is to move on the helix in incremental dimensions, and the
total height of the helix.
For calculating a helix that is to be cut in a upward direction, you
need the following data:
Y
X
Z
CC
Thread revolutions n
Total height h
Incremental
total angle IPA
Starting coordinate Z
Thread revolutions + thread overrun at
the start and end of the thread
Thread pitch P x thread revolutions n
Thread revolutions x 360° + angle for
beginning of thread + angle for thread
overrun
Thread pitch P x (thread revolutions +
thread overrun at start of thread)
Shape of the helix
The table below illustrates in which way the shape of the helix is
determined by the work direction, direction of rotation and radius
compensation.
Internal thread Work direction Direction Radius compensation
Right-handed Z+ DR+ RL
Left-handed Z+ DR– RR
Right-handed Z– DR– RR
Left-handed Z– DR+ RL
External thread
Right-handed Z+ DR+ RR
Left-handed Z+ DR– RL
Right-handed Z– DR– RL
Left-handed Z– DR+ RR
6.5 P
ath Cont
ours
—
P
olar Coor
dinat
es