BUCHI NIRCal - Manual (Page 181)

Chemometrics
NIRCal 5.5 Manual, Version A 181
3.18.40 Durbin-Watson Factor Statistics
Description Statistical test for the determination of the linearity.
Use Addition information for statistics like regression slope and intercept.
Method PCR / PLS / Cluster (CLU) / SIMCA
Matrices ID 62
Tip The C-Set and V-Set selection has a big influence on the Durbin-Watson
value.
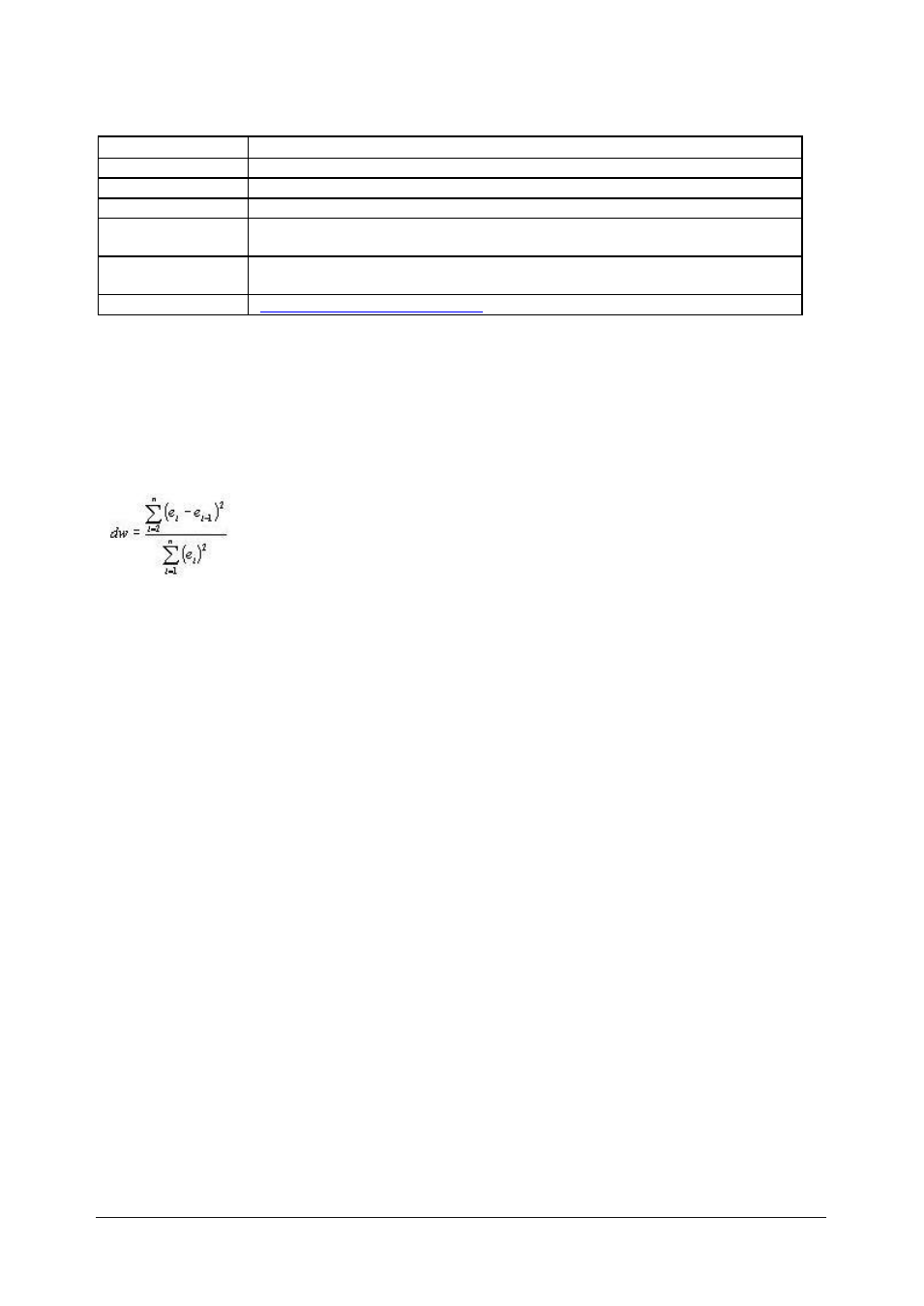
Details dw = Durbin-Watson = Sum of consecutive/successive Residual Difference
Square / Sum of Residual Square
Related Topic Calibration Protocol Quantitative
Definition: "The Durbin-Watson test is a test for first-order serial correlation in the residuals of a time
series regression. A value of 2.0 for the Durbin-Watson statistic indicates that there is no serial
correlation. This result is biased toward the finding that there is no serial correlation if lagged values of
the regressors are in the regression.
Formally, the statistic is: d=(sum from t=2 to t=T of: (et-et-1)2/(sum from t=1 to t=T of: et2) where the
series of "et" are the residuals from a regression.
Use Durbin-Watson test to assess correlation between adjacent observations.
e = Property residual = (Original property
– Predicted property)
The Durbin-Watson test checks for sequential dependence in which each error (and also residual) is
correlated with those before and after it in the sequence."
Interpretation:

ranges from 0 (perfect positive correlation) to 4 (perfect negative correlation);

values from 1.5 (= du) to 2.5 (= 4-du) indicate no serious violation of independence ( for n >
30 )
0______1___ 1.5__ 2 __2.5 ___3______4
Results of dw:

0 <= dw <= 4 always;

the distribution of dw is symmetric about 2;

if successive residuals are positively serially correlated, that is positively correlated in their
sequence, dw will be near 0;

if successive residuals are negatively serially correlated, that is negatively correlated in their
sequence, dw will be near 4, so that (4 - dw) will be near 0.
Abbreviation:
dl = dw lower limit;
du = dw upper limit;
k = 1, considering 2 dimensional plots like regression plot;
Residuals from a fittet straigth line Y = b0 + b1* X;
n = number of C-Set resp. V-Set spectra;
alpha = 5% significance, typical;
Limits for dl and du after k, n, alpha are to find in reference:
[Savin, N.E. and White, K.J., "The Durbin-Watson Test for Serial Correlation with Extreme Sample
Sizes or Many Regressors", Econometrica, Vol. 45, 1977, pp. 1989-1996.]
Order : "The observations and residuals have a natural order."