Convergence of the power series – Texas Instruments TI-86 User Manual

Page 266

254

Chapter 19: Applications

19APPS.DOC TI-86, Chap 19, US English Bob Fedorisko Revised: 02/13/01 2:41 PM Printed: 02/13/01 3:05 PM Page 254 of 18

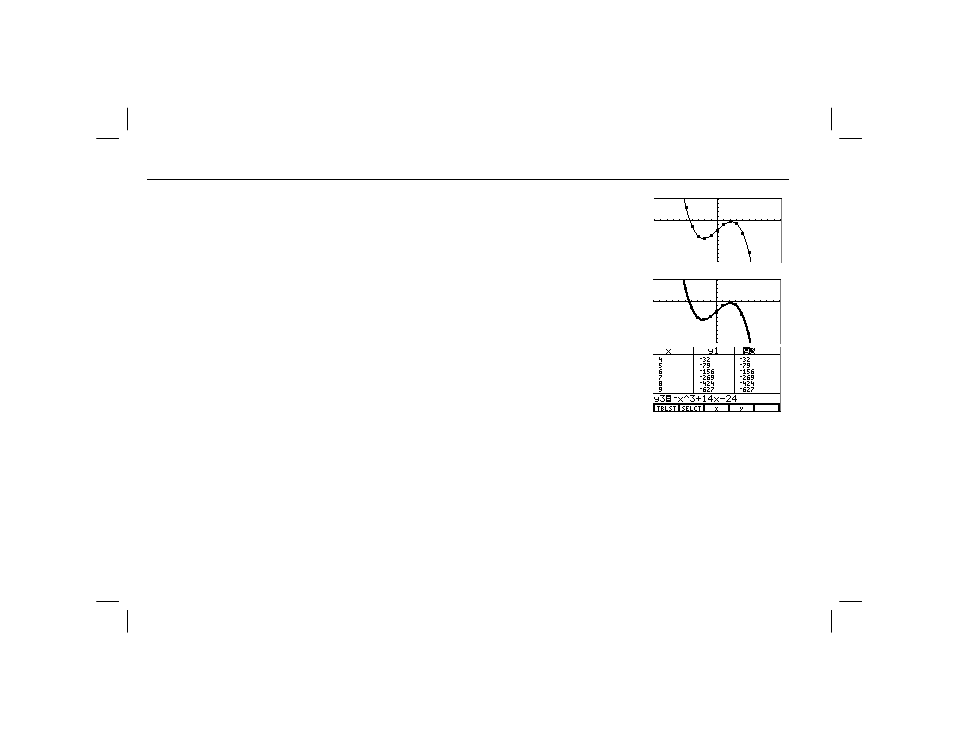
¸ Support this conjecture by graphing

(to which Cp(x) is

stored), and

Plot1

together.

¹ In the equation editor, enter the apparent characteristic

polynomial of matrix

and select ¼ (thick) graph style as shown.

y3=

x^3+14x

Ƹ Graph

, and

Plot1

ƹ Deselect

in the equation editor.

ƺ Select

TABLE

from the

TABLE

menu to display

and

in the

table.

Compare the values for the characteristic polynomial.

Convergence of the Power Series

A closed-form analytic antiderivative of (sin x)

àx does not exist. However, substituting t for

x, you can find an infinite series analytic solution by taking the series definition of sin t,
dividing each term of the series by t, and then integrating term by term to yield:

n+1

à((2n N1)(2n N1)!)

n=1

Plot finite approximations of this power series solution on the TI

-86 with

sum

and

seq