PASCO OS-8500 INTRODUCTORY OPTICS SYSTEM - Manual (Page 46)

Introductory Optics System 012-02744K
®
40
Crossed Slits
Examine the diffraction pattern formed by aperture H, the crossed slits. As you watch the
pattern, slowly rotate the Diffraction Plate so first one slit is vertical, then the other.
➀ Describe the diffraction pattern in terms of the patterns formed by each individual slit.
Random Array of Circular Apertures
Examine the diffraction pattern formed by aperture I, the random array of circular
apertures. The pattern is similar to that formed by diffraction through a single circular
aperture. To verify this, use a pin to poke a small hole in a piece of black construction
paper. Look at the Light Source filament through this hole. In the pattern formed by the
random array, the patterns from all the circular apertures are superposed, so the com-
bined diffraction pattern is brighter.
In the random array, smaller circles are used than you can produce with a pin.
➀ What effect does the smaller diameter of the circles have on the diffraction pattern?
In observing single slit diffraction, you found that the narrower the slit, the greater the
separation between the fringes in the diffraction pattern. This is generally true. For any
aperture, diffraction effects are most pronounced in a direction parallel with the smallest
dimension of the aperture.
➁ Use the above generalization to explain the symmetry of the diffraction pattern formed
by a circular aperture.
Square Array of Circular Apertures
Examine the diffraction pattern formed by aperture J, the square array of circular aper-
tures.
➀ How is this pattern similar to that formed by the random array? How is it different?
Each circular aperture in the array forms a circular diffraction pattern with maxima and
minima appearing at different radii. However, the regularity of the array causes there to
be interference between the patterns formed by the individual circles. This is analogous
to the way in which the double slit interference pattern creates maxima and minima that
are superimposed on the single slit patterns created by the individual slits.
➁ On a separate sheet of paper, draw the diffraction pattern you would expect if there were
no interference between the patterns from the different holes (as in the random array).
Clearly indicate the maxima and the minima.
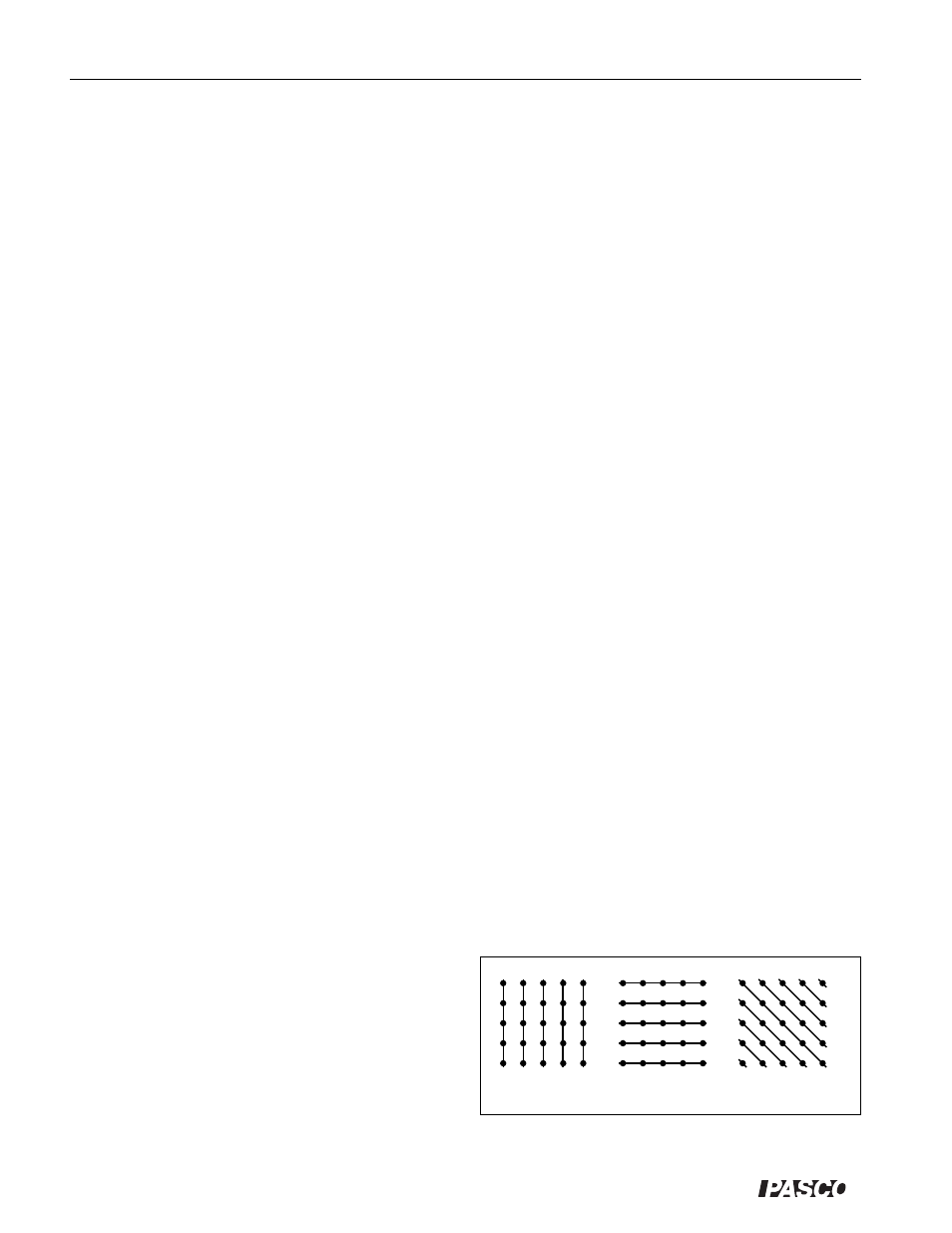
➂ To understand the interference that takes place, consider the array of points as if it were
actually a collection of parallel slits, such as those shown in Figures 17.2a, b, and c.
Draw the diffraction patterns that would be created by each of these collections of
parallel slits. Clearly label the maxima and
minima.
➃ Your drawing from step 2 shows where the light
is diffracted to from each individual circular
aperture. To approximate the effect of interfer-
ence between circular apertures, superimpose a
copy of one of your interference patterns from
step 3 over your drawing from step 2. Only
where maxima overlap, will there be maxima in
the combined pattern. Repeat this procedure for
each of your interference drawings.
Figure 17.2 Square Array Interference
(a) (b) (c)