PASCO OS-8500 INTRODUCTORY OPTICS SYSTEM - Manual (Page 30)

Introductory Optics System 012-02744K
®
24
Attach the Diffraction Plate to the other side of the Component Holder, as shown. Center pattern D, with the
slits vertical, in the aperture of the Slit Mask. Look through the slits. By centering your eye so that you look
through both the slits and the window of the Diffraction Plate, you should be able to see clearly both the
interference pattern and the illuminated scale on the Diffraction Scale.
➤ ➤ ➤ ➤ ➤NOTE: In this experiment, you look through the narrow slits at the light source, and the diffraction
pattern is formed directly on the retina of your eye. You then see this diffraction pattern superimposed
on your view of the illuminated diffraction scale. The geometry is therefore slightly more complicated
than it would be if the pattern were projected onto a screen, as in most textbook examples. (A very
strong light source, such as a laser, is required in order to project a sharp image of a diffraction pattern
onto a screen.)
The essential geometry of the experiment is shown in Figure 9.2. At the zeroth maxima, light rays from
slits A and B have traveled the same distance from the slits to your eye, so they are in phase and interfere
constructively on your retina. At the first order maxima (to the left of the viewer) light from slit B has
traveled one wavelength farther than light from slit A, so the rays are again in phase, and constructive
interference occurs at this position as well.
At the nth order maxima, the light from slit B has traveled n wavelengths farther than the light from slit
A, so again, constructive interference occurs. In the diagram, the line AC is constructed perpendicular to
the line PB. Since the slits are very close together (in the experiment, not the diagram), lines AP and BP
are nearly parallel. Therefore, to a very close approximation, AP = CP. This means that, for constructive
interference to occur at P, it must be true that BC = n
λ.
From right triangle ACB, it can be seen that BC = AB sin
θ, where A is the distance between the two slits
on the Diffraction Plate. Therefore, AB sin
θ = nλ. (The spacing between the slits, AB, is listed in the
Equipment section of this manual.) Therefore, you need only measure the value of
θ for a particular
value of n to determine the wavelength of light.
To measure
θ, notice that the dotted lines in the illustration show a projection of the interference pattern onto the
Diffraction Scale (as it appears when looking through the slits). Notice that
θ´ = arctan X/L. It can also be shown from the diagram that, if BP is parallel to AP as we have already
assumed, then
θ´ = θ. Therefore, θ = arctan X/L; and AB sin (arctan X/L) = nλ.
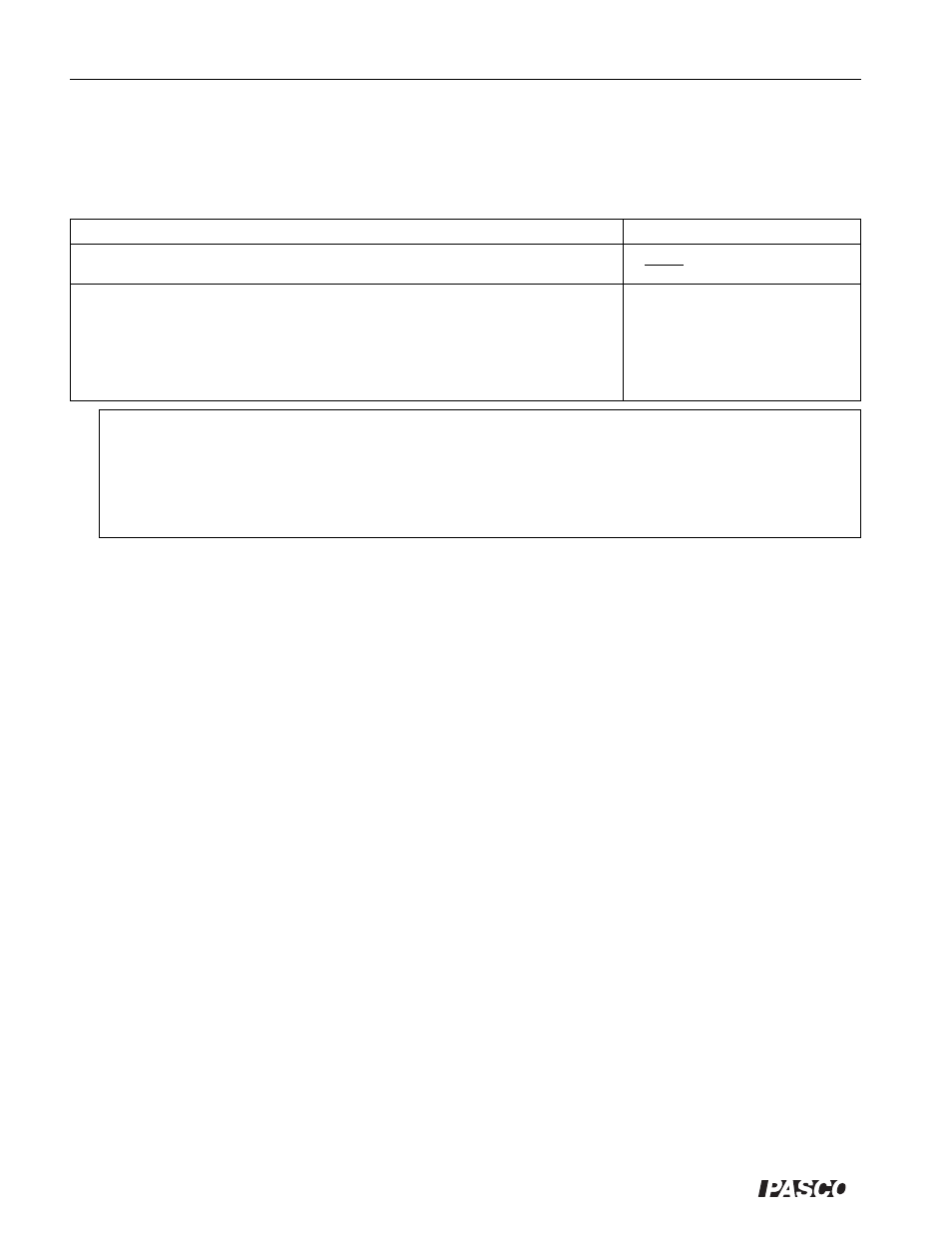
Looking through the pair of slits (pattern D) at the Light Source filament, make measurements to fill in Table
9.1. Alternately place the Red, Green, and Blue color filters over the Light Source aperture to make the meas-
urements for the different colors of light. If you have time, make measurements with the other two-slit patterns
as well (patterns E and F on the Diffraction Plate). Perform the calculations shown to determine the wavelength
of Red, Green, and Blue Light.
Additional Questions
➀ Assume, in the diagram showing the geometry of the experiment, that AP and BP are parallel.
Show that
θ = θ´.
➁ Suppose the space between the slits was smaller than the wavelength of light you were trying to measure.
How many orders of maxima would you expect to see?
AB
n
( )
split
spacing
( )
Data Calculations
Color n AB X L sin (arctan X/L) =
λ
Red
Green
Blue
Table 9.1 Data and Calculations