Guralp Systems CMG-3TB - Manual (Page 56)

CMG-3TB
Poles and zeroes
Most users of seismometers find it convenient to consider the sensor as
a “black box”, which produces an output signal
V from a measured
input x. So long as the relationship between V and x is known, the
details of the internal mechanics and electronics can be disregarded.
This relationship, given in terms of the Laplace variable s, takes the
form
( V / x ) (s) = G × A × H (s)
In this equation
•
G is the acceleration output sensitivity (gain constant) of the
instrument. This relates the actual output to the desired input
over the flat portion of the frequency response.
•
A is a constant which is evaluated so that A × H (s) is
dimensionless and has a value of 1 over the flat portion of the
frequency response. In practice, it is possible to design a system
transfer function with a very wide-range flat frequency
response.
The normalising constant A is calculated at a normalising
frequency value fm = 1 Hz, with s = j fm, where j = √–1.
•
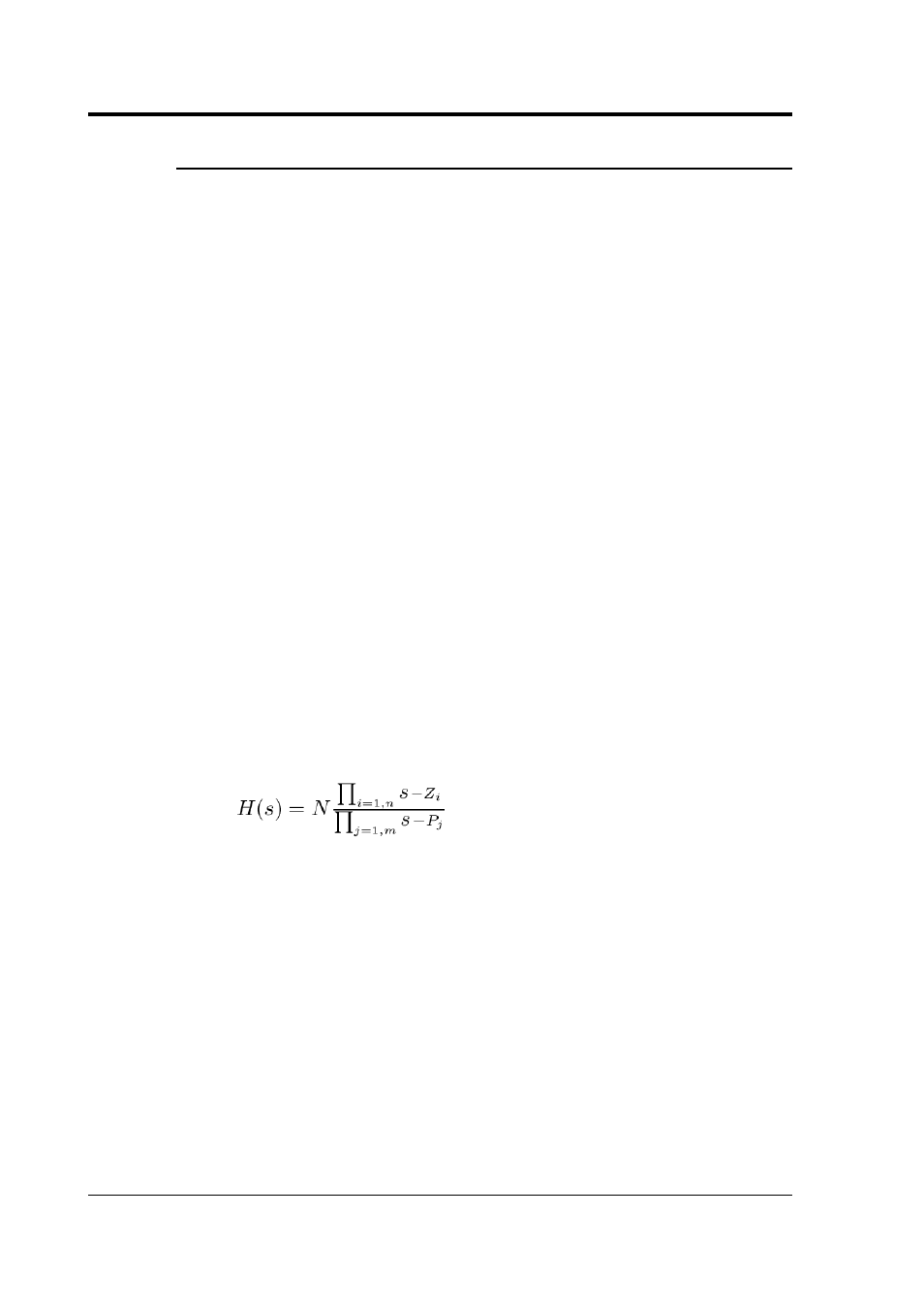
H (s) is the transfer function of the sensor, which can be
expressed in factored form:
In this equation z
n
are the roots of the numerator polynomial,
giving the zeros of the transfer function, and p
m
are the roots of
the denominator polynomial giving the poles of the transfer
function.
In the calibration pack, G is the sensitivity given for each component
on the first page, whilst the roots z
n
and p
m
, together with the
normalising factor A, are given in the Poles and Zeros table. The poles
and zeros given are measured directly at Güralp Systems' factory using
a spectrum analyser. Transfer functions for the vertical and horizontal
sensors may be provided separately.
56 Issue C