PASCO ME-9833 Physical Pendulum Set - Manual (Page 7)

Physical Pendulum Set Model No. ME-9833
7
®
Experiment 1: Parallel Axis Theorem
Equipment
Theory
The moment of inertia about a parallel axis, I
pivot
, is the moment of inertia about the center of
gravity, I
cm
, plus the moment of inertia of the entire object treated as a point mass at the center of
gravity:
where L is the perpendicular distance from the center of gravity (cog) to the pivot
point.
Procedure
Use the Rotary Motion Sensor to measure the angular acceleration of a rotating irregular shaped
object when it rotates about its center of gravity due to a net torque, and also when it rotates about a
parallel axis a distance “L” away from the center of gravity. Use the angular acceleration and the
known applied torque to determine the moment of inertia about the center of gravity and the
moment of inertia about the parallel axis.
Equipment Setup: Center of Gravity
1. Set up the Rotary Motion Sensor horizontally on a support rod so that the three step pulley is
on top. Start the DataStudio program on the computer and connect the sensor to the computer
interface.
2. Mount a Super Pulley with Clamp to the platform area at the end of the sensor opposite to
the support rod.
3. Connect a string to one of the steps on the three step pulley and drape the string over the
Super Pulley. Adjust the angle of the Super Pulley with Clamp so that the string is tangent
to the step on the three step pulley.
4. Use calipers to carefully measure the diameter of the step to which you attached the
string. Calculate the radius of the step and record it in the data table (Table 1).
5. Measure and record the mass, M, of the irregular shape object to be measured.
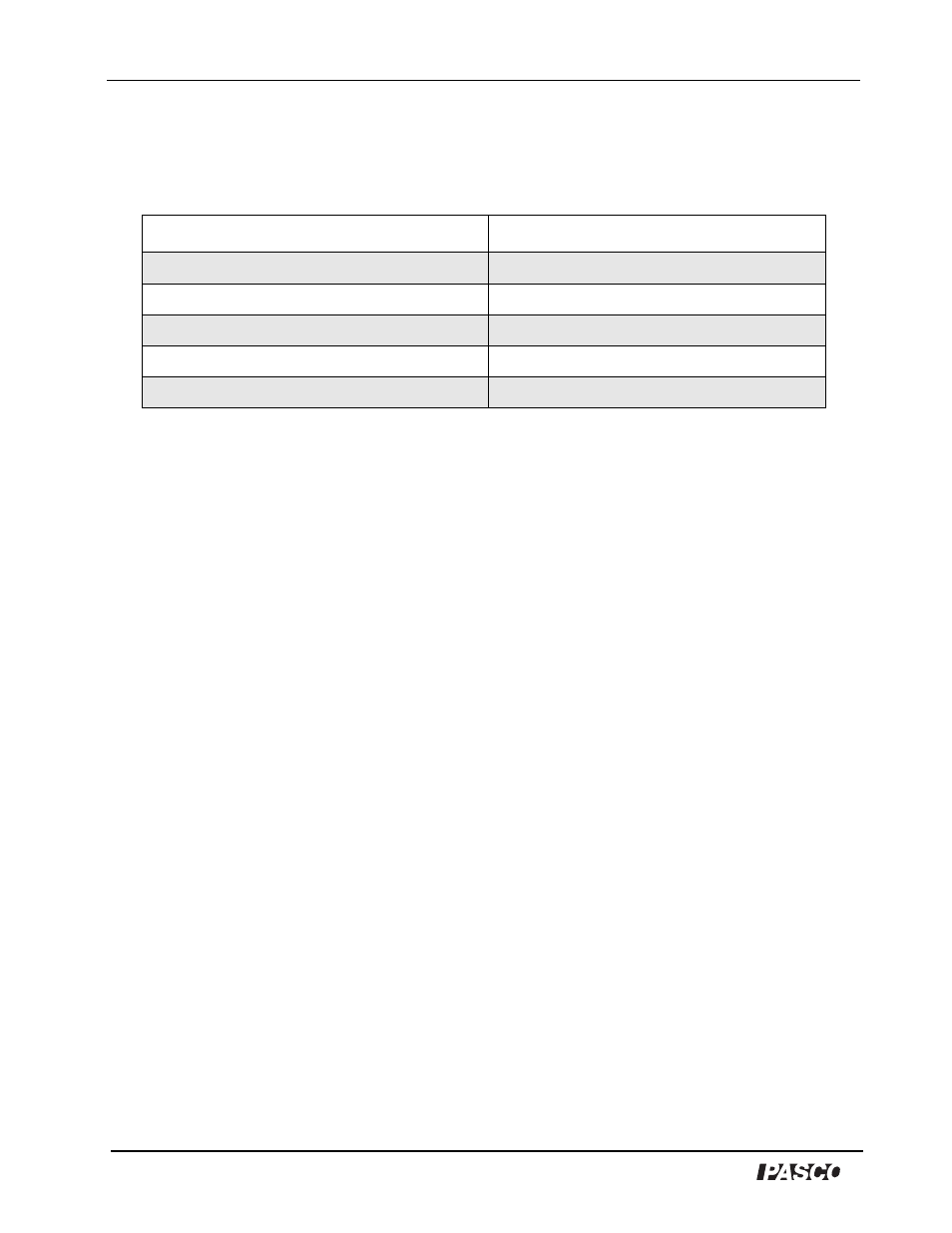
Item Item
PASCO Interface and DataStudio software Base and Support Rod
Rotary Motion Sensor String or thread
Irregular Shape from Physical Pendulum Set Mass and Hanger Set
Super Pulley with Clamp Vernier calipers
Balance
I
pivot
I
cm
ML
2
+ =