PASCO OS-8459 Beginning Optics System - Manual (Page 15)

®
M o d e l N o . O S - 8 4 5 9 E x p e r i m e n t 5 : T o t a l I n t e r n a l R e f l e c t i o n
15
Experiment 5: Total Internal Reflection
Purpose
In this experiment, you will determine the critical angle at which total internal reflec-
tion occurs in the acrylic rhombus and confirm your result using Snell’s Law.
Theory
For light crossing the boundary between two transpar-
ent materials, Snell’s Law states
n
1
sin
θ
1
= n
2
sin
θ
2
where
θ
1
is the angle of incidence,
θ
2
is the angle of
refraction, and n
1
and n
2
are the respective indices of
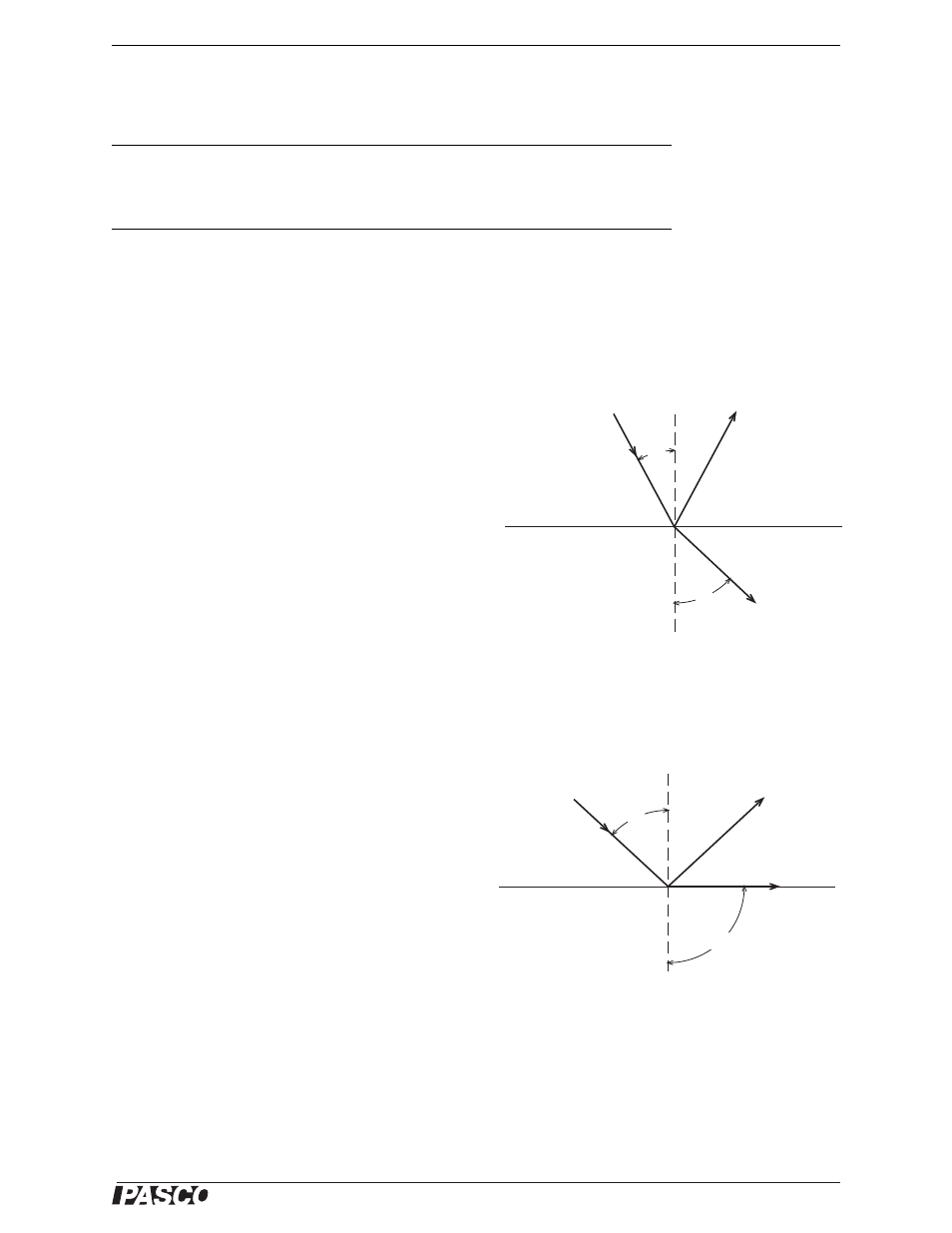
refraction of the materials (see Figure 5.1).
In this experiment, you will study a ray as it passes out
of the rhombus, from acrylic (n = 1.5) to air (n
air
= 1).
If the incident angle (
θ
1
) is greater than the critical
angle (
θ
c
), there is no refracted ray and total internal
reflection occurs. If
θ
1
=
θ
c
, the angle of the refracted
ray (
θ
2
) is 90°, as in Figure 5.2.
In this case, Snell’s Law states:
n sin
θ
c
= 1 sin 90°
Solving for the sine of critical angle gives:
Required Equipment from Beginning Optics System
Light Source
Rhombus from Ray Optics Kit
Other Required Equipment
Protractor
White paper
Surface
Refracted ray
(n
1
> n
2
)
Incident ray
n
1
n
2
q
1
q
2
Reflected ray
Figure 5.1
Refracted ray
Incident ray
n
n
air
= 1
q
c
Reflected ray
90°
Figure 5.2
sin
θ
c
1
n
--- =