9 arithmetic algorithms in the 899 coulometer – Metrohm 899 Coulometer User Manual
Page 113
■■■■■■■■■■■■■■■■■■■■■■
10 Appendix
899 Coulometer
■■■■■■■■
103
Acknowledgement of
the instrument
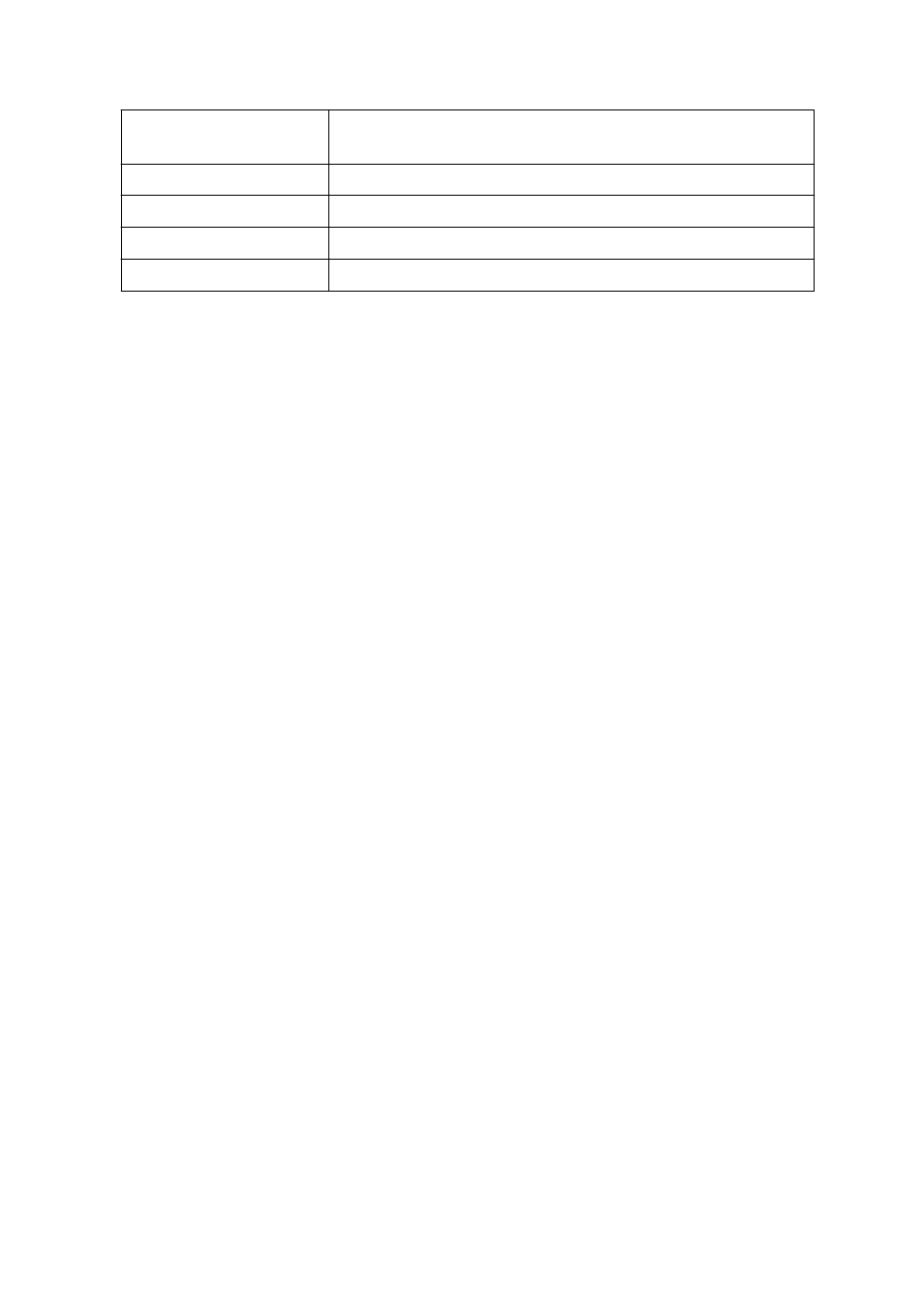
Comment
OK
Command executed
E1
Method not found
E2
Invalid variable
E3
Invalid command
10.9
Arithmetic algorithms in the 899 Coulometer
Numerical format
The software of the 899 Coulometer calculates in accordance with the
widespread standard IEEE 754 (IEEE Standard for Binary Floating-Point
Arithmetic for Microprocessor Systems). This means that the numbers are
used in calculations in "double precision" (64 bit). Decimal numbers are
converted into binary numbers in the computer and used in this form for
calculations. The output on the display and in reports once again contains
decimal numbers; the binary numbers are thus converted back into deci-
mal numbers. In order to be able to check the internal calculations per-
formed by the computer yourself in accordance with IEEE 754, the num-
bers are reproduced in the calculation report in complete accuracy. A min-
imal difference may arise between an originally entered decimal number
and the internal computer representation in complete accuracy in the
range of the rear decimal places. This difference results from the fact that
an exact binary equivalent does not exist for every decimal number. If, for
example, you enter the sample size 50.3 mg, this will be depicted in the
calculation report in "double precision" with 5.02999999999999E+01.
Rounding-off process
Measured values and results are rounded to the defined number of deci-
mal places (commercial rounding, in accordance with the US Pharmaco-
peia USP). If the digit at the first dropped decimal place is 1, 2, 3 or 4,
then it will be rounded off; if this digit is 5, 6, 7, 8 or 9, then it will be
rounded up. Negative digits will be rounded in accordance with their
amount, i.e. away from zero.
Examples:
2.33 yields 2.3
2.35 yields 2.4
2.47 yields 2.5
–2.38 yields –2.4
–2.45 yields –2.5
