Campbell Scientific 4WFBS120, 4WFBS350, 4WFBS1K 4 Wire Full Bridge Terminal Input Modules - Manual (Page 28)

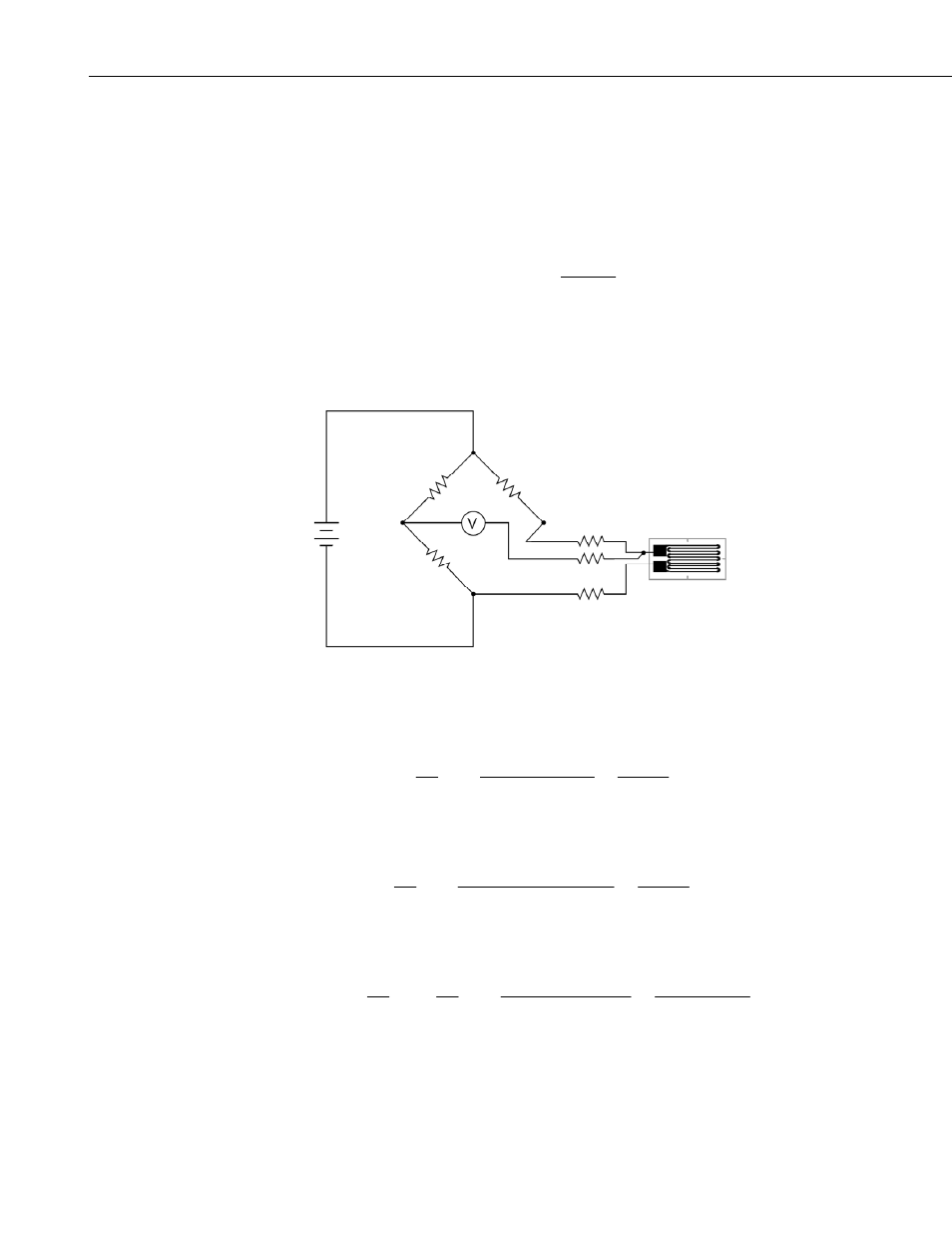
4WFBS120, 4WFBS350, 4WFBS1K 4 Wire Full Bridge Terminal Input Modules (TIM)
4.4.1.1 Mathematical Lead Compensation Circuit and Equations
If the lead resistance is known, the sensitivity error can be mathematically
corrected for by multiplying the output by a simple factor (1+R
L
/R
G
) where R
L
is the nominal resistance of one of the lead legs and R
G
is the resistance of the
strain gauge. The Gauge Factor can be multiplied by the inverse of this value,
R
G
/(R
G
+ R
L
), to derive an adjusted Gauge Factor.
⎟
⎟
⎞
⎜
⎜
⎛
× = g
raw adj
R
GF GF
⎠ ⎝
+
L g
R R
4.4.1
The adjusted Gauge Factor, GF
adj
, would be used in the StrainCalc function to
derive the µ Strain. The proof used to derive this adjusted Gauge Factor is
shown below:
R
2
= 1K Ω
R
1
= 1K Ω
R
D
R
L
R
L
R
4
=Gauge
Excite
+ -
R
L
FIGURE 4.4-1. Three wire ¼ bridge strain circuit
Balanced Bridge Condition
2 1
1
L D L G
L G
BAL I
O
R R
R
R R R R
R R
E
E
+
−
+ + +
+
=
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
4.4.2
Strained Bridge Condition
2 1
1
G L D L G
G L G
STR I
O
R R
R
R R R R R
R R R
E
E
+
−
Δ + + + +
Δ + +
=
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
4.4.3
Change in Bridge Output (V
R
)
L G D
L G
G G L D
G L G
BAL I
O
STR I
O
R 2R R R
R R
R R 2R R
R R R
E
E
E
E
V
+ +
+
−
Δ + + +
Δ + +
=
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
−
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
=
4.4.4
22