2 trapezoidal weir (cipolletti), Selection of the actual flow (q), Calculation of the actual flow value (q) – VEGA VEGASON 71 - D User Manual
Page 27
VEGASON 71 - D
27
7 Output results
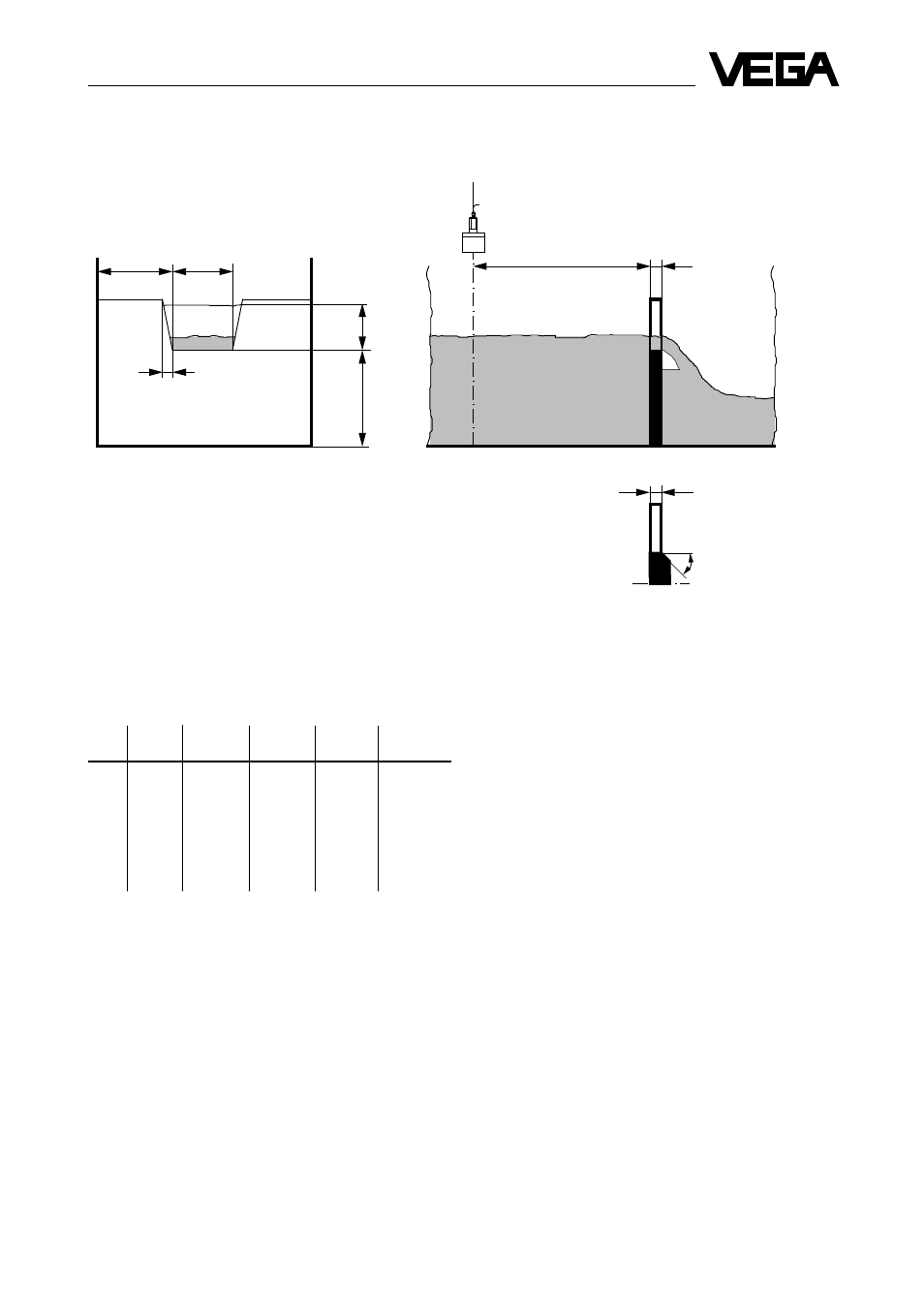
7.6.2 Trapezoidal weir (Cipolletti)
With sharp weir edge (Cipolletti)
3 - 4 x h
max
≥
2 x h
max
Explanation:
Q
max
= max. flow in m
3
/h
B
= weir width (bottom) in mm or m
K
= weir specific factor
h
max
= max. weir height in mm or m
k
= thickness of the weir in mm
B
45
°
k
≈
3 mm
Selection of the actual flow (Q)
Flow schedule for usual trapezoidal weir
B
K
Q
max
h
max
Q
min
h
min
in mm (Factor)
in m
3
/h
in mm
in m
3
/h
in mm
300
2011
116,8
150
29,5
60
450
3014
354,4
240
44,3
60
600
4011
760,4
330
58,9
60
800
5353
1457,0
420
78,7
60
1000
6688
2581,0
530
93,3
60
1500
10043
6523,0
750
147,6
60
2000
13381
14397,0
1050
196,7
60
3000
20082
36893,0
1500
295,1
60
If a trapezoidal weir acc. to above value is used, the value
for Q
max
can be taken out of the respective column and can
be used for programming of mode
0 - 82
…
0 - 84
.
Programming acc. to selection Q
max
= 36893 m
3
/h
-
Mode
0 - 82
, flow = 3689
-
Mode
0 - 83
, multiplier = 1
-
Mode
0 - 84
, time unit = 3 (h)
Calculation of the actual flow value (Q)
The following formula can be used for calculation of the
actual flow for liquid levels < h
max
… > h
min
.
Q = K • h
1,5
Q in m
3
/h
B in m
h in m
Assumed values for a calculation example
B =
0,6 m
K =
4011 (derived from B)
h =
0,27 m
Calculation example
Q =
4011• 0,27
1,5
= 562,73 m
3
/h
Programming acc. to calculation example
Q
max
= 562,73 m
3
/h
- Mode
0 - 82
, flow = 0563
- Mode
0 - 83
, multiplier = 0
- Mode
0 - 84
, time unit = 3 (h)
Weir
K (Factor)
k
≈
3 mm
1
/
4
h
max
h
max
2 - 3 x h
max
or
