HP SmartCalc 300s - Manual (Page 20)

■ Sum sub-menu(sums),Var sub-menu(number of
samples,mean, standard deviatian),and MinMax
sub-menu(maximum value,minmum value)
operations are the same those for linear regression
calculations.
Quadratic Regression Calculation:
• For examples:
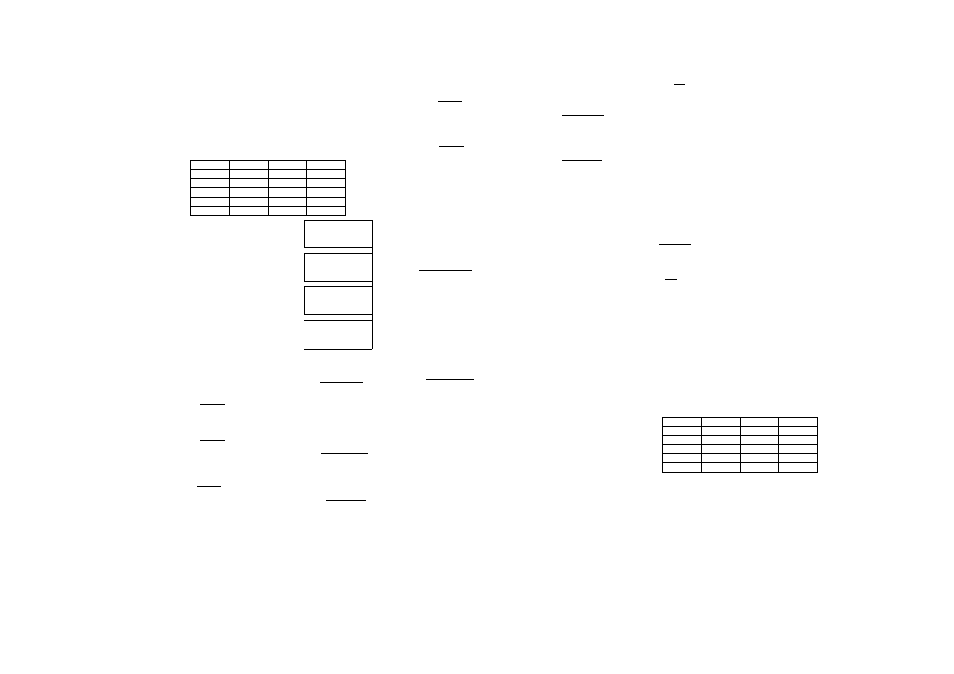
All the data be used as follow table:
X y X y
1.0 1.0 2.1 1.5
1.2 1.1 2.4 1.6
1.5 1.2 2.5 1.7
1.6 1.3 2 . 7 1.8
1.9 1.4 3.0 2.0
sK_+cx^:
1:1-VAR, 2:A-rBX
3: -rCX^ 4:lnX ^
5:^'X^ 6:A-B^X
7:A-X^B 8:1/X
STAT
1 ^ 1
' 1 1
2 1.2
3 1.5 i:2 1
STAT B
0
1:A
3:C
55i2 2:B
4:5^1
6:y
0.7028598638
ISHIFT im(STAT) H(Reg) B
E](B)S 0.2576384379
STAT B
ISHIFT im(STAT) H(Reg) C
)H 0.05610274153
y=3^-xi=?
_____ I sta T
rslISHiFT im(STAT) 0(Reg) 3ih
3](xi)H
4.502211457
y= 3-^X2=?
______ I STAT B I
i3llSHIFT imfSTAT) [7](Reg) 3ib
S](^)S -9.094472563
x=2-^y=?
STAT B I
rSIISHIFT im(STAT) [7](Reg) 2 y
y)S 1.442547706
Comments for Other Types of Regression
For details about the calculation formula of the
command included in each regression type, refer to
the indicated calculation formulas
For example:
Logarithmic Regression (In X)
y=A+BlnX
A Ey-B-EInx
75— n
p n-Eilnxly-EInxEy
“ n-E(lnx)2-(Elnx)5
V — n-E(lnx)y^ElnxEy
7{n-E(lnxy-(Elnx)2}{n-E)7-(Eyn
A -fci
x=e ®
y-A+Blnx
e Exponential Regression (e" X)
y=Ae“
A=exp(5iny_B^^
D _ xl n y-E x*£ I ny
n£x2-(Ex)2
n*Exlny-ExElny
V{n-Ex2-(i:x)2^{n-i:(|ny)2-(Elny)2}
A lny-ln>A
^=Ae^
ab Exponential Regression (A ■ B~X)
y=AB"
A= ex
B=exp(TSl|n^|^)
nExIny-ExElny
7{n-Ex2-(Ex)2}{n-E(lny)2-{Slny)2}
-----------------------------------SS----------------------------------- '
_lny-
In
InA
y=AET
Power Regression(A • X B)
y^AX'
A=exp(51yc551iit^
D nEinxInv-Einx'Elnv
n.E(lnxP-(Elnx)2
n-EinxIny-EinxElny
y{n-E(lnx)2(Elnx}^{n.E(lny)2-(Elny)2}
. Iny—InA
x=e^
^=Ax‘
Inverse Regression (1/X)
y=A4
A Tly-BEx’
f- Sxy
VSxxSyy
Sxx=E(x'p-
(Ex')2
Syy=Ey2-%i'
Sxy=E(x')y-^4^
6__ B_
y-A
9=A+i
Comparison Regression Curves
The following example uses the data input in as
following table:
X y X y
1.0 1.0 2.1 1.5
1.2 1.1 2.4 1.6
1.5 1.2 2.5 1.7
1.6 1.3 2.7 1.8
1.9 1.4 3.0 2.0
Compare the correlation coefficient for logarithmic,
e exponential,
ab exponential. Power, and inverse
regression.
57