Rainbow Electronics MAX8514 - Manual (Page 26)

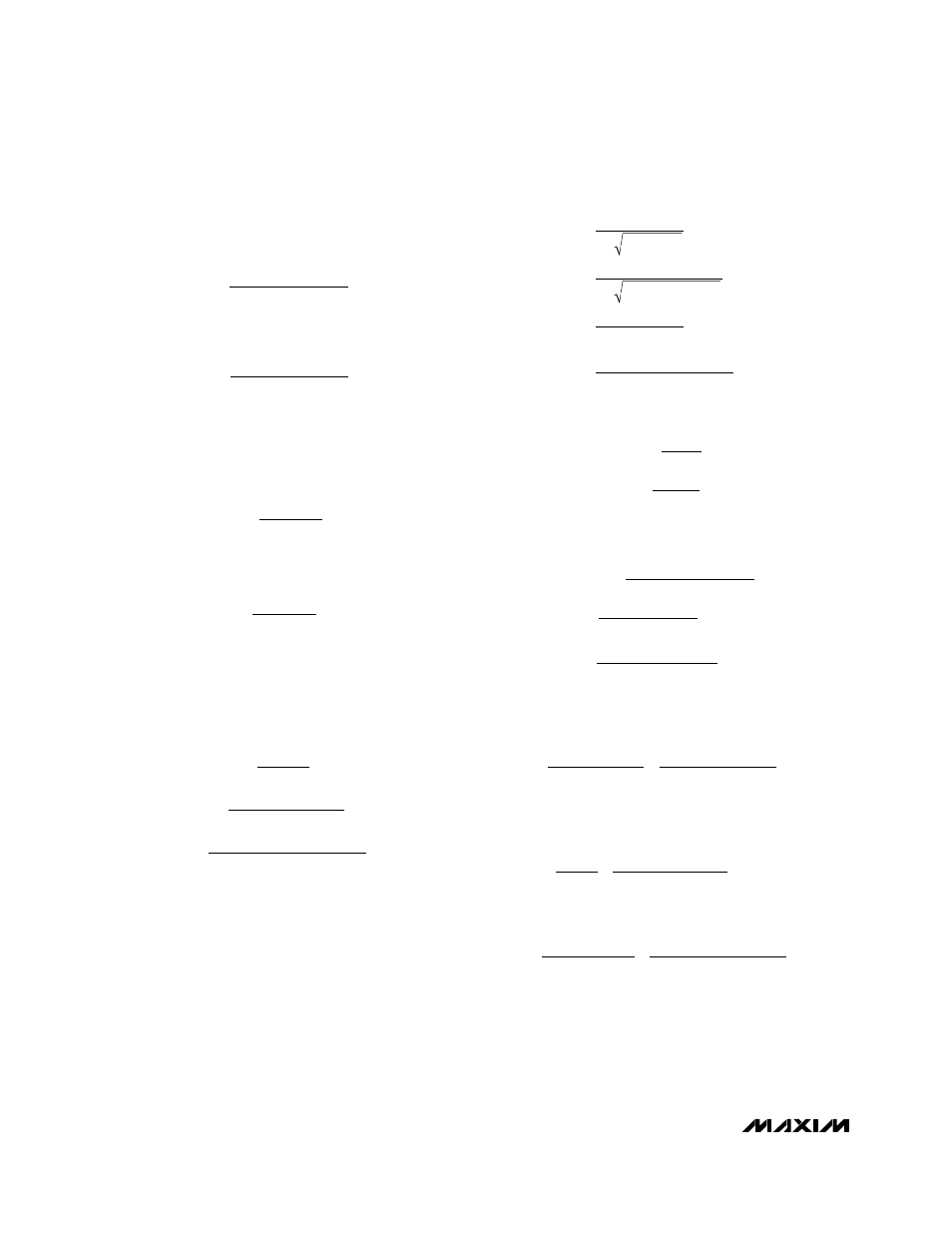
MAX8513/MAX8514
Wide-Input, High-Frequency, Triple-Output Supplies
with Voltage Monitor and Power-On Reset
26 ______________________________________________________________________________________
This gain between f Z1 and f Z2 is also set by the ratio of
R3/R1, where R1 is selected in the OUT1 Voltage
Setting section. Therefore:
And similar to Case 1, C5 can be calculated as:
Set the error-amplifier third pole, f P3 , at approximately
1/2 the switching frequency. The gain of the error
amplifier at f
C
(between f
P2
and f
P3
) is set by the ratio
of R3/R I and is also equal to:
where R I is:
Therefore:
Similar to Case 1, R4, C11, and C12 can be calculated as:
Below is a numerical example to calculate the error-
amplifier compensation values for Case 2:
V IN = 12V (nomimal input voltage)
V
RAMP
= 1V
V OUT1 = 3.3V
V FB1 = 1.25V
L1A = 6.2µH
C4 = 560µF/ 10V OS-Con capacitor, with ESR = 0.015Ω
f
S = 300kHz
Pick R2 = 8.06kΩ. Then:
Pick f C = 50kHz, which is less than f S / 5.
Use 20kΩ.
Use 12nF.
Use 2.2kΩ.
Use 3.9nF.
Pick f
P
3 = f
S
/ 2 = 150kHz.
C
R f k kHz
nF
ZESR
11
1
2 4
1
2 2 2 18 95
3 82
. .
. =
Ч Ч
=
Ч Ч
=
π π Ω
R R G k k
R
R R
R R
k k
k k
k
I MOD fc
I
I
. .
. .
. .
.
( )
= Ч = Ч =
=
Ч
=
Ч
=
3 20 0 0923 1 846
4
1 13 3 1 846
13 3 1 846
2 14
Ω Ω
Ω Ω
Ω Ω
Ω
1- -
C
R f k kHz
nF
PMOD
5
2
3
2
20 2 7
11 8
.
. =
Ч Ч
=
Ч Ч
=
π π Ω
G
kHz
kHz kHz
G
f
f G
kHz
kHz
R R G k k
MOD DC
EA fZ fZ
PMOD
ZESR MOD fc
EA fZ fZ
( )
( )
( )
( )
.
.
.
.
. .
.
. . .
= Ч
Ч
=
=
=
Ч
=
= Ч = Ч =
−
12
2 7
18 95 50
0 0923
2 7
18 95 0 0923
1 543
3 1 13 3 1 543 20 48
2
1 2
1 2 -
Ω Ω
R k
V
V
k
G
V
V
MOD DC
IN
RAMP
1 8 06
3 3
1 25
1 13 3
12
.
.
.
.
( )
= × =
= =
Ω Ω -
f
L A C
H F
kHz
f
R C
F
kHz
PMOD
ZESR
ESR
=
Ч
=
Ч
=
=
Ч
=
Ч Ч
=
.
.
.
.
1
2 1 4
1
2 6 2 560
2 7
1
2 4
1
2 0 015 560
18 95
π
π µ µ
π
π µ Ω
R
R R
R R
C
R f
C
C
C R f
I
I
ZESR
P
4
1
1
11
1
2 4
12
5
2 5 3 1
3
=
Ч
=
Ч Ч
=
Ч Ч Ч
-
-
π
π
R R G G
I MOD fc D ( )
= Ч Ч 3
R
R R
R R
I
=
×
+
1 4
1 4
G
G
EA fc
MOD fc
( )
( )
=
1
C
R f
PMOD
5
2
3
=
Ч Ч π
R
R f
f G
PMOD
ZESR MOD fc
3
1
( )
=
Ч
Ч