Rainbow Electronics MAX8514 User Manual
Page 24
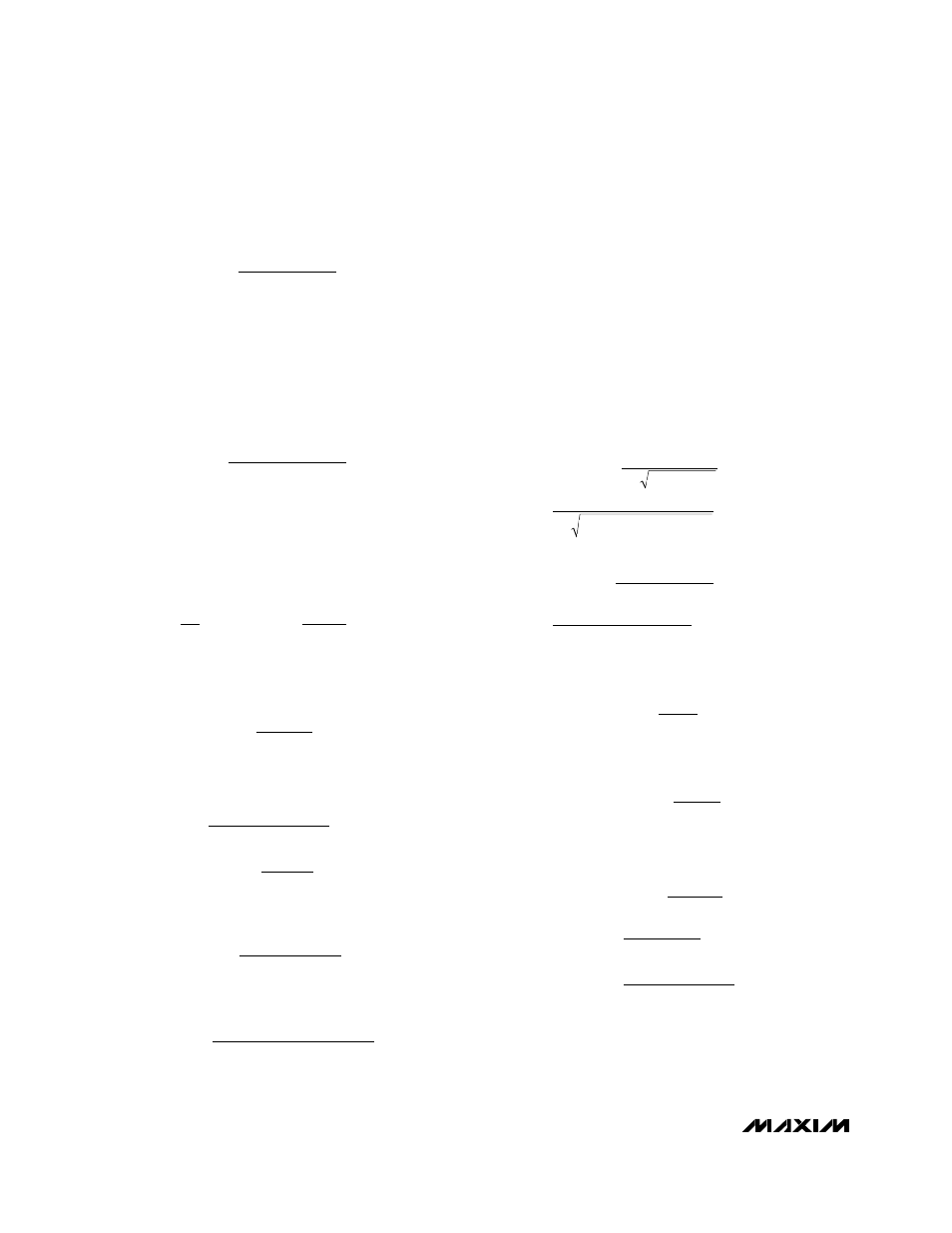
MAX8513/MAX8514
Wide-Input, High-Frequency, Triple-Output Supplies
with Voltage Monitor and Power-On Reset
24
______________________________________________________________________________________
This gain is also set by the ratio of R3/R1 where R1 is
calculated in the OUT1 Voltage Setting section. Thus:
Due to the underdamped (Q > 1) nature of the output
LC double pole, the error-amplifier zero frequencies
must be set less than the LC double-pole frequency to
provide adequate phase boost. Set the error-amplifier
first zero, f
Z1
, at 1/4th the LC double-pole frequency and
the second zero, f
Z2
, at the LC double-pole frequency.
Hence:
Set the error-amplifier f
P2
at f
ZESR
, and f
P3
to 1/2 the
switching frequency, if f
ZESR
< 1/2 f
S
. If f
ZESR
> 1/2 f
S
,
then set f
P2
at 1/2 f
S
and f
P3
at f
ZESR
.
The gain of the error amplifier between f
P2
and f
P3
is
set by the ratio of R3/R
I
and is equal to:
where R
I
is the parallel combination of R1 and R4 and
is equal to:
Therefore:
C11 can then be calculated as:
and C12 as:
Below is a numerical example to calculate the error-
amplifier compensation values used in the Typical
Applications Circuit of Figure 5:
V
IN
= 12V (nomimal input voltage)
V
RAMP
= 1V
V
OUT1
= 3.3V
V
FB1
= 1.25V
L1A = 1.8µH
C4 = 47µF/ 6.3V ceramic, with R
ESR
= 0.008Ω
f
S
= 1.4MHz
The LC double-pole frequency is calculated as:
Pick R2 = 8.06kΩ.
The modulator gain at DC is:
Pick f
C
= 100kHz.
G
kHz
kHz
G
f
f G
kHz
kHz
R
R
G
k
k
MOD fc
EA fZ
fZ
PMOD
C MOD fC
EA fZ
fZ
( )
(
)
(
)
(
)
.
.
.
.
.
.
.
.
=
Ч
=
=
=
Ч
=
=
Ч
=
Ч
=
−
−
12
17 4
100
0 363
17 4
100
0 363
0 479
3
1
13 3
0 479
6 37
2
1
2
1
2
Ω
Ω
G
V
V
MOD DC
IN
RAMP
(
)
=
= 12
R
k
V
V
k
1
8 06
3 3
1 25
1
13 3
.
.
.
.
=
×
=
Ω
Ω
-
f
L A
C
kHz
f
R
C
kHz
PMOD
ZESR
ESR
.
.
.
=
Ч
=
Ч
Ч
Ч
=
=
Ч
Ч
=
Ч
Ч
Ч
=
1
2
1
4
1
2
1 8 10
47 10
17 3
1
2
4
1
2
0 008 47 10
423
6
6
6
π
π
π
π
-
-
-
C
C
C
R
f
P
12
5
2
5
3
3
=
Ч
Ч
Ч
(
)
π
-1
C
R
f
P
11
1
2
4
2
=
Ч
Ч
π
R
R
f
f
G
and
R
R
R
R
R
I
PMOD
P
EA fZ fZ
I
I
(
)
=
Ч
Ч
=
Ч
3
4
1
1
2
1
2
-
-
R
R
R
R
R
I
=
×
+
1
4
1
4
R
R
G
f
f
I
EA fZ fZ
P
PMOD
3
1
2
2
(
)
=
-
C
R
f
PMOD
5
2
3
=
Ч
Ч
π
R
R
f
f
G
Z
C
MOD fc
3
1
2
( )
=
Ч
Ч
