PASCO ME-9891 Flexible I-beam - Manual (Page 4)

®
F l e x i b l e I - b e a m T h e or y
4
Moment Functions
To predict the deflection of a beam, it is first necessary to express the internal moment
M as a function of x along the entire length of the beam. In some cases, the beam must
be divided into regions with a different function for each region.
A detailed description of this analysis can be found in most structural analysis text
books. Here, we will simply give the solutions for two examples relevant to the exper-
iment below.
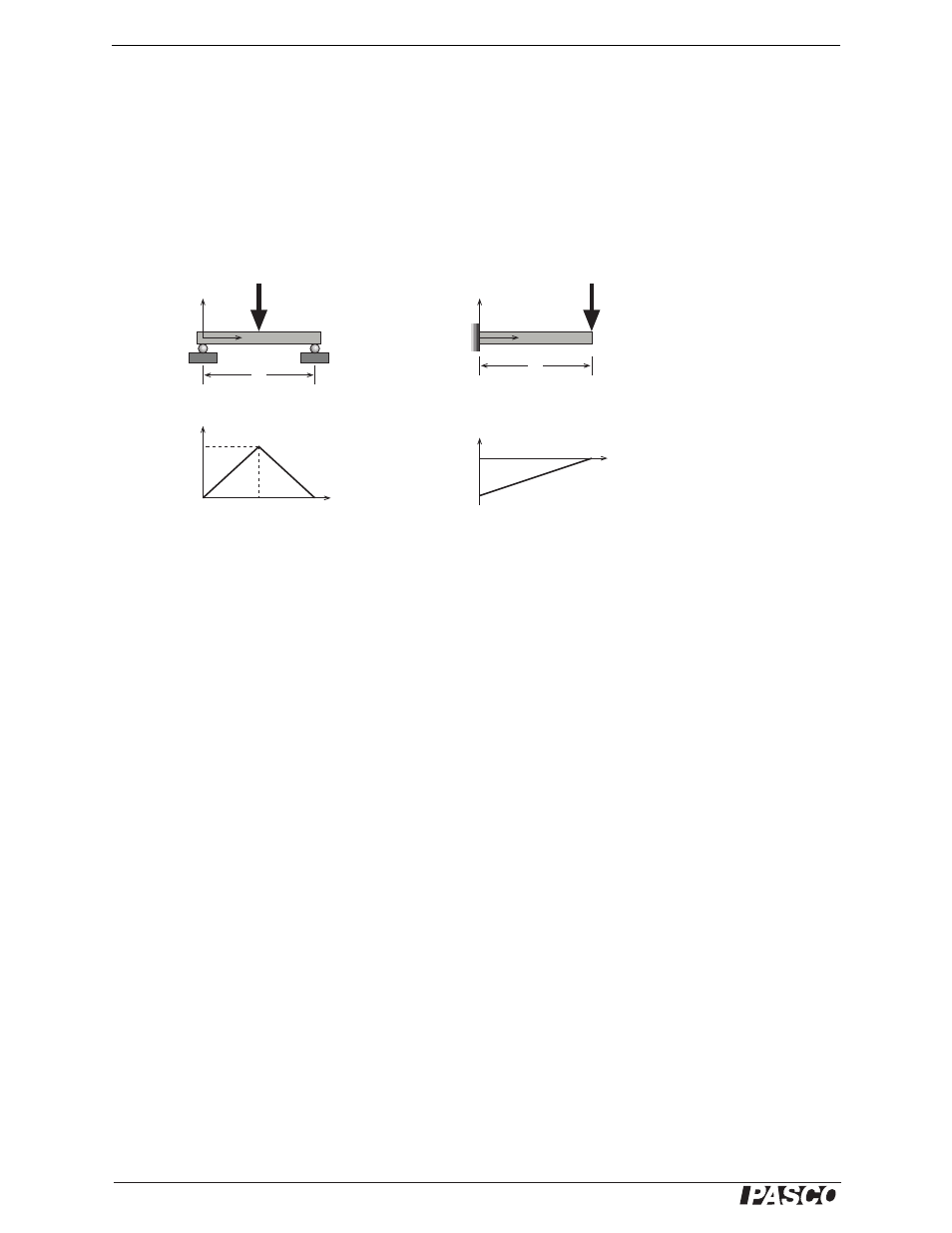
Figure 6
The beam in Figure 6a is supported on two rollers with the load applied in the center.
Each support applies an upward force equal to F/2. In the left half of the beam, the
internal moment is
for . The internal moment in the right
half is .
The cantilevered beam in Figure 6b is supported by a single fixed support. To counter
the load, the support applies an upward force equal to F, and a counter-clockwise
moment of Fx. The internal moment (for any value of x along the length of the beam)
is M = F(x - L).
F
L
y
x
(b)
M
x
-FL
L
y
L
x
F
(a)
M
x
FL/4
L L/2
M
1
Fx 2 ⁄ = 0 x L 2 ⁄ ≤ ≤
M
2
F x L –
( ) 2 ⁄
– =