Vernier Spectro Pro User Manual
Page 47
Spectro Pro Reference
Spectro Pro
8
(
)
a
x
y
x
x y
i
i
i
i
i
=
−
∑
∑
∑
∑
1
2
∆
(
)
b
N
x y
x
y
i
i
i
i
=
−
∑
∑
∑
1
∆
where
( )
∆ =
−
∑
∑
N
x
x
i
i
2
2
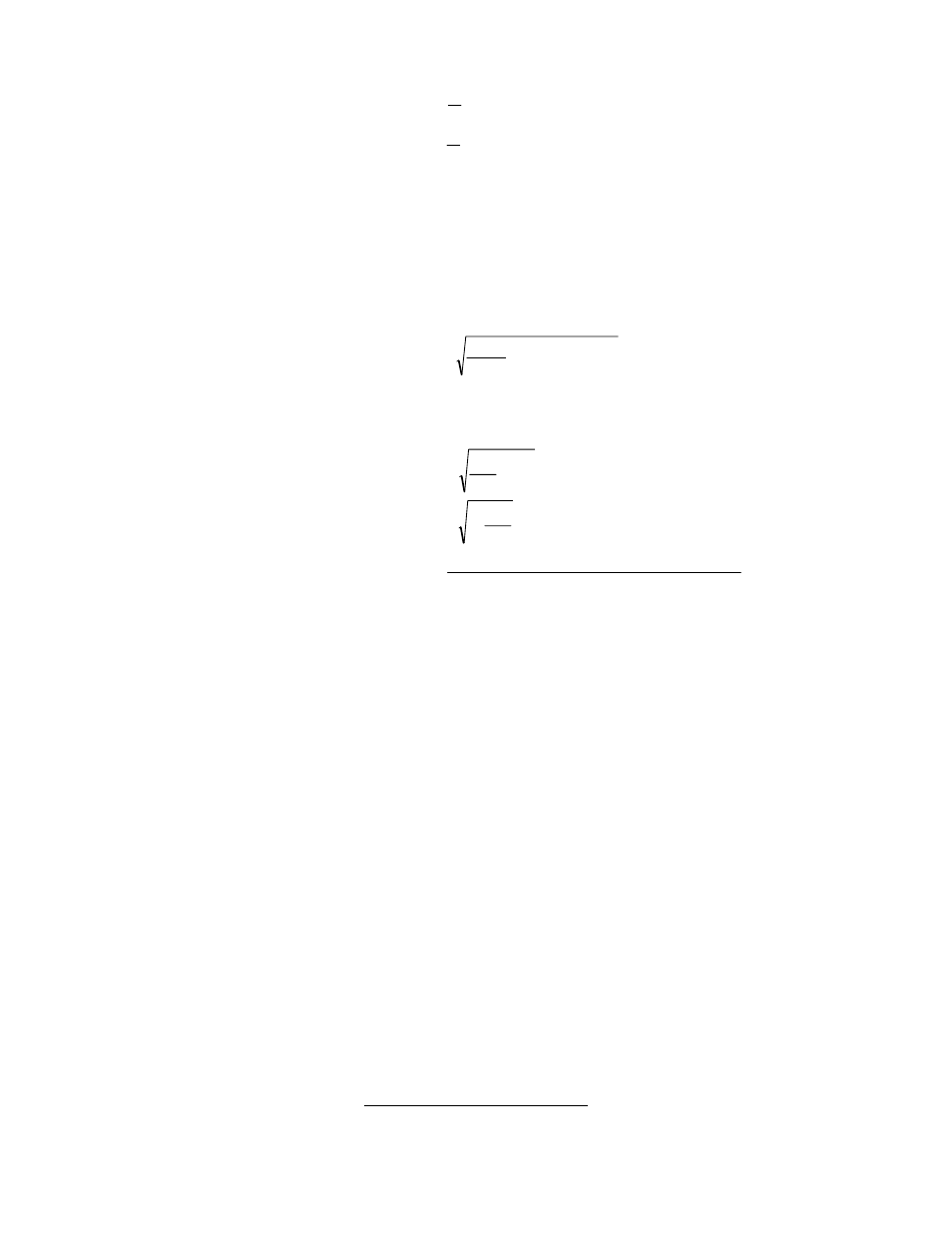
Measures of the goodness of fit are many. Most common are the scatter
standard deviation,
σ
s
, the linear correlation coefficient r, and the
uncertainties of the parameters a and b,
σ
a
and
σ
b
. The scatter standard
deviation measures how far away, on average, the data points y
i
fall from
the fitted line, measured along a vertical line.
1
σ
s
is also called the root
mean square error, and is defined as
(
)
σ
s
i
i
N
y
a
bx
=
−
− −
∑
1
2
2
.
We use N - 2 weighting since two parameters have been determined in
the curve fit.
The remaining quantities are defined as
σ
σ
a
s
i
x
=
∑
2
2
∆
σ
σ
b
s
N
=
2
∆
( )
( )
r
N
x y
x
y
N
x
x
N
y
y
i
i
i
i
i
i
i
i
=
−
−
−
∑
∑
∑
∑
∑
∑
∑
2
2
1 2
2
2
1 2
/
/
The first two quantities are interpreted as the variance of the fitted
parameters, and so can be used as 67% confidence level uncertainties of
the slope and intercept.
The correlation coefficient, r, is commonly calculated by scientific
calculators, but is a difficult quantity to interpret. The correlation
coefficient is intended to measure the degree of correlation between the
x and y values. It is not directly a measure of goodness of fit. For no
correlation at all (random values), r is near zero. For perfect correlation r
is
±
1. From r one can determine a probability that the x and y values are
correlated. In the natural sciences, however, there is usually the
assumption that the two are correlated, and so the r value is not very
useful. Far more useful to a student or scientist is the uncertainty of the
slope and intercept. These uncertainties answer the question “How well
did the data determine a slope (or intercept)?”.
No provision has been given to weighted fits, since in computer-
acquired data all data points are generally equally reliable.
More information on curve fitting and the interpretation of the fitted
parameters can be found in Data Reduction and Error Analysis for the
Physical Science, 2
nd
edition, Philip R. Bevington and D. Keith
Robinson, McGraw-Hill, Inc., 1992.
The automatic curve fit function can be disabled in the Options, found in
the Experiments menu.
Spectro Pro will superimpose a function (sometimes called a model)
over your data using the Manual Curve Fit option. In contrast to the
1
The least squares fitting method assumes that the uncertainties in the x values
are negligible compared to the uncertainties of y.
Manual Curve Fit
