Rainbow Electronics MAX1587A - Manual (Page 25)

MAX1586A/MAX1586B/MAX1587A
High-Efficiency, Low-I
Q
PMICs with
Dynamic Core for PDAs and Smart Phones
______________________________________________________________________________________ 25
must also have low impedance at the switching fre-
quency. Ceramic, polymer, and tantalum capacitors
are suitable, with ceramic exhibiting the lowest ESR
and lowest high-frequency impedance.
Output ripple with a ceramic output capacitor is
approximately:
V RIPPLE = I L(PEAK) [1 / (2π x f OSC x C OUT )]
If the capacitor has significant ESR, the output ripple
component due to capacitor ESR is:
V RIPPLE(ESR) = I L(PEAK) x ESR
Output capacitor specifics are also discussed in the
Compensation and Stability section.
Compensation and Stability
The relevant characteristics for REG1, REG2, and
REG3 compensation are:
1) Transconductance (from FB_ to CC_), gm EA
2) Current-sense amplifier transresistance, R CS
3) Feedback regulation voltage, V FB (1.25V)
4) Step-down output voltage, V OUT , in V
5) Output load equivalent resistance, R LOAD = V OUT /
I LOAD
The key steps for step-down compensation are:
1) Set the compensation RC zero to cancel the R
LOAD
C OUT pole.
2) Set the loop crossover at or below approximately
1/10th the switching frequency.
For example, with V
IN(MAX)
= 5V, V
OUT
= 2.5V for
REG2, and I OUT = 800mA, then R LOAD = 3.125Ω. For
REG2, R CS = 0.75V/A and gm EA = 87µS.
Choose the crossover frequency, f C ≤ f OSC / 10.
Choose 100kHz. Then calculate the value of the com-
pensation capacitor, C
C
:
C C = (V FB / V OUT ) x (R LOAD / R CS ) x (gm / (2π x f C ))
= (1.25 / 2.5) x (3.125 / 0.75) x (87 x 10 -6 / (6.28
x 100,000)) = 289pF
Choose 330pF, the next highest standard value.
Now select the compensation resistor, R C , so transient-
droop requirements are met. As an example, if 3% tran-
sient droop is allowed for the desired load step, the
input to the error amplifier moves 0.03 x 1.25V, or
37.5mV. The error-amplifier output drives 37.5mV x
gm
EA , or I EAO = 37.5mV x 87µS = 3.26µA across R C to
provide transient gain. Find the value of R
C
that allows
the required load-step swing from:
R
C
= R
CS
x I
IND(PK)
/ I
EAO
where I
IND(PK)
is the peak inductor current. In a step-
down DC-DC converter, if L IDEAL is used, output cur-
rent relates to inductor current by:
I IND(PK) = 1.25 x I OUT
So for an 800mA output load step with V IN = 3.6V and
V OUT = 2.5V:
R C = R CS x I IND(PK) / I EAO = (0.75V/A) x
(1.25 x 0.8A) / 3.26µA = 230kΩ
We choose 240kΩ. Note that the inductor does not limit
the response in this case since it can ramp at (V
IN -
V OUT ) / L, or (3.6 - 2.5) / 3.3µH = 242mA/µs.
The output filter capacitor is then selected so that the
C
OUT R LOAD pole cancels the R C C C zero:
C OUT x R LOAD = R C x C C
For the example:
R LOAD = V OUT x I LOAD = 2.5V / 0.8A =
3.125Ω
C OUT = R C x C C / R LOAD = 240kΩ x 330pF /
3.125Ω = 25µF
We choose 22µF.
Recalculate R C using the selected C OUT .
R C = C OUT x R LOAD / C C = 208kΩ
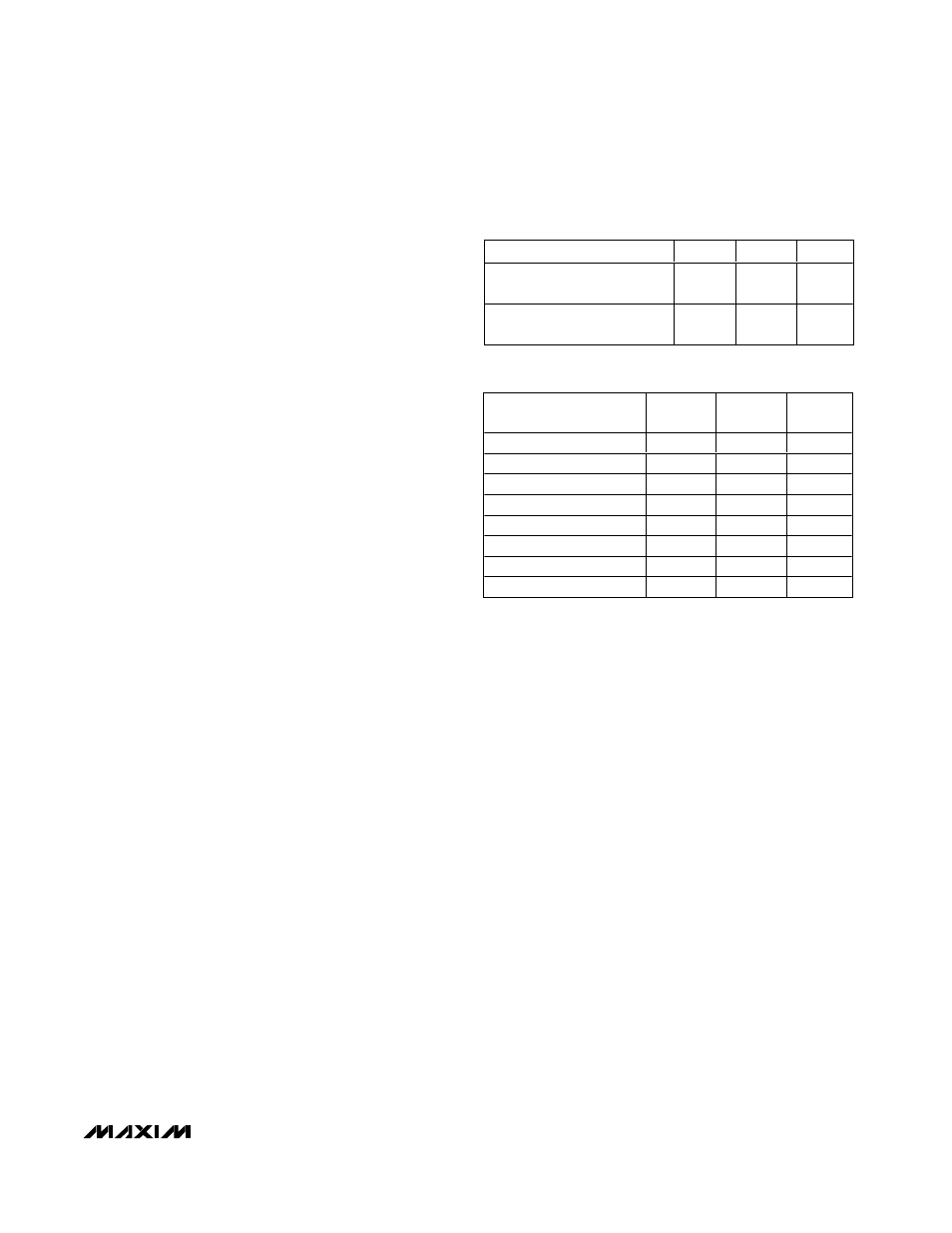
PARAMETER REG1 REG2 REG3
Error-Amplifier
Transconductance, gmEA
87µS 87µS 68µS
Current-Sense Amp
Transresistance, R CS
0.5V/A 0.75V/A 1.25V/A
Table 4. Compensation Parameters
COMPONENT OR
PARAMETER
REG1 REG2 REG3
V
OUT
3.3V 2.5V 1.3V
Output Current 1300mA 900mA 500mA
Inductor 3.3µH 6.8µH 10µH
Load-Step Droop 3% 3% 3%
Loop Crossover Freq (fC) 100kHz 100kHz 100kHz
C C 330pF 270pF 330pF
R C 240k
Ω
240k
Ω
240k
Ω
C OUT 22µF 22µF 22µF
Table 5. Typical Compensation Values