V• e – Casio fx-50F PLUS User Manual
Page 60
E-59
No. 05
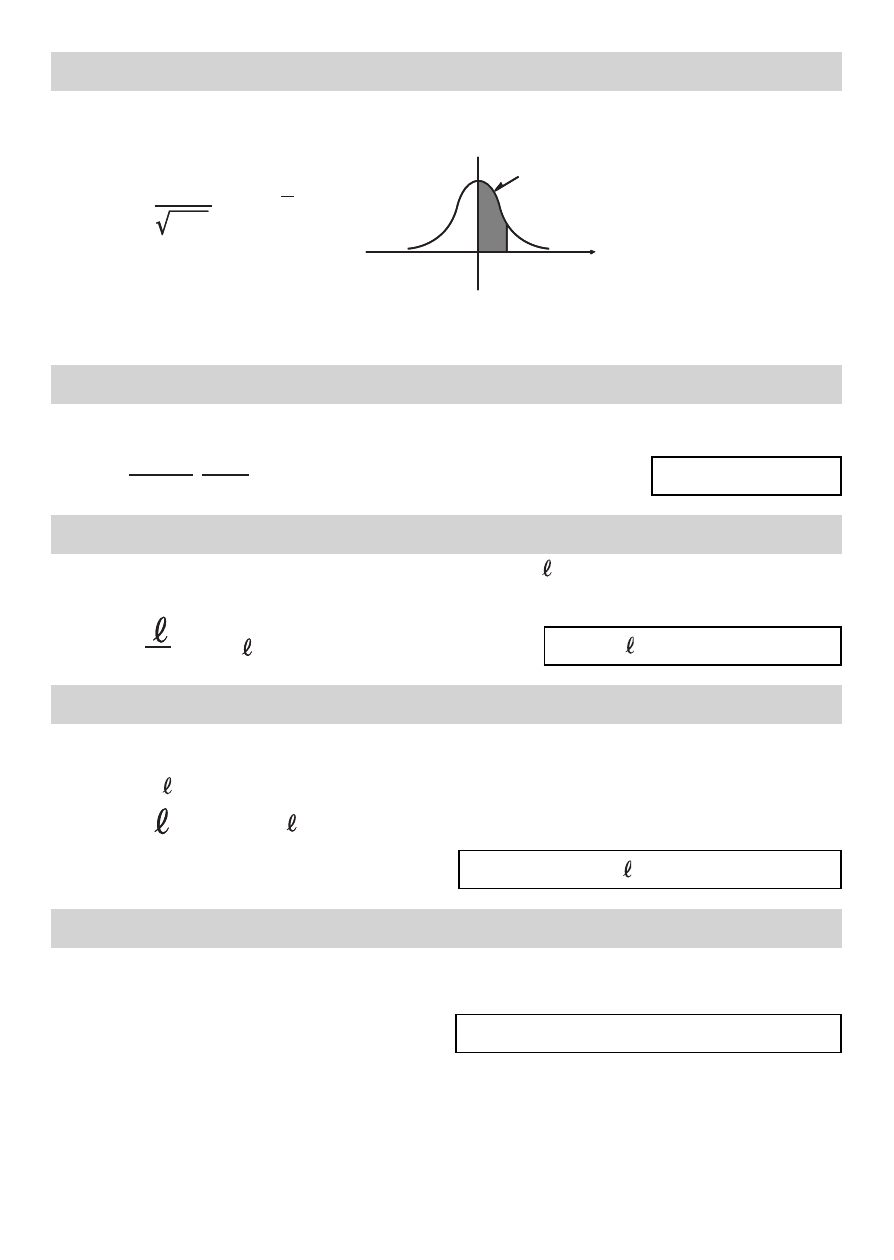
Normal Probability Function Q(
x
)
Uses Hastings’ estimate formula to determine the probability of a standard normal
distribution Q(
x
) illustrated below when the standardized variate (
x
) is known.
Important!
Since this is an estimate formula, proper precision may not be obtainable.
No. 06
Coulomb’s Law
Determines the force (
F
) between two charges of quantities
Q
and
q
, over a separation of
r
.
(
Ƥ
0
: permittivity)
Q
(
x
)
=
e
dt
(0
x
<
1
×
10
50
)
2
π
1
|
x
|
∫
2
2
t
−
0
Q x
x
Q
(
x
)
=
e
dt
(0
x
<
1
×
10
50
)
2
π
1
|
x
|
∫
2
2
t
−
0
Q x
x
F
=
Q
q
(
r
>
0)
4
πε
0
1
r
2
F
=
Q
q
(
r
>
0)
4
πε
0
1
r
2
Units:
Q
,
q
: C,
r
: m
No. 07
Resistance of a Conductor
Determines resistance
R
of a conductor when its length ( ) and cross sectional area (
S
),
and the resistance of its component material (
ρ ) are known.
R
=
ρ
(S, ,
ρ
>
0)
S
R
=
ρ
(S, ,
ρ
>
0)
S
Units: : m,
S
: m
2
,
ρ: Ω·m,
R
:
Ω
No. 08
Magnetic Force
Determines the motive force (
F
) in a conductor with electric current (
I
) fl owing through it
and placed in a magnetic fi eld of uniform magnetic force density (
B
), when the length of the
conductor is and the angle formed by the conductor and magnetic fi eld is
Ƨ
.
Units:
B
: T,
I
: A, : m,
Ƨ
: ° (degrees),
F
: N
No. 09
Change in Terminal Voltage of R in an RC Series Circuit
Determines the terminal voltage (
V
R
) of terminal
R
at time
t
in an RC series circuit when
voltage
V
is applied to a circuit with a resistance of
R
and capacitance of
C
.
F
=
IB
(
>
0, 0˚
| | 90˚)
sin
θ
θ
F
=
IB
(
>
0, 0˚
| | 90˚)
sin
θ
θ
V
R
=
V•e
−
t
/
CR
(
C
,
R
,
t
>
0)
V
R
=
V•e
−
t
/
CR
(
C
,
R
,
t
>
0)
Units:
R
:
Ω,
C
: F,
t
: seconds,
V
and
V
R
: V
