National Instruments FP-RTD-122 - Manual (Page 8)

FP-RTD-122 and cFP-RTD-122 8 ni.com
The [c]FP-RTD-122 uses a linearization curve known as the
Callendar-Van Dusen equation to measure the temperature of
RTDs. The equation is as follows:
Temperatures below 0 °C:
R
T
= R
0
[1 + A
× T + B × T 2 + C
× T 3 × (T – 100 °C)]
Temperatures above 0
°C:
R
T
= R
0
[1 + A × T + B × T 2 ]
T = temperature in
°C
R
T
= RTD resistance at temperature T
R
0
= RTD nominal resistance at 0 °C
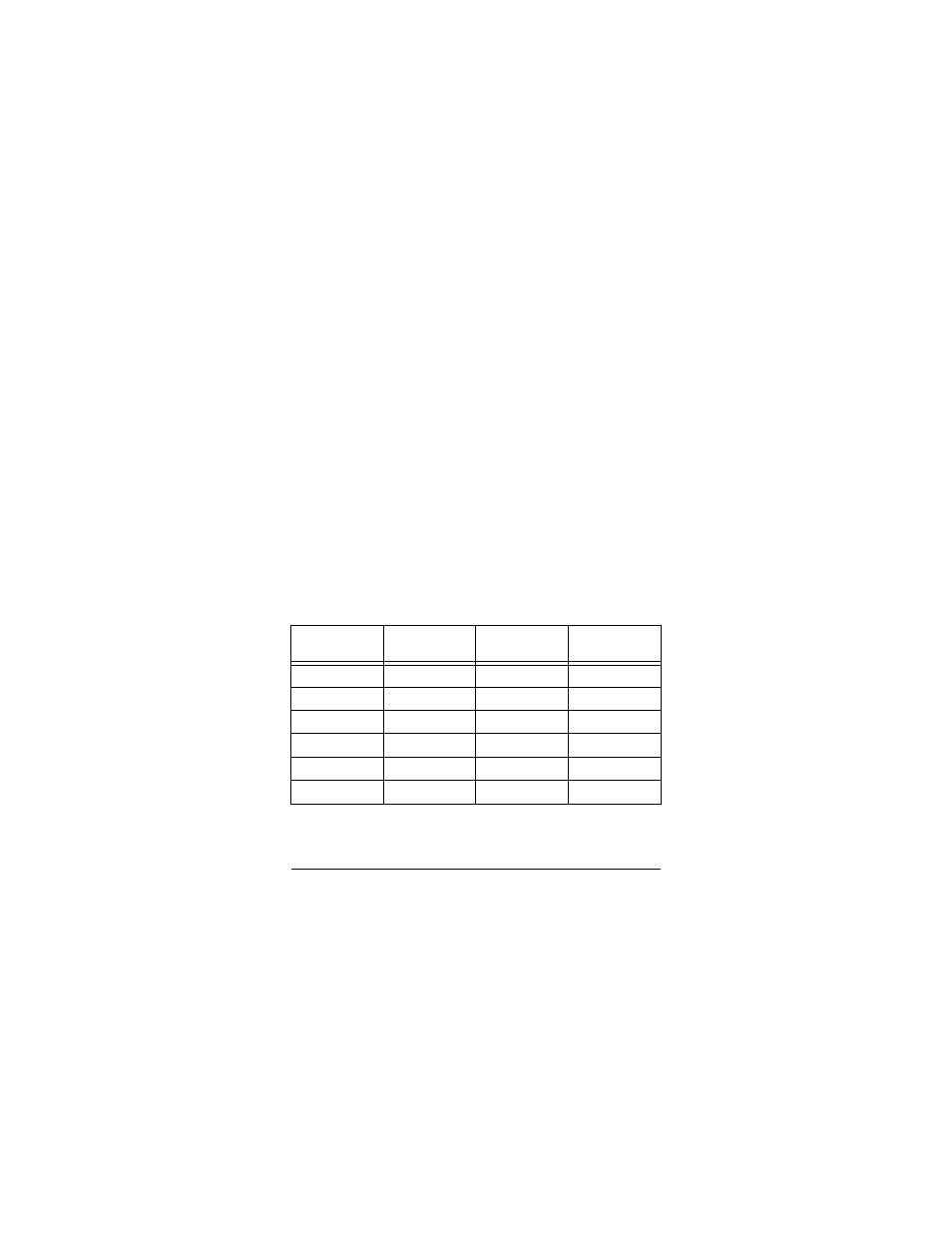
A, B, C are coefficients given in Table 2.
Table 2 lists the coefficients used in this equation for each of the
TCR values that the [c]FP-RTD-122 supports. If you have a
nonstandard RTD that does not match one of these linearization
curves, measure the resistance with the [c]FP-RTD-122 and
convert the resistance to temperature in the manner suggested by
the RTD vendor.
Three-Wire Compensation of Lead
Resistance Errors
The [c]FP-RTD-122 uses a three-wire compensation technique to
compensate for the lead resistances. The SENSE lead measures the
resistance of the return COM lead. If the EX+ lead has the same
resistance as the COM lead, the [c]FP-RTD-122 corrects for the
Table 2. Callendar-Van Dusen Coefficients Used by the [c]FP-RTD-122
TCR
m Ω/Ω/°C
A
( °C) –1
B
( °C) –2
C
( °C) –4
3.750 a 3.81
Ч
10 –3 –6.02
Ч
10 –7 –6.0
Ч
10 –12
3.851 b 3.9083
Ч
10 –3 –5.775
Ч
10 –7 –4.183
Ч
10 –12
3.911 c 3.9692
Ч
10 –3 –5.8495
Ч
10 –7 –4.233
Ч
10 –12
3.916 d 3.9739
Ч
10 –3 –5.870
Ч
10 –7 –4.4
Ч
10 –12
3.920 e 3.9787
Ч
10 –3 –5.8686
Ч
10 –7 –4.167
Ч
10 –12
3.928 f 3.9888
Ч
10 –3 –5.915
Ч
10 –7 –3.85
Ч
10 –12