Applications information, Table 2. suggested values (v, 5v, i – Rainbow Electronics MAX1623 User Manual
Page 10: 3a, f = 300khz)
MAX1623
3A, Low-Voltage, Step-Down Regulator with
Synchronous Rectification and Internal Switches
10 _______________________________________________________________________________________
flow. A copper area of 0.4in
2
showed thermal resis-
tance of 60°C/W.
Airflow over the IC can significantly reduce
θ
JA
.
Power Dissipation
The MAX1623’s power dissipation consists mostly of con-
duction losses in the two internal power switches. Power
dissipation due to supply current in the control section
and average current used to charge and discharge the
gate capacitance of the two power switches is less than
30mW at 300kHz. This number is reduced when switch-
ing frequency is reduced as the part enters Idle Mode.
Combined conduction loss in the two power switches is
calculated by:
P
D
= I
LOAD2
(R
ON
)
where R
ON
= 100m
Ω (max).
The
θ
JA
required to deliver this amount of power is cal-
culated by:
θ
JA
= (T
J(MAX)
– T
A(MAX)
) / P
D
where:
T
J(MAX)
= maximum allowed junction temperature
T
A(MAX)
= maximum ambient temperature expected
Applications Information
Inductor L1
The inductor value can be adjusted to optimize the
design for size, cost, and efficiency. Three key inductor
parameters must be specified: inductance value (L),
peak current (I
PEAK
), and DC resistance (R
DC
). The fol-
lowing equation includes a constant, denoted as LIR,
which is the ratio of inductor peak-to-peak AC current
to DC load current. A higher value of LIR allows smaller
inductance, but results in higher losses and ripple. A
good compromise between size and losses is found at
a 30% ripple current to load current ratio (LIR = 0.3),
which corresponds to a peak inductor current 1.15
times the DC load current:
where:
f = switching frequency
I
OUT
= maximum DC load current
LIR = ratio of AC to DC inductor current, typically
0.3
The peak inductor current at full load is 1.15 x I
OUT
if
the above equation is used; otherwise, the peak current
can be calculated by:
The inductor’s DC resistance is a key parameter for effi-
ciency and must be minimized, preferably to less than
25m
Ω at I
OUT
= 3A. To reduce EMI, use a shielded
inductor.
Input and Output Filter
Capacitors (C1, C2)
Use a low-ESR input capacitor according to the input
ripple-current requirements and voltage rating.
In addition to C1, place a 10µF ceramic bypass capacitor
from the power input (pin 2, 4, 6) to power ground (pin
15, 17, 19) within 5mm of the IC.
The output filter capacitor determines the output volt-
age ripple and output load-transient response, as well
as the loop’s stability.
The output ripple in continuous-conduction mode is:
where f is the switching frequency.
V
I
LIR ESR
1
2
f
C2
OUT(RPL)
OUT(MAX)
C2
=
Ч
+
Ч Ч
π
I
I
V
V
V
V
RIPPLE
LOAD
OUT
IN
OUT
IN
=
−
(
)
I
I
V
(V
V
)
2
f
L
V
PEAK
OUT
OUT
IN(MAX)
OUT
IN(MAX)
=
+
−
Ч Ч
Ч
L
V
(V
V
)
V
f
(I
) (LIR)
OUT
IN(MAX)
OUT
IN(MAX)
OUT
=
−
Ч Ч
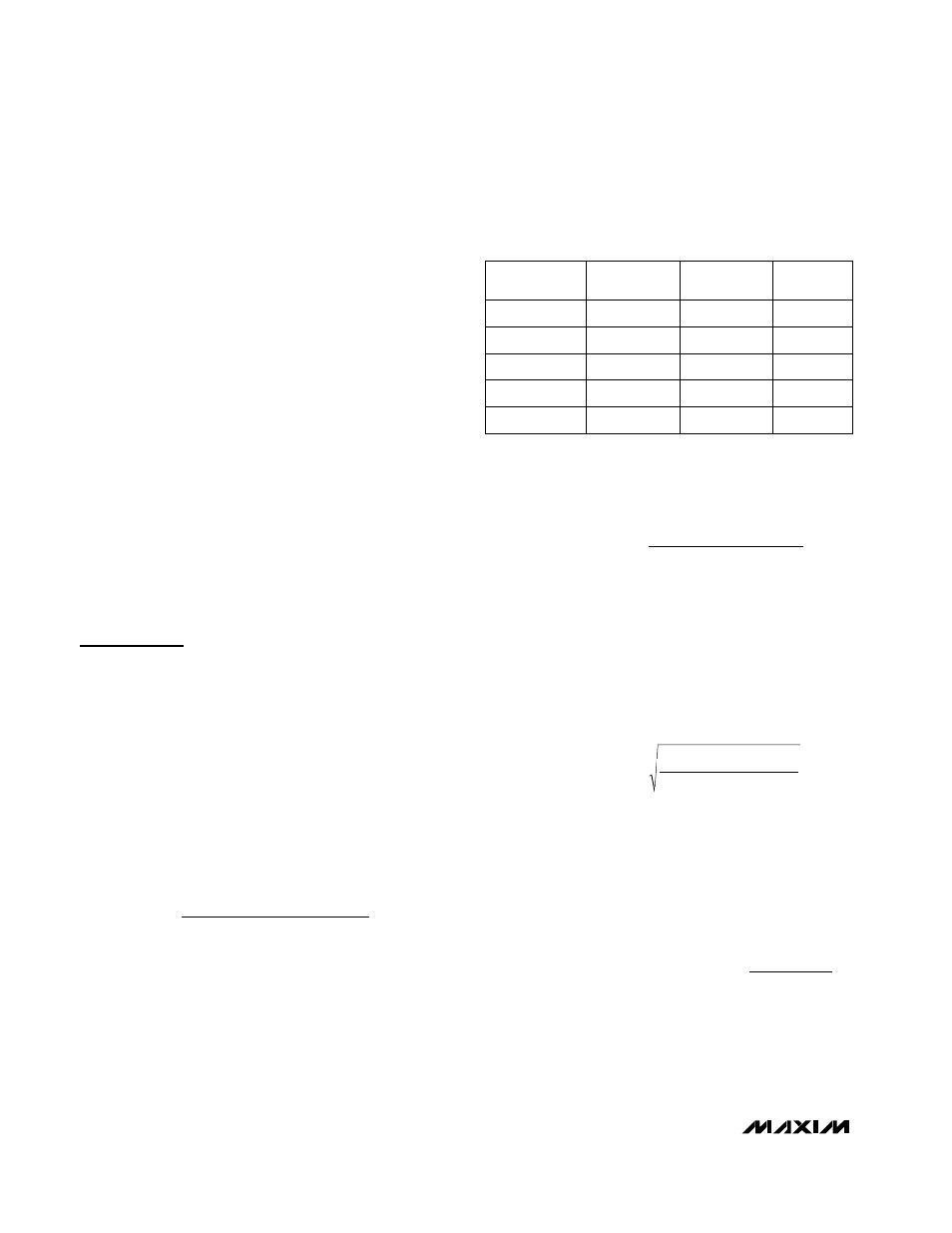
Table 2. Suggested Values (V
IN
= 5V,
I
O
= 3A, f = 300kHz)
T
OFF
(µs)
L
(µH)
3.3
1.10
4.7
2.5
1.67
4.7
V
OUT
(V)
1.8
2.16
4.7
1.5
2.38
3.9
1.1
2.68
3.3
R
TOFF
(k
Ω
)
120
180
240
260
280
