Rainbow Electronics LM20 - Manual (Page 5)

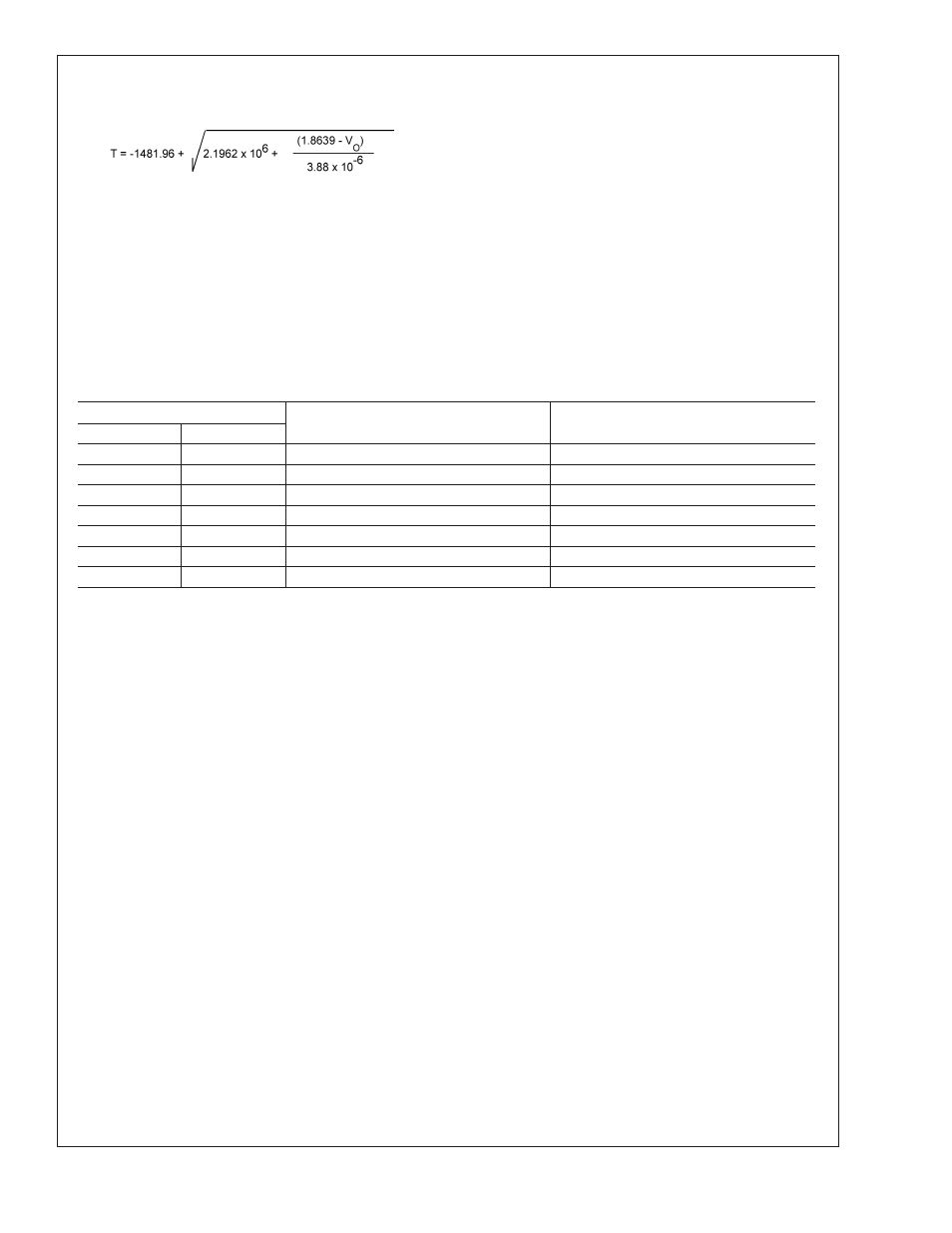
1.0 LM20 Transfer Function
(Continued)
A linear transfer function can be used over a limited tempera-
ture range by calculating a slope and offset that give best
results over that range. A linear transfer function can be
calculated from the parabolic transfer function of the LM20.
The slope of the linear transfer function can be calculated
using the following equation:
m = −7.76 x 10 −6 x T − 0.0115,
where T is the middle of the temperature range of interest
and m is in V/˚C. For example for the temperature range of
T
min
=−30 to T
max
=+100˚C:
T=35˚C
and
m = −11.77 mV/˚C
The offset of the linear transfer function can be calculated
using the following equation:
b = (V
OP
(T
max
) + V
OP
(T) + m x (T
max
+T))/2
,
where:
• V
OP
(T
max
) is the calculated output voltage at T
max
using
the parabolic transfer function for V
O
• V
OP
(T) is the calculated output voltage at T using the
parabolic transfer function for V
O
.
Using this procedure the best fit linear transfer function for
many popular temperature ranges was calculated in Figure
2. As shown in Figure 2 the error that is introduced by the
linear transfer function increases with wider temperature
ranges.
2.0 Mounting
The LM20 can be applied easily in the same way as other
integrated-circuit temperature sensors. It can be glued or
cemented to a surface. The temperature that the LM20 is
sensing will be within about +0.02˚C of the surface tempera-
ture to which the LM20’s leads are attached to.
This presumes that the ambient air temperature is almost the
same as the surface temperature; if the air temperature were
much higher or lower than the surface temperature, the
actual temperature measured would be at an intermediate
temperature between the surface temperature and the air
temperature.
To ensure good thermal conductivity the backside of the
LM20 die is directly attached to the pin 2 GND pin. The
tempertures of the lands and traces to the other leads of the
LM20 will also affect the temperature that is being sensed.
Alternatively, the LM20 can be mounted inside a sealed-end
metal tube, and can then be dipped into a bath or screwed
into a threaded hole in a tank. As with any IC, the LM20 and
accompanying wiring and circuits must be kept insulated and
dry, to avoid leakage and corrosion. This is especially true if
the circuit may operate at cold temperatures where conden-
sation can occur. Printed-circuit coatings and varnishes such
as Humiseal and epoxy paints or dips are often used to
ensure that moisture cannot corrode the LM20 or its connec-
tions.
The thermal resistance junction to ambient ( θ
JA
) is the pa-
rameter used to calculate the rise of a device junction tem-
perature due to its power dissipation. For the LM20 the
equation used to calculate the rise in the die temperature is
as follows:
T
J
= T
A
+ θ
JA
[(V + I
Q
) + (V + − V
O
) I
L
]
where I
Q
is the quiescent current and I
L
is the load current on
the output. Since the LM20’s junction temperature is the
actual temperature being measured care should be taken to
minimize the load current that the LM20 is required to drive.
The tables shown in Figure 3 summarize the rise in die
temperature of the LM20 without any loading, and the ther-
mal resistance for different conditions.
Temperature Range Linear Equation
V
O
=
Maximum Deviation of Linear Equation
from Parabolic Equation (˚C) T
min
(˚C) T
max
(˚C)
−55 +130 −11.79 mV/˚C x T + 1.8528 V ± 1.41
−40 +110 −11.77 mV/˚C x T + 1.8577 V ± 0.93
−30 +100 −11.77 mV/˚C x T + 1.8605 V ± 0.70
-40 +85 −11.67 mV/˚C x T + 1.8583 V ± 0.65
−10 +65 −11.71 mV/˚C x T + 1.8641 V ± 0.23
+35 +45 −11.81 mV/˚C x T + 1.8701 V ± 0.004
+20 +30 −11.69 mV/˚C x T + 1.8663 V ± 0.004
FIGURE 2. First order equations optimized for different temperature ranges.
LM20
www.national.com 5