Averaged power spectrum, Averaged cross spectrum – National Instruments NI Spectral Measurements Toolkit User Manual
Page 28
NI Spectral Measurements Toolkit User Guide
28
ni.com
Averaged Power Spectrum
The following equations describe the averaging methods you can apply
to a complex FFT spectrum to yield an averaged power spectrum. The
No averaging method converts the complex FFT spectrum to a real power
spectrum.
The averaged power spectrum is equivalent to the square of the magnitude
of the averaged FFT spectrum.
Averaged Cross Spectrum
If you have two FFT spectra, X and Y, the cross spectrum S
xy
results
from multiplying the complex conjugate of spectrum X by spectrum Y as
follows:
S
xy
= conj(X)
× Y
For RMS averaging, an averaged cross spectrum consists of the average of
the individual cross spectra as follows:
S
xy
= < conj(X)
× Y >
For vector averaging, an averaged cross spectrum consists of the vector
average of each spectrum computed before multiplying the two averaged
spectra as follows:
S
xy
= conj(<X>)
× <Y>
A cross spectrum has no peak-hold average.
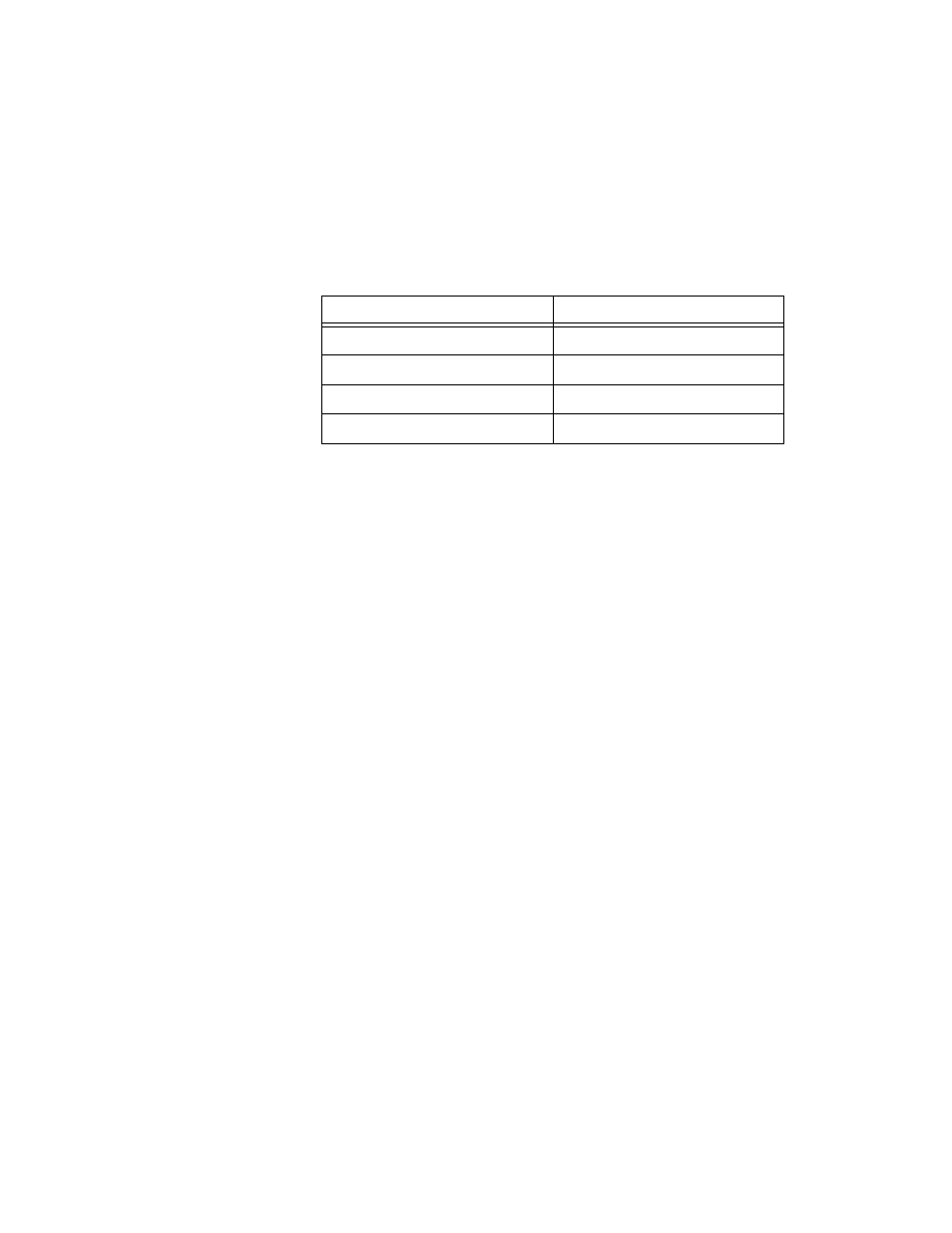
Table 3. Averaged Power Spectrum Averaging Methods and Equations
Averaging Method
Equation
No averaging
Y = X conj(X)
Vector averaging
Y
k
= <X>
k
conj(<X>
k
)
RMS averaging
Y
k
= <X conj(X)>
k
Peak-hold
Y
k
= max(X
k
, Y
k-1
)
2
